
Bayesian Approach We can truncate the estimator as A0元<-A0 A={元 -A0≤元≤A0 元>A0 Q:which one is better? whxiong@uestc.edu.cn 6
whxiong@uestc.edu.cn Bayesian Approach 6 We can truncate the estimator as A¸ = 8 >< >: ¡A0 x¹< ¡A0 x¹ ¡A0 6 x¹6 A0 A0 x¹> A0 Q: which one is better?
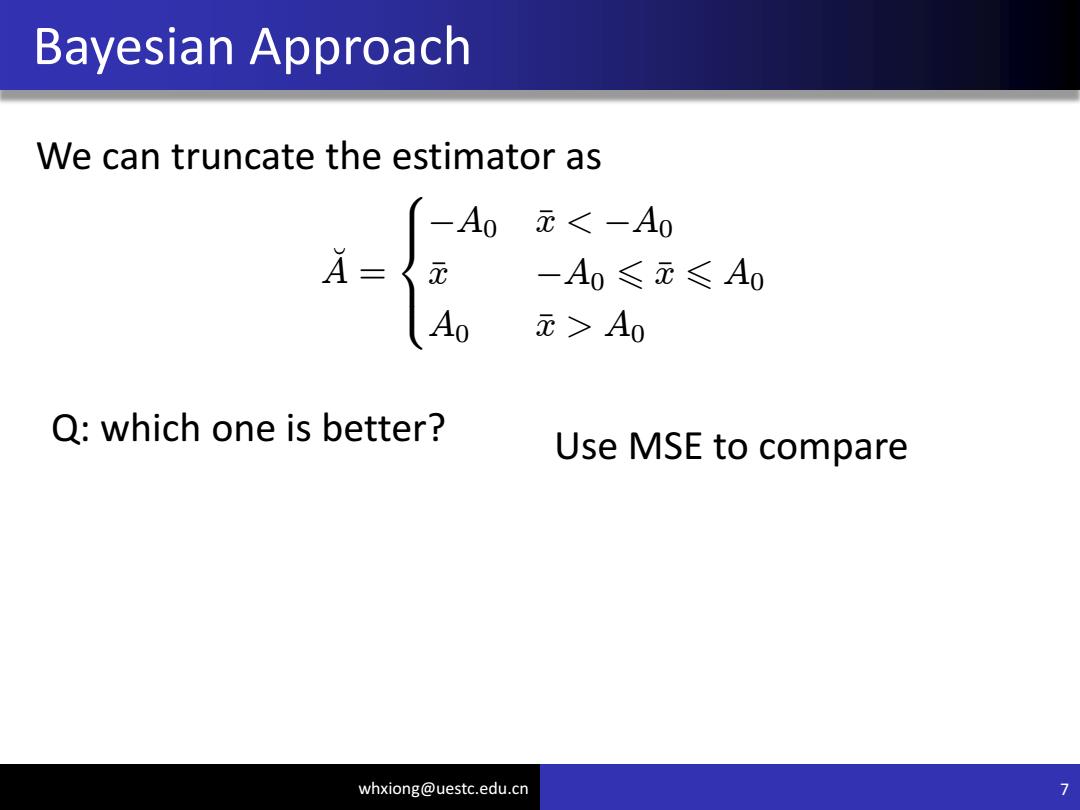
Bayesian Approach We can truncate the estimator as A0元<-A0 A={元 -A0≤元≤A0 A 元>A0 Q:which one is better? Use MSE to compare whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Bayesian Approach 7 We can truncate the estimator as A¸ = 8 >< >: ¡A0 x¹< ¡A0 x¹ ¡A0 6 x¹6 A0 A0 x¹> A0 Q: which one is better? Use MSE to compare

Bayesian Approach We can truncate the estimator as -A0元<-A0 A= 〈元 -A0≤元≤A0 (A0 元>A0 Q:which one is better? Use MSE to compare MsE④=(④-A2Paa,AdA MSE(A)=(A-A)2PA(A,A)dA whxiong@uestc.edu.cn 8
whxiong@uestc.edu.cn Bayesian Approach 8 We can truncate the estimator as MSE(A b ) = Z +1 ¡1 (A b ¡ A) 2 PA b (A b ; A)dA b A¸ = 8 >< >: ¡A0 x¹< ¡A0 x¹ ¡A0 6 x¹6 A0 A0 x¹> A0 Q: which one is better? MSE(A ¸ ) = Z +1 ¡1 (A ¸¡ A) 2 PA¸(A ¸ ; A)dA ¸ Use MSE to compare

Bayesian Approach 。PDFS i=27a{xA- whxiong@uestc.edu.cn 9
whxiong@uestc.edu.cn PDFs Bayesian Approach 9 p(A b ) = 1 (2¼¾2 =N) 1 2 ¢exp ½ ¡ 1 2¾2 =N (A b ¡ A) ¾

Bayesian Approach 。PDFS () m{wi p(A)Prob(A<-A1)6(A+Ao) + Pa(A,A)u(+Ao)-u(A-Ao) +Prob(A>Ao)6(A-Ao)) whxiong@uestc.edu.cn 10
whxiong@uestc.edu.cn PDFs Bayesian Approach 10 p(A b ) = 1 (2¼¾2 =N) 1 2 ¢exp ½ ¡ 1 2¾2 =N (A b ¡ A) ¾ p(A¸) = Prob(A· < ¡A1)±(A· + A0) + PAb (Ab; A) h u(A¸ + A0) ¡ u(A¸¡ A0) i + Prob(A¸ > A0)±(A¸¡ A0)