
Estimation Theory Maximum Likelihood Estimator (MLE) Wenhui Xiong NCL UESTC whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Estimation Theory Maximum Likelihood Estimator (MLE) Wenhui Xiong NCL UESTC

Review MVU Estimator 。CRLB→factorize a1np(x9=I(8)(g(x)-0) 80 RBLS: Use sufficient statistics:T(x)ET] unbiased estimator over sufficient statistics:g[T(x)] BLUE:if the unknown is a linear function of the data 6=(HC-1Ⅲ)-1HPC-1x whxiong@uestc.edu.cn 2
whxiong@uestc.edu.cn Review MVU Estimator 2 RBLS: Use sufficient statistics: T(x) unbiased estimator over sufficient statistics: g[T(x)] E[µ · jT(x)] BLUE: if the unknown is a linear function of the data CRLB factorize µ ^ = (HT C¡1 H) ¡1 HT C¡1 x @lnp(x; µ) @µ = I(µ)(g(x) ¡ µ)

How about CRLB method? Example:Estimating DC in WGN with unknown variance A k出=g即{立∑取-4月 whxiong@uestc.edu.cn 3
whxiong@uestc.edu.cn 3 How about CRLB method? p(x; A) = 1 (2¼A) N=2 exp ½ ¡ 1 2A ³Xx[n] ¡ A ´2 ¾ Example: Estimating DC in WGN with unknown variance A

How about CRLB method? Example:Estimating DC in WGN with unknown variance A x=即{T公- olnp(x;A) ∂A -+∑-用+∑i-心 whxiong@uestc.edu.cn
whxiong@uestc.edu.cn How about CRLB method? @ ln p(x; A) @A = ¡ N 2A + 1 A X(x[n] ¡ A) + 1 2A2 X(x[n] ¡ A) 2 p(x; A) = 1 (2¼A) N=2 exp ½ ¡ 1 2A ³Xx[n] ¡ A ´2 ¾ Example: Estimating DC in WGN with unknown variance A
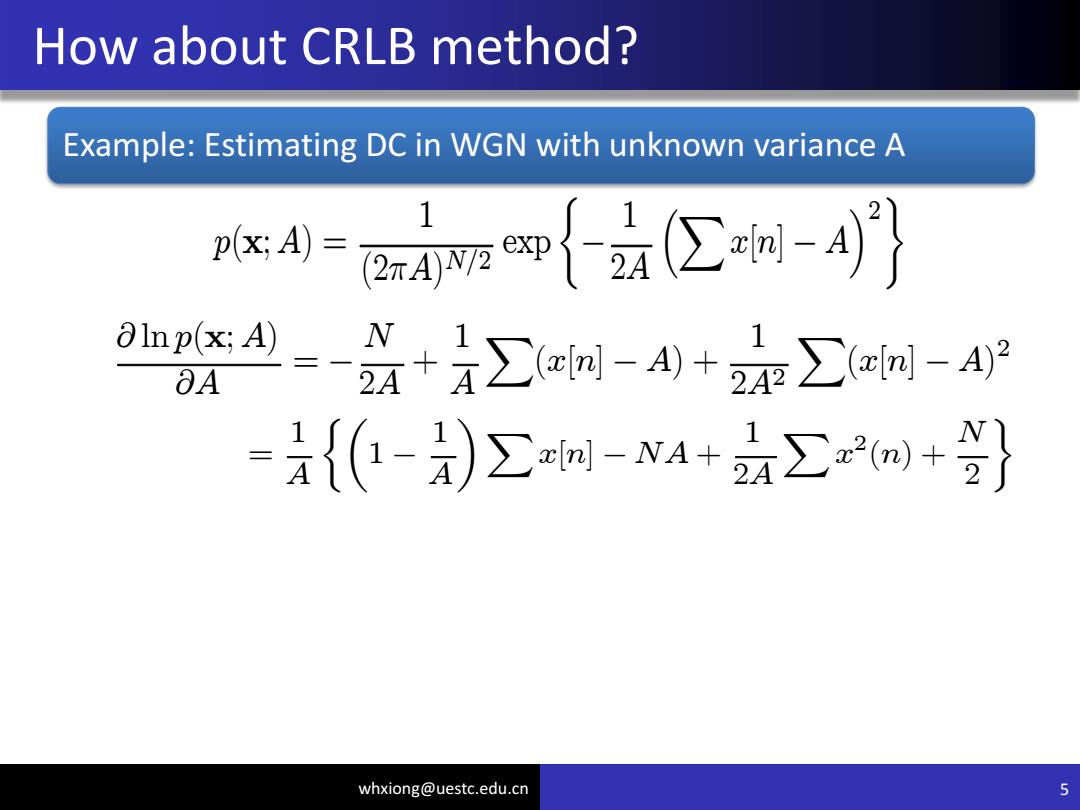
How about CRLB method? Example:Estimating DC in WGN with unknown variance A (x;A)= 卿{7公-月 0px-司 6A -4∑o网-A+京∑州-A {(1-)∑网-NA+立∑2侧+} whxiong@uestc.edu.cn 5
whxiong@uestc.edu.cn 5 = 1 A ½µ 1 ¡ 1 A ¶Xx[n] ¡ NA + 1 2A Xx 2 (n) + N 2 ¾ How about CRLB method? @ ln p(x; A) @A = ¡ N 2A + 1 A X(x[n] ¡ A) + 1 2A2 X(x[n] ¡ A) 2 p(x; A) = 1 (2¼A) N=2 exp ½ ¡ 1 2A ³Xx[n] ¡ A ´2 ¾ Example: Estimating DC in WGN with unknown variance A