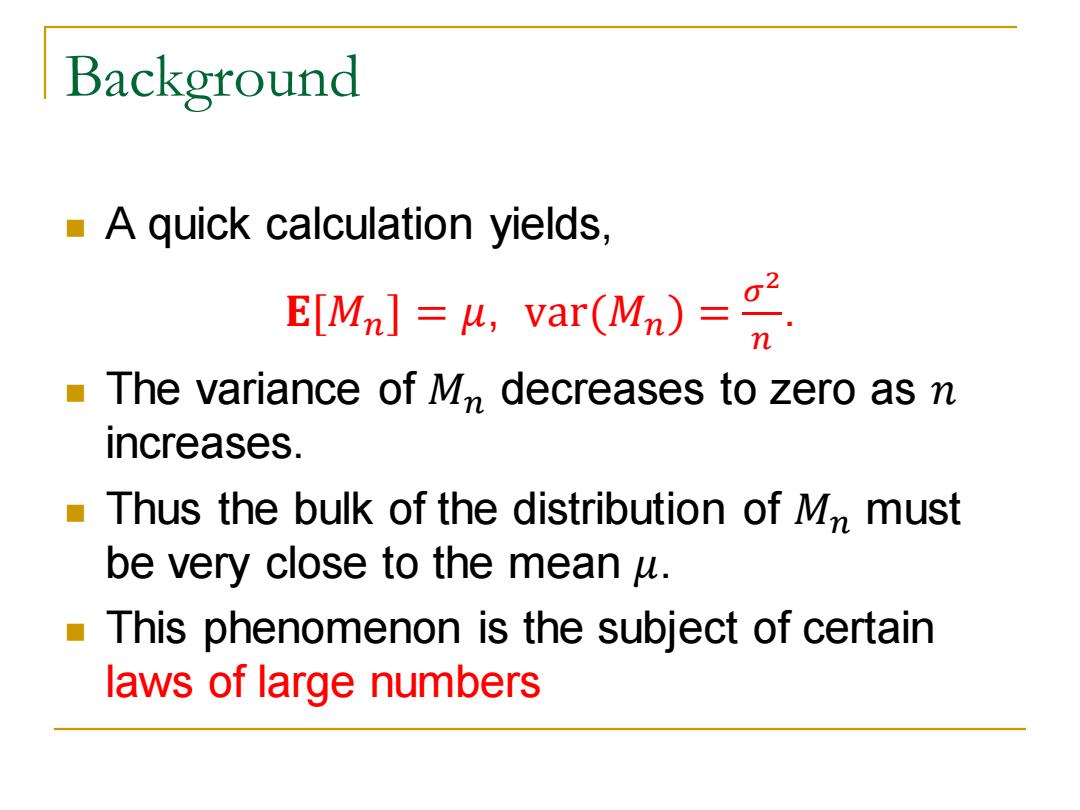
Background A quick calculation yields, E[Mn]u,var(Mn)= n The variance of M decreases to zero as n increases. Thus the bulk of the distribution of Mm must be very close to the mean u. This phenomenon is the subject of certain laws of large numbers
Background ◼ A quick calculation yields, 𝐄[𝑀𝑛] = 𝜇, var(𝑀𝑛) = 𝜎 2 𝑛 . ◼ The variance of 𝑀𝑛 decreases to zero as 𝑛 increases. ◼ Thus the bulk of the distribution of 𝑀𝑛 must be very close to the mean 𝜇. ◼ This phenomenon is the subject of certain laws of large numbers
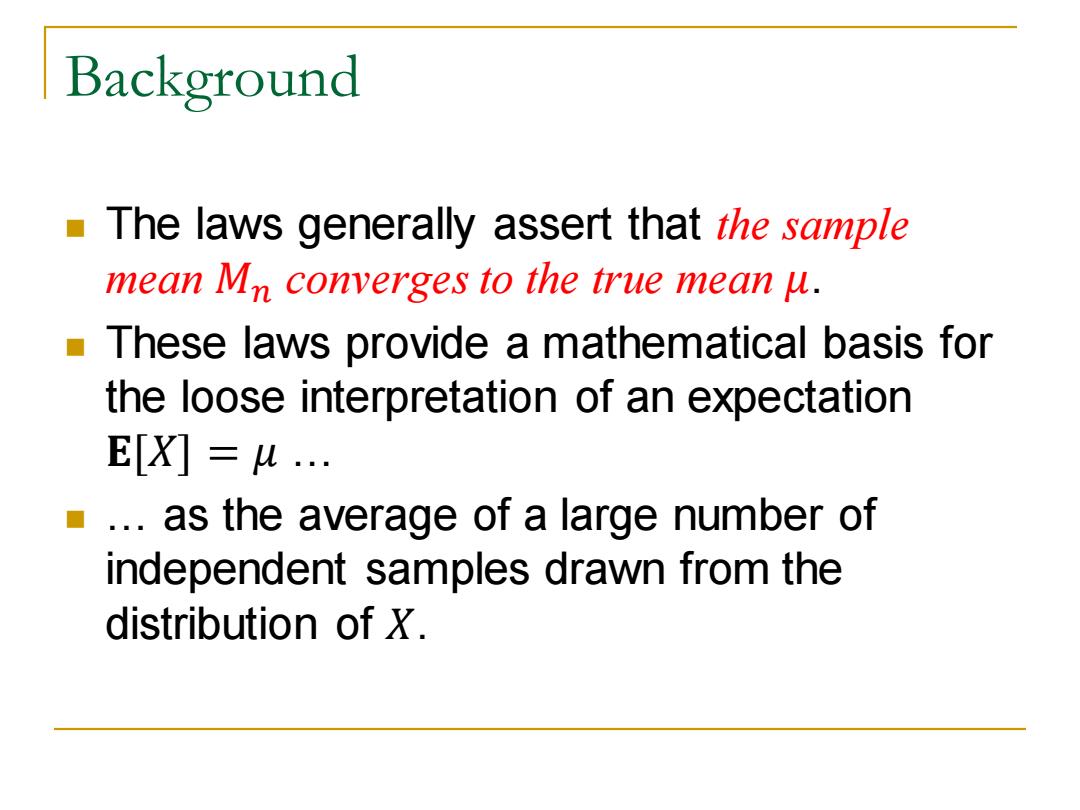
Background The laws generally assert that the sample mean Mn converges to the true mean u. These laws provide a mathematical basis for the loose interpretation of an expectation EX]=八.… .. as the average of a large number of independent samples drawn from the distribution of X
Background ◼ The laws generally assert that the sample mean 𝑀𝑛 converges to the true mean 𝜇. ◼ These laws provide a mathematical basis for the loose interpretation of an expectation 𝐄[𝑋] = 𝜇 … ◼ … as the average of a large number of independent samples drawn from the distribution of 𝑋
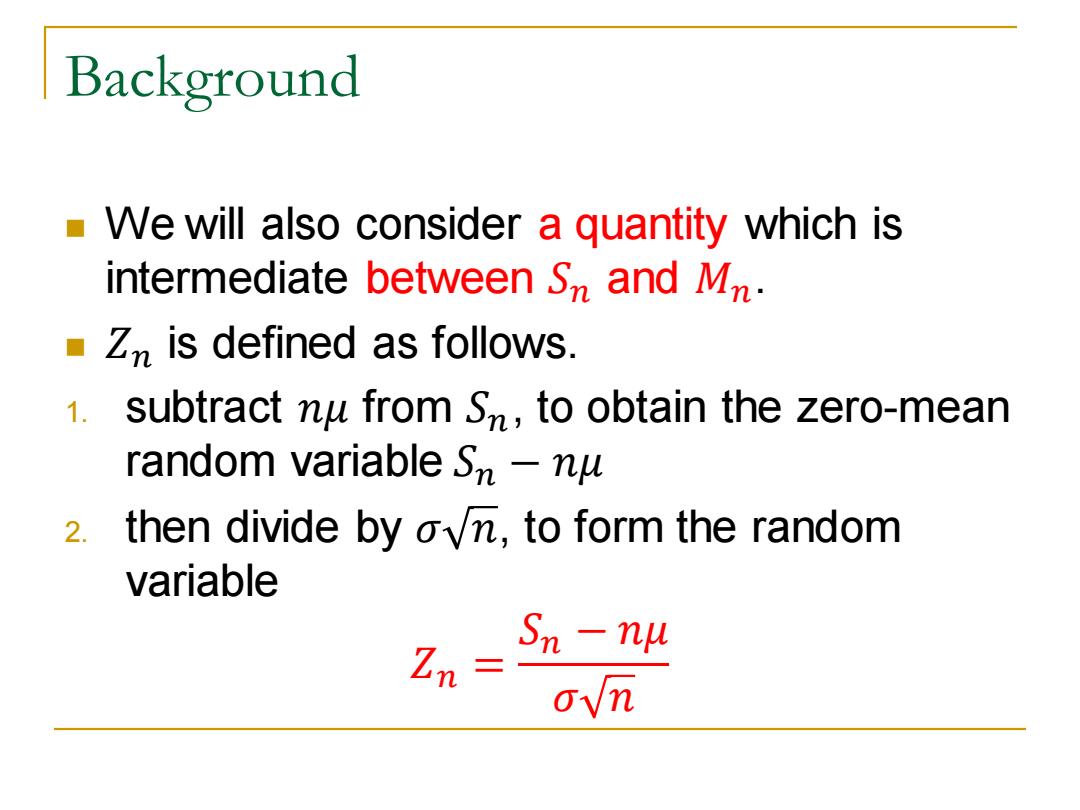
Background We will also consider a quantity which is intermediate between Sn and Mn. Zm is defined as follows. 1. subtract nu from S,to obtain the zero-mean random variable Sm-nu 2.then divide by ovn,to form the random variable In= Sn -nu σVm
Background ◼ We will also consider a quantity which is intermediate between 𝑆𝑛 and 𝑀𝑛. ◼ 𝑍𝑛 is defined as follows. 1. subtract 𝑛𝜇 from 𝑆𝑛, to obtain the zero-mean random variable 𝑆𝑛 − 𝑛𝜇 2. then divide by 𝜎 𝑛, to form the random variable 𝑍𝑛 = 𝑆𝑛 − 𝑛𝜇 𝜎 𝑛
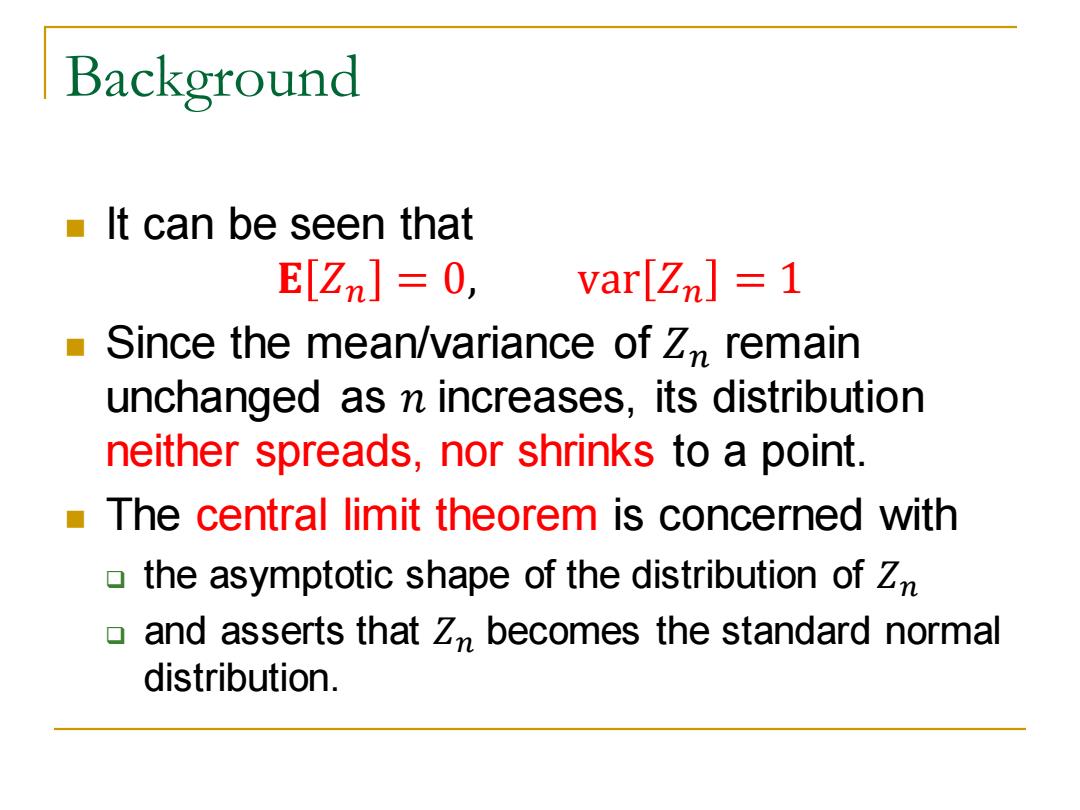
Background It can be seen that E[Zn]=0, var[Zn]1 Since the mean/variance of Zm remain unchanged as n increases,its distribution neither spreads,nor shrinks to a point. The central limit theorem is concerned with 0 the asymptotic shape of the distribution of Zn and asserts that Z,becomes the standard normal distribution
Background ◼ It can be seen that 𝐄 𝑍𝑛 = 0, var 𝑍𝑛 = 1 ◼ Since the mean/variance of 𝑍𝑛 remain unchanged as 𝑛 increases, its distribution neither spreads, nor shrinks to a point. ◼ The central limit theorem is concerned with ❑ the asymptotic shape of the distribution of 𝑍𝑛 ❑ and asserts that 𝑍𝑛 becomes the standard normal distribution
Application Limit theorems are useful for several reasons: (a)Conceptually.They provided an interpretation of expectations/probabilities in terms of a long sequence of identical independent experiments
Application ◼ Limit theorems are useful for several reasons: ◼ (a) Conceptually. They provided an interpretation of expectations/probabilities in terms of a long sequence of identical independent experiments