上海交通大学：《复杂系统动力学计算机辅助分析》课程教学资源_Chapter 6_Char6.3 Equations of motion of constrained planar

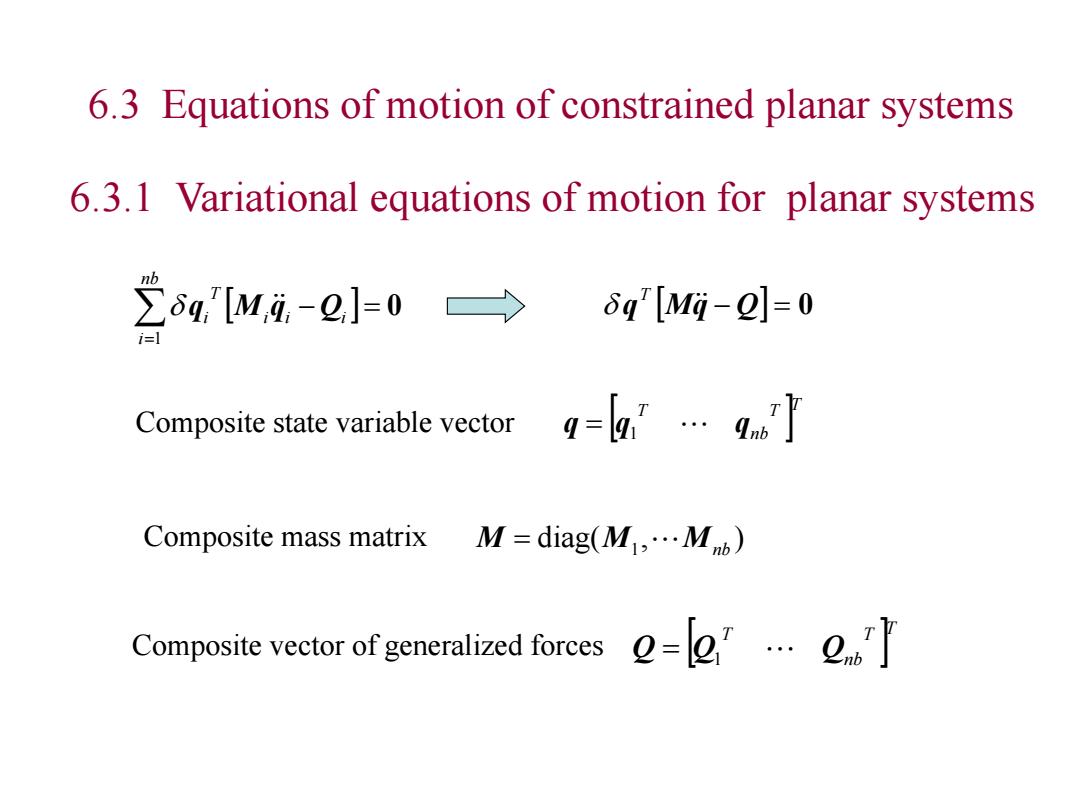
6.3 Equations of motion of constrained planar systems 6.3.1 Variational equations of motion for planar systems ∑δ[M,i-g]=0> δq[M-]=0 Composite state variable vector g=h…g Composite mass matrix M=diag(M,.M) Composite veetor of generalized forces

6.3 Equations of motion of constrained planar systems 6.3.1 Variational equations of motion for planar systems Composite state variable vector   T T nb T q  q1  q diag( , ) C M  M1 Mnb omposite mass matrix Composite vector of generalized forces   T T nb T Q  Q1  Q      0  nb i i i i T i 1  q M q Q q Mq Q  0 T 

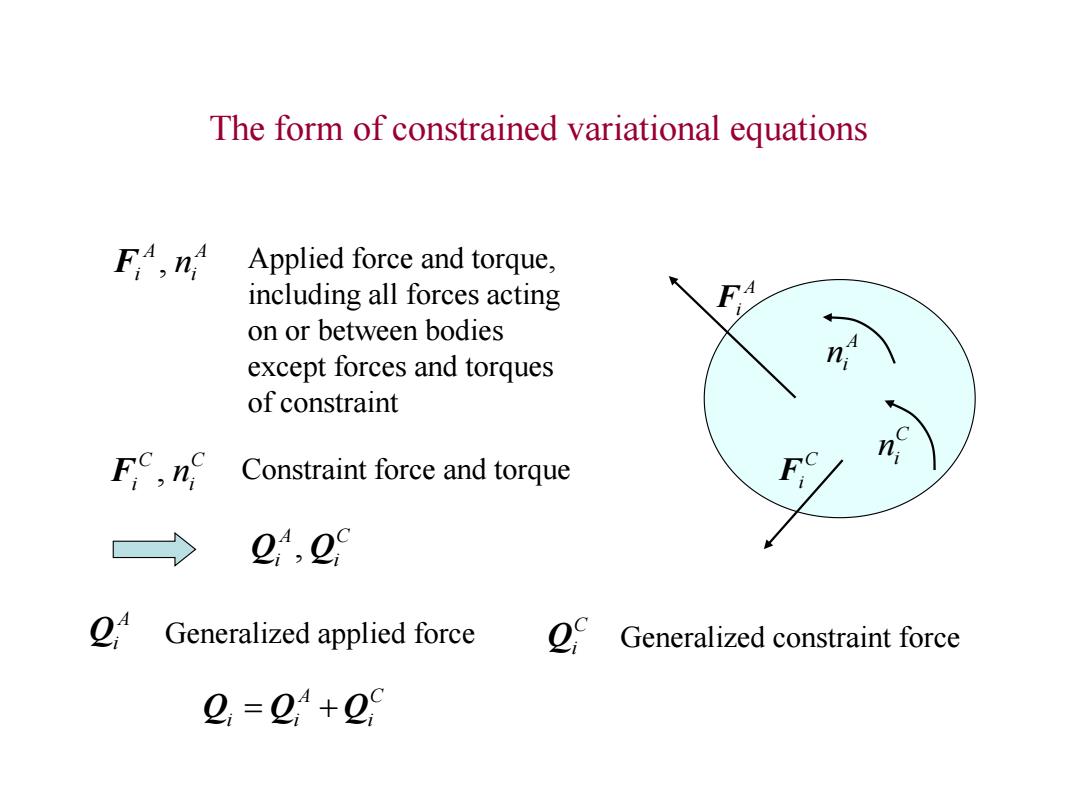
The form of constrained variational equations FA,n Applied force and torque, including all forces acting on or between bodies except forces and torques of constraint Fc.nc Constraint force and torque ，2,29 2 Generalized applied force 2 Generalized constraint force 2=2,+2

A Fi A ni C Fi C ni The form of constrained variational equations C i A Qi  Qi  Q A i A i F , n Applied force and torque, including all forces acting on or between bodies except forces and torques of constraint Constraint force and torque C i C i F , n C i A Qi , Q A Qi Generalized applied force C Qi Generalized constraint force

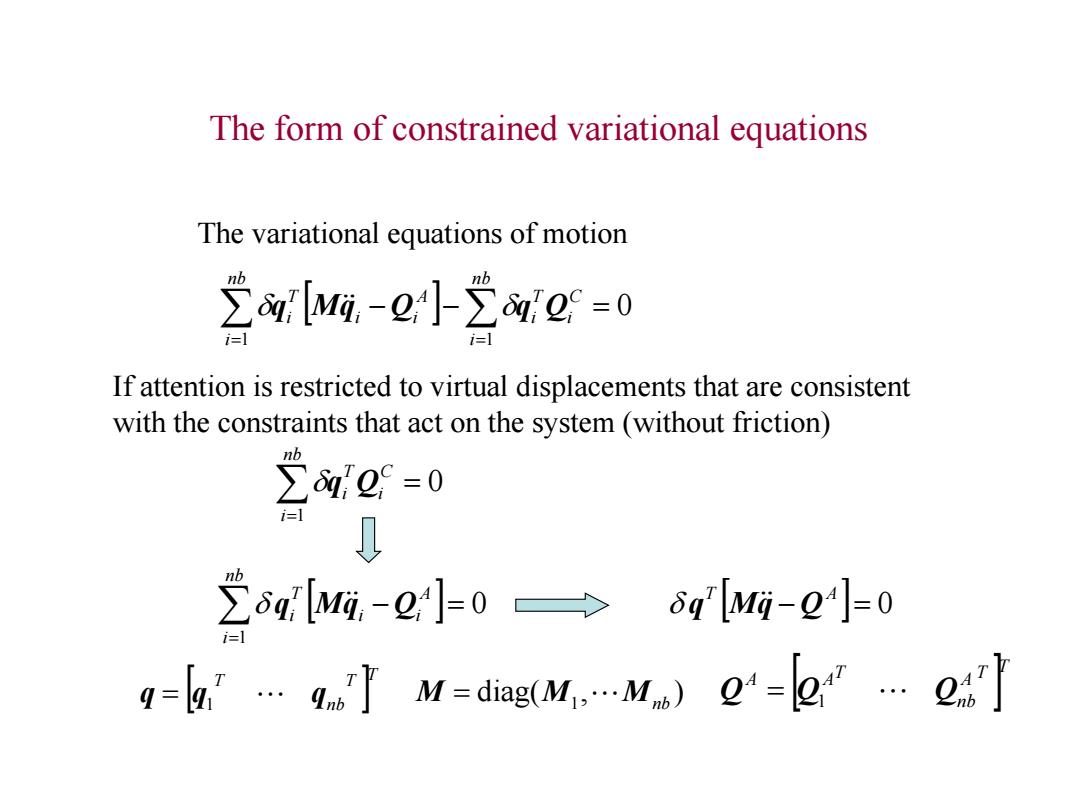
The form of constrained variational equations The variational equations of motion ∑mMi,-g]∑me=0 If attention is restricted to virtual displacements that are consistent with the constraints that act on the system (without friction) ∑42，=0 i= 0 2ogMg.-2l=0>6g-2l-0 g=a…g7M=diag(M,…M）Q=27…2

The variational equations of motion   0 1 1       nb i C i T i nb i A i i T qi Mq Q q Q If attention is restricted to virtual displacements that are consistent with the constraints that act on the system (without friction) 0 1    nb i C i T qi Q   0 1     nb i A i i T  qi Mq Q    0 T A  q Mq Q   T T nb T q  q1  q diag( , ) M  M1 Mnb   T T A nb T A A Q  Q1  Q The form of constrained variational equations

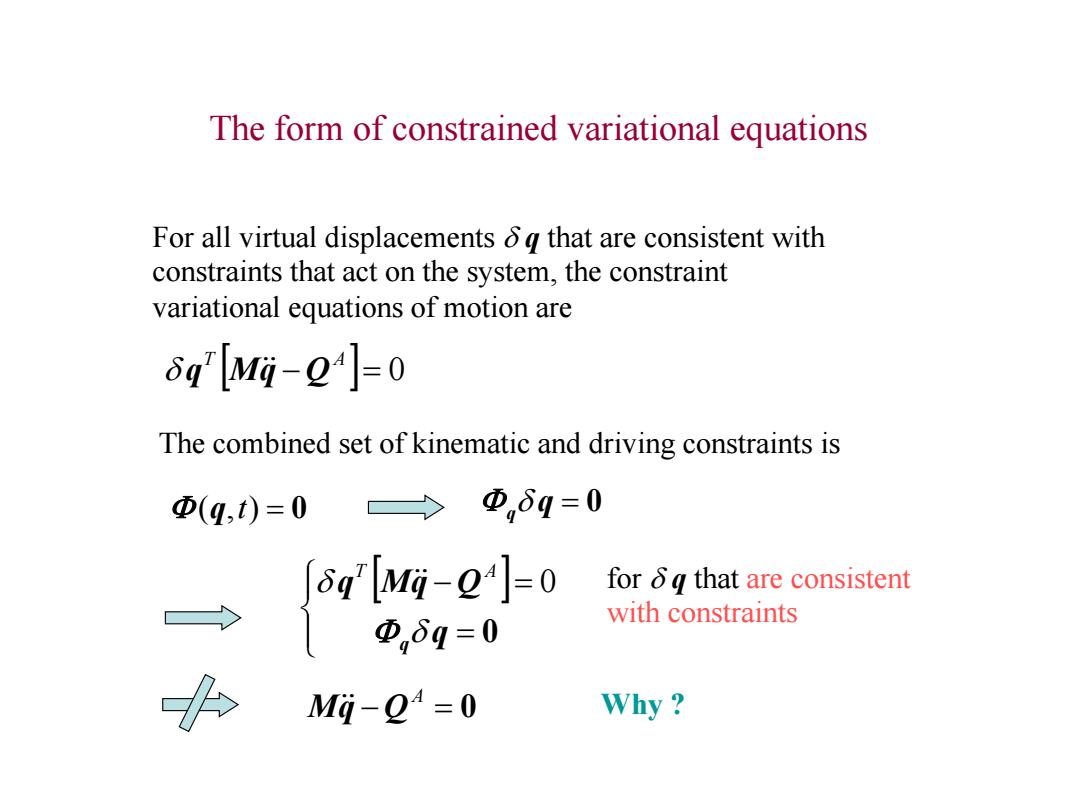
The form of constrained variational equations For all virtual displacements &g that are consistent with constraints that act on the system,the constraint variational equations of motion are δq[Mi-0]=0 The combined set of kinematic and driving constraints is Φ(q,t)=0 >中，8q=0 ∫δqMi-g]=0 for &g that are consistent Φ，δq=0 with constraints A Mi-04=0 Why

   0 T A  q Mq Q The form of constrained variational equations  (q,t)  0 q  0  q For all virtual displacements  q that are consistent with constraints that act on the system, the constraint variational equations of motion are The combined set of kinematic and driving constraints is          q 0 q Mq Q q   0 T A  for  q that are consistent with constraints   0 A Mq Q Why ?

6.3.2 Lagrange Multiplier Let b be an n vector of constants,x be an n vector of variables, and A be an mXn constant matrix If bx=0 holds for all x that satisfy x=0 There exists an m vectorA of Lagrange Multiplier such that bx+Ax=x"(b+)=0 for arbitrary x b+AT元=0 Since Mi-Q4δq=0 holds for all6 that satisfy中，δq=0 There exists an m vector of Lagrange Multiplier such that M-2δg+2'Φ，6q=6g(Mi-g+Φ，2)=0 for arbitraryδq >Mi-2+Φ2=0 Why

6.3.2 Lagrange Multiplier There exists an m vector l of Lagrange Multiplier such that Let b be an n vector of constants, x be an n vector of variables, and A be an m×n constant matrix b x  0 T If holds for all x that satisfy Ax  0  l  (  l)  0 T T T T b x Ax x b A for arbitrary x Mq Q   q  0 T A  q  0 Since holds for all  q that satisfy  q There exists an m vector l of Lagrange Multiplier such that     l      l  0 T T A T T A q Q q Mq Q  q  q  q Mq for arbitrary  q   l  0 A T Q q Mq Why ?  l  0 T b A