Uncertainties Efficiency Scoring Monte Carlo PDFs Sampling RNGs
Monte Carlo Uncertainties Efficiency PDFs RNGs Sampling Scoring

(Pseudo)Random numbers Non-correlated sequences of numbers generated by an iterative equation. Repeatability after a very long number of random numbers. Non-uniform sequence. 0.015 ·Reproducible:“seed', 10k 0.014 1M siue. 100M 0.013 Ij+1=(aI;+c)mod m 0.012 where 0.011 a =663608941 0.01 0.009 C =0 0.008 m 232 0.007 0 0.2 0.4 0.6 0.8 X
(Pseudo) Random numbers • Non-correlated sequences of numbers generated by an iterative equation. • Repeatability after a very long number of random numbers. • Non-uniform sequence. • Reproducible: “seed”. Ij+1 = (aIj + c) mod m where: a = 663608941 c = 0 m = 232

Random numbers >Uniformly distributed numbers in [0,1] Most useful method for obtaining random numbers for computer use is a pseudo random number generator >How random are these pseudo random numbers? Anyone who considers arithmetical methods of producing random digits is,of course,in a state of sin. John von Neumann(1951)
Random Numbers Uniformly distributed numbers in [0,1] Most useful method for obtaining random numbers for computer use is a pseudo random number generator How random are these pseudo random numbers? Anyone who considers arithmetical methods of producing random digits is, of course, in a state of sin. John von Neumann (1951)

Next: Using a computer to generate random events: We need to be able to generate random numbers X with any given probability function f(x),or a given cumulative distribution c(x). 1)Uniformly distributed random numbers 2)General random numbers:can be obtained from a sequence of independent uniform random numbers
Next: Using a computer to generate random events: We need to be able to generate random numbers X with any given probability function f(x), or a given cumulative distribution c(x) . 1) Uniformly distributed random numbers 2) General random numbers: can be obtained from a sequence of independent uniform random numbers
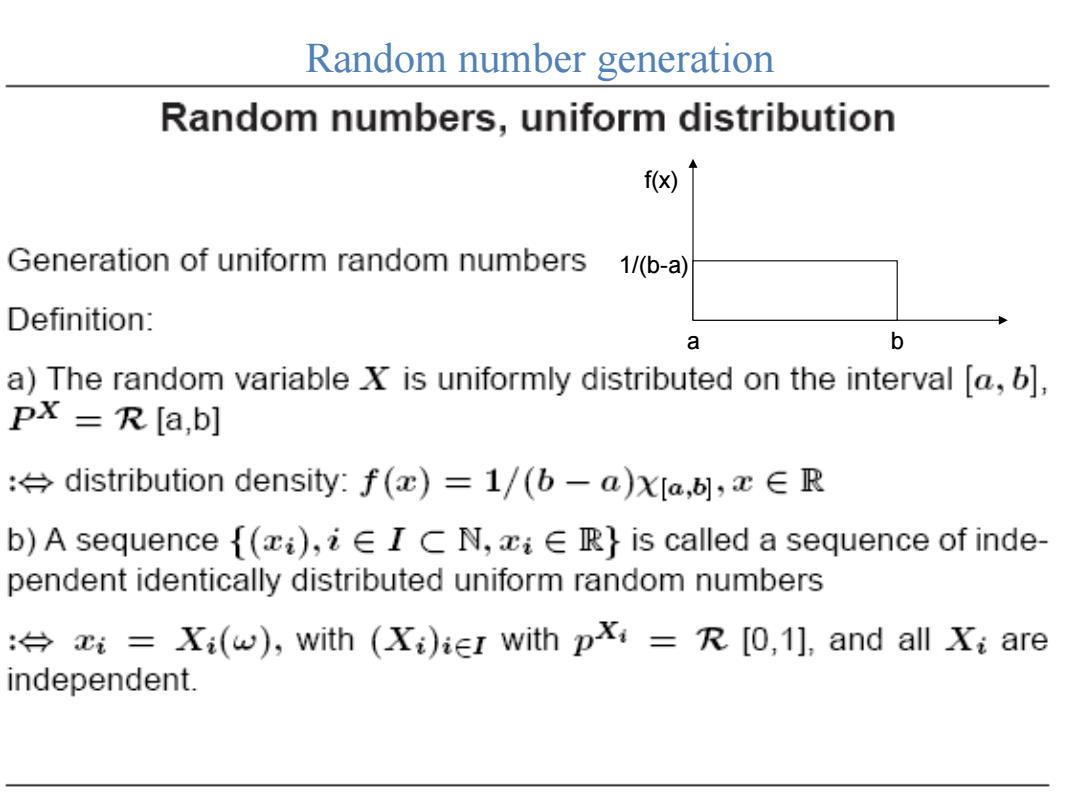
Random number generation Random numbers,uniform distribution f(x) Generation of uniform random numbers 1/(b-a) Definition: a b a)The random variable X is uniformly distributed on the interval [a,b], PX =R [a,b] :distribution density:f()=1/(b-a)x[a,6],E R b)A sequence f(xi),iE I C N,xiE R}is called a sequence of inde- pendent identically distributed uniform random numbers :=Xi(w),with (Xi)ier with p=R [0,1],and all Xi are independent
a b f(x) 1/(b-a) Random number generation