
自然数上的运算 ·加法(递归定义) o m+0=m ▣】 m+n*=(m+n)* ·乘法(递归定义) 口m*0=0 m*n+=m+m*n 这样的序关系定义,是否准确? a≤biff]c∈N.a+c=b
自然数上的运算 ◼ 加法(递归定义) ❑ m+0=m ❑ m+n+=(m+n) + ◼ 乘法(递归定义) ❑ m*0=0 ❑ m*n+=m + m*n 这样的序关系定义,是否准确? ab iff cN. a+c=b

问题2: 关于自然数有“公理”吗? 皮亚诺公理
皮亚诺公理

关于整数的公理 ■对于加法与乘法封闭: ■加法与乘法满足交换律: ■加法与乘法满足结合律; ■乘法对加法满足分配律; ■加法和乘法各自有单位元素 (0和1) ■方程十x=O有整数解(称为a的逆元素,并可由此定义“减法”) ■如果c≠0,ac=bc-→a=b(消去律) ▣(基于1,2,3…}定义“正整教”和“大于”、“小于”)对任意☑,Q>0 a=0,和a<0三者必有其一,也仅有其一; ■任一正整数的非空集合必含最小元素(良序性)
关于整数的公理 ◼ 对于加法与乘法封闭; ◼ 加法与乘法满足交换律; ◼ 加法与乘法满足结合律; ◼ 乘法对加法满足分配律; ◼ 加法和乘法各自有单位元素(0和1) ◼ 方程a+x=0有整数解(称为a的逆元素,并可由此定义“减法”) ◼ 如果c0,ac=bc → a=b(消去律) ◼ (基于{1,2,3,…}定义“正整数”和“大于”、“小于”)对任意a,a>0, a=0, 和a<0三者必有其一,也仅有其一; ◼ 任一正整数的非空集合必含最小元素(良序性)
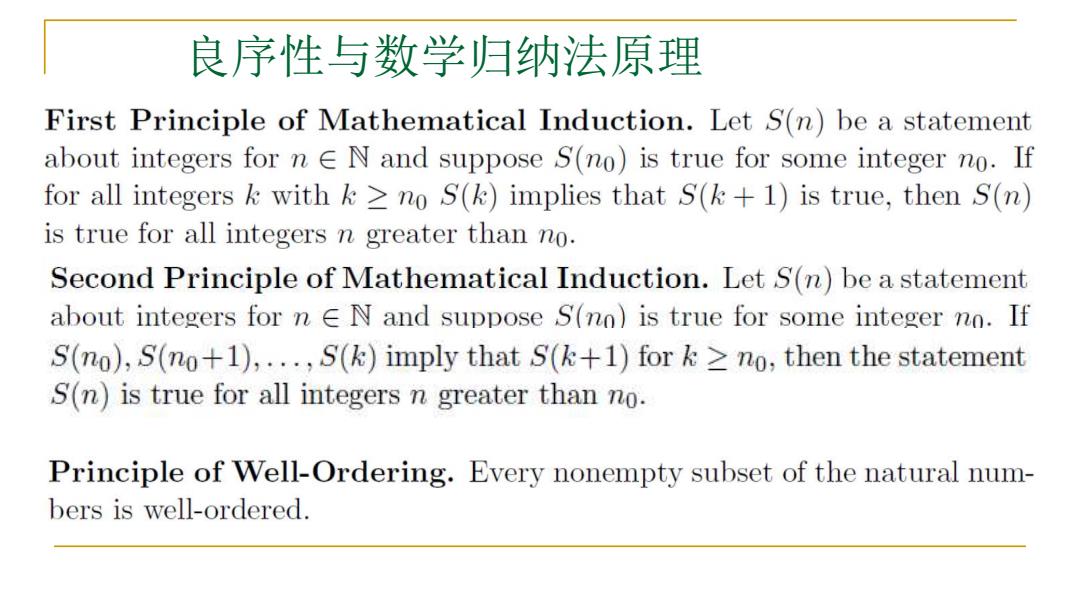
良序性与数学归纳法原理 First Principle of Mathematical Induction.Let S(n)be a statement about integers for n E N and suppose S(no)is true for some integer no.If for all integers k with k no S(k)implies that S(k+1)is true,then S(n) is true for all integers n greater than no. Second Principle of Mathematical Induction.Let S(n)be a statement about integers for n EN and suppose S(no)is true for some integer no.If S(no),S(no+1),...,S(k)imply that S(+1)for k no,then the statement S(n)is true for all integers n greater than no. Principle of Well-Ordering.Every nonempty subset of the natural num- bers is well-ordered
良序性与数学归纳法原理

间题3: 你能说说为什么归纳法原 理与良序性质是等价的吗?