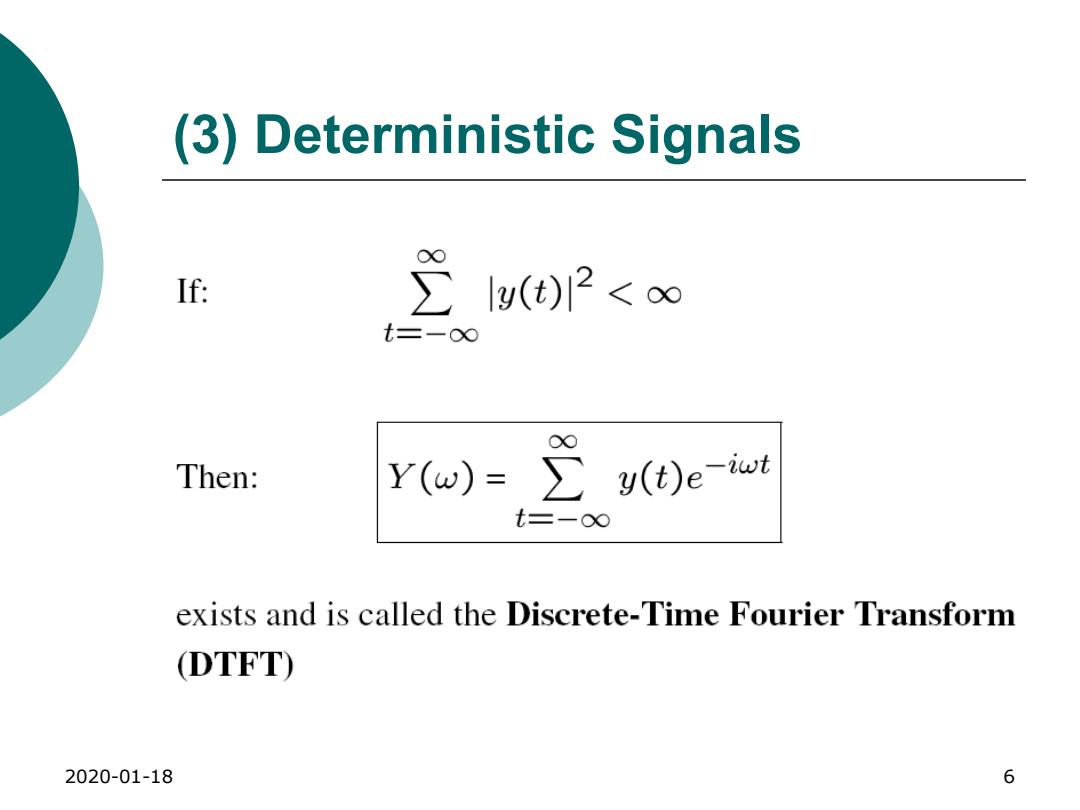
(3)Deterministic Signals If: ∑1g(t)2<o∞ 0o Then: Y(w)=∑y(t)e-iwt t=-o∞ exists and is called the Discrete-Time Fourier Transform DTFT) 2020-01-18 6
2020-01-18 6 (3) Deterministic Signals
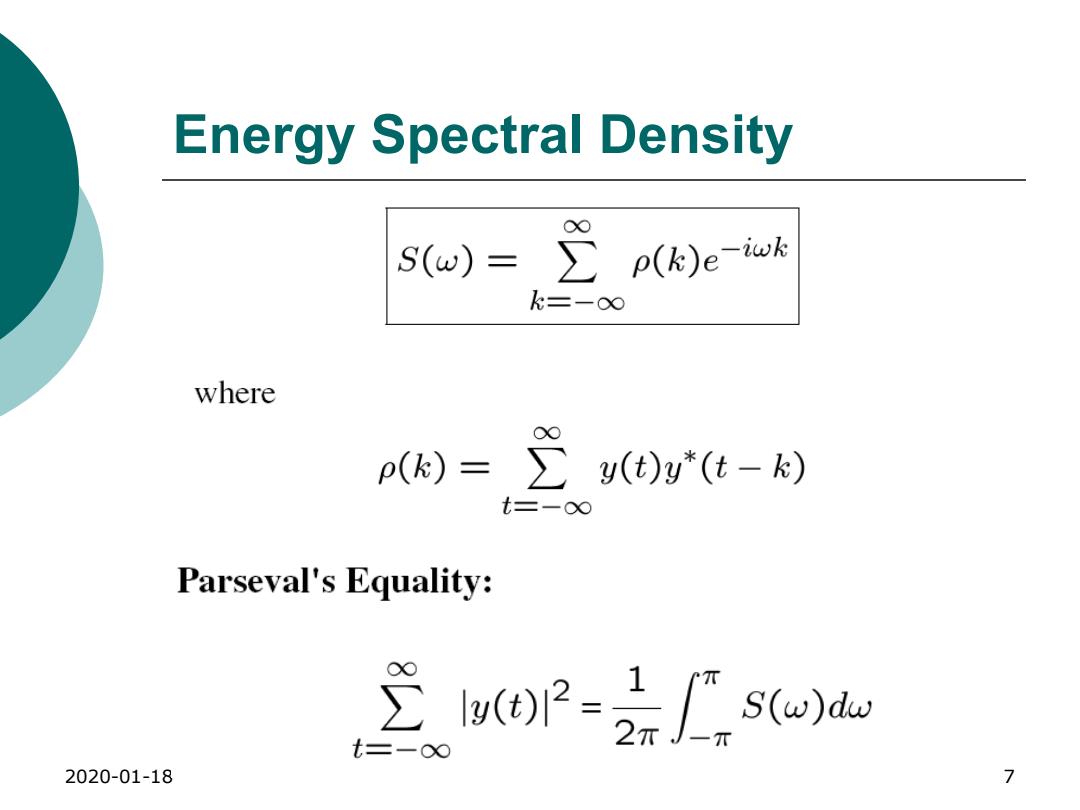
Energy Spectral Density O0 S(w)=∑p(k)eiwk k=-0∞ where p(k)=∑y(t)y*(t-k) t=-0 Parseval's Equality: 22-2 S(w)dw 2020-01-18 7
2020-01-18 7 Energy Spectral Density
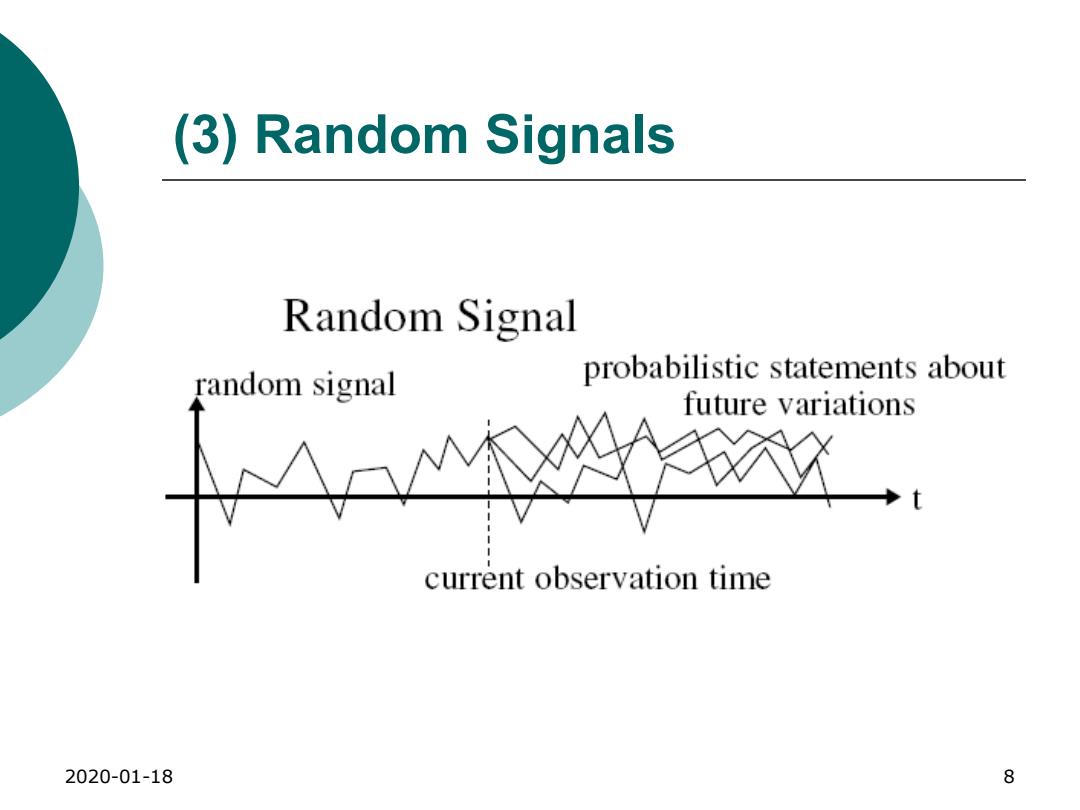
(3)Random Signals Random Signal random signal probabilistic statements about future variations △M2 current observation time 2020-01-18 8
2020-01-18 8 (3) Random Signals
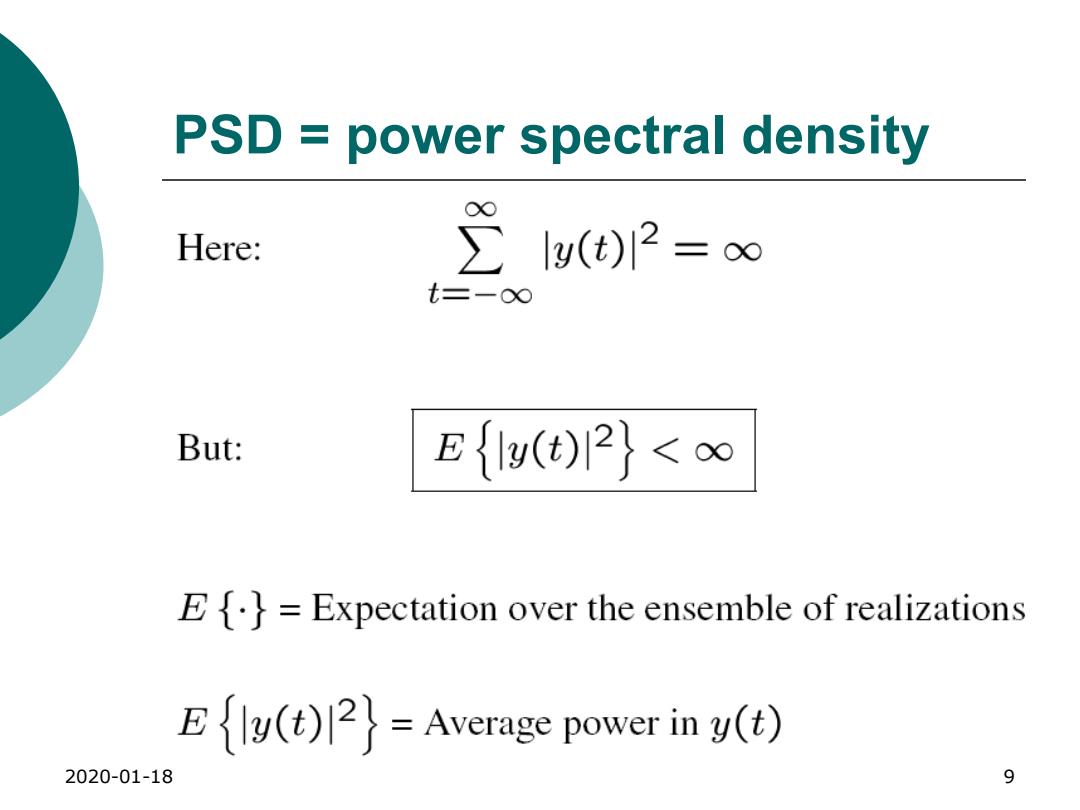
PSD power spectral density Here: 21(e)2=∞ t三-O∞ But: E{(t)12<∞ E{.}=Expectation over the ensemble of realizations (t)2=Average power in y(t) 2020-01-18 9
2020-01-18 9 PSD = power spectral density

First Definition of PSD (w)=∑r(k)e-iwk k=-0∞ where r(k)is the autocovariance sequence (ACS) r(k)=E{y(t)y*(t-k)} r(k)=r*(-k), r(0)≥r(k) 2020-01-18 10
2020-01-18 10 First Definition of PSD