
Condorcet's Paradox Consider an election with two candidates and n voters. Majority is a good idea:Whoever gets more votes wins. What about three candidates? One idea:Use pairwise comparisons. But this runs into a problem. 11
Condorcet’s Paradox ◼ Consider an election with two candidates and 𝑛 voters. ◼ Majority is a good idea: Whoever gets more votes wins. ◼ What about three candidates? ◼ One idea: Use pairwise comparisons. ◼ But this runs into a problem. 11
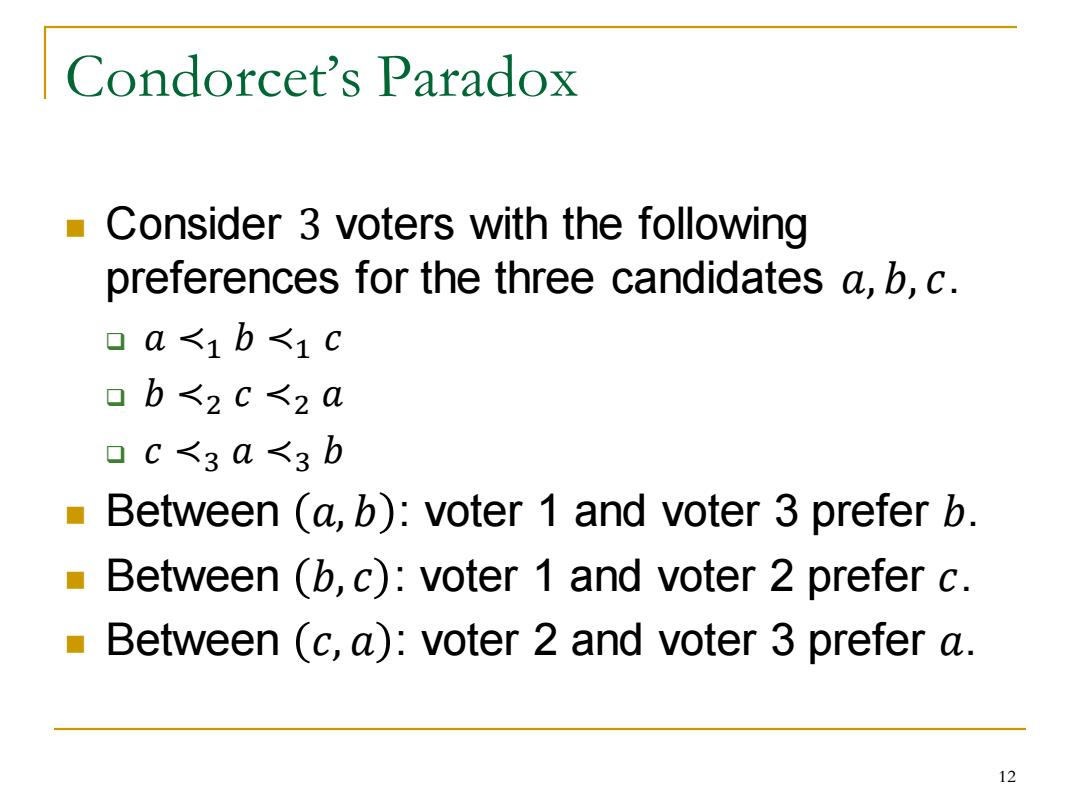
Condorcet's Paradox Consider 3 voters with the following preferences for the three candidates a,b,c. 口a<1b<1C 口b<2C<2a ▣C<3a<3b Between (a,b):voter 1 and voter 3 prefer b. Between (b,c):voter 1 and voter 2 prefer c. Between (c,a):voter 2 and voter 3 prefer a. 12
Condorcet’s Paradox ◼ Consider 3 voters with the following preferences for the three candidates 𝑎, 𝑏, 𝑐. ❑ 𝑎 ≺1 𝑏 ≺1 𝑐 ❑ 𝑏 ≺2 𝑐 ≺2 𝑎 ❑ 𝑐 ≺3 𝑎 ≺3 𝑏 ◼ Between 𝑎, 𝑏 : voter 1 and voter 3 prefer 𝑏. ◼ Between 𝑏, 𝑐 : voter 1 and voter 2 prefer 𝑐. ◼ Between 𝑐, 𝑎 : voter 2 and voter 3 prefer 𝑎. 12