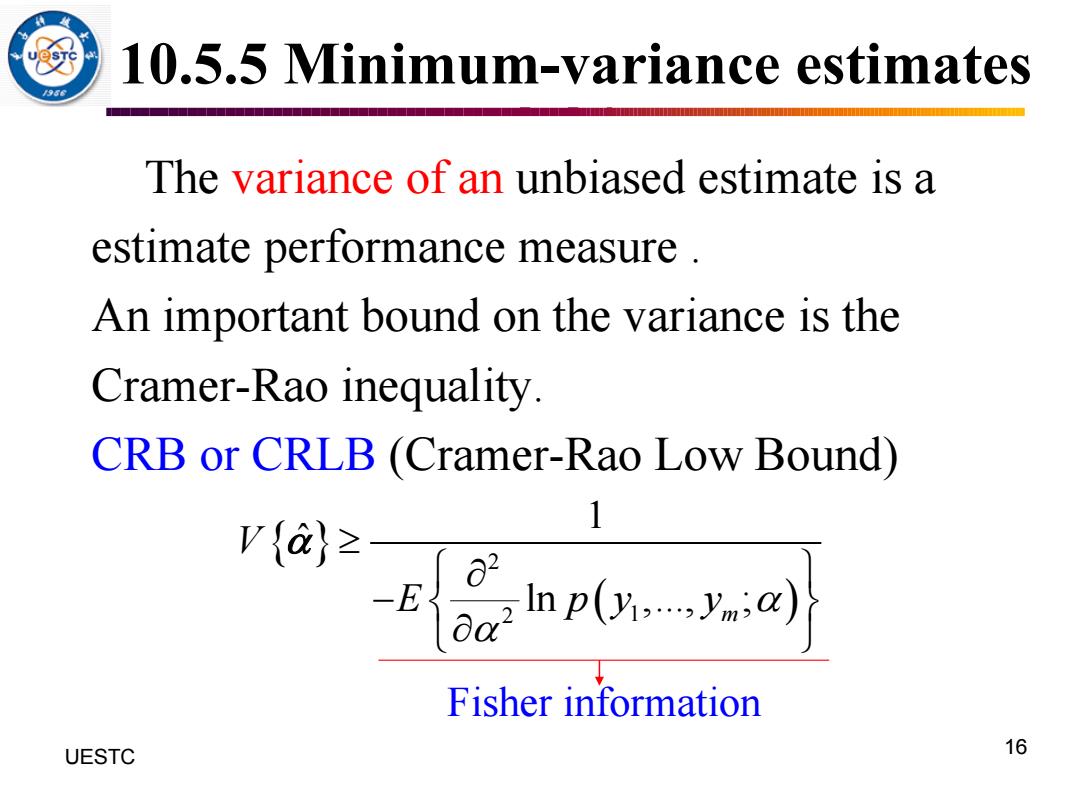
10.5.5 Minimum-variance estimates The variance of an unbiased estimate is a estimate performance measure An important bound on the variance is the Cramer-Rao inequality. CRB or CrLB (Cramer-Rao Low Bound) 1 V{a}≥ n(i Fisher information UESTC 16
16 UESTC 10.5.5 Minimum-variance estimates The variance of an unbiased estimate is a estimate performance measure . An important bound on the variance is the Cramer-Rao inequality. CRB or CRLB (Cramer-Rao Low Bound) ( ) 2 2 1 1 ˆ ln ,..., ; m V E p y y − Fisher information
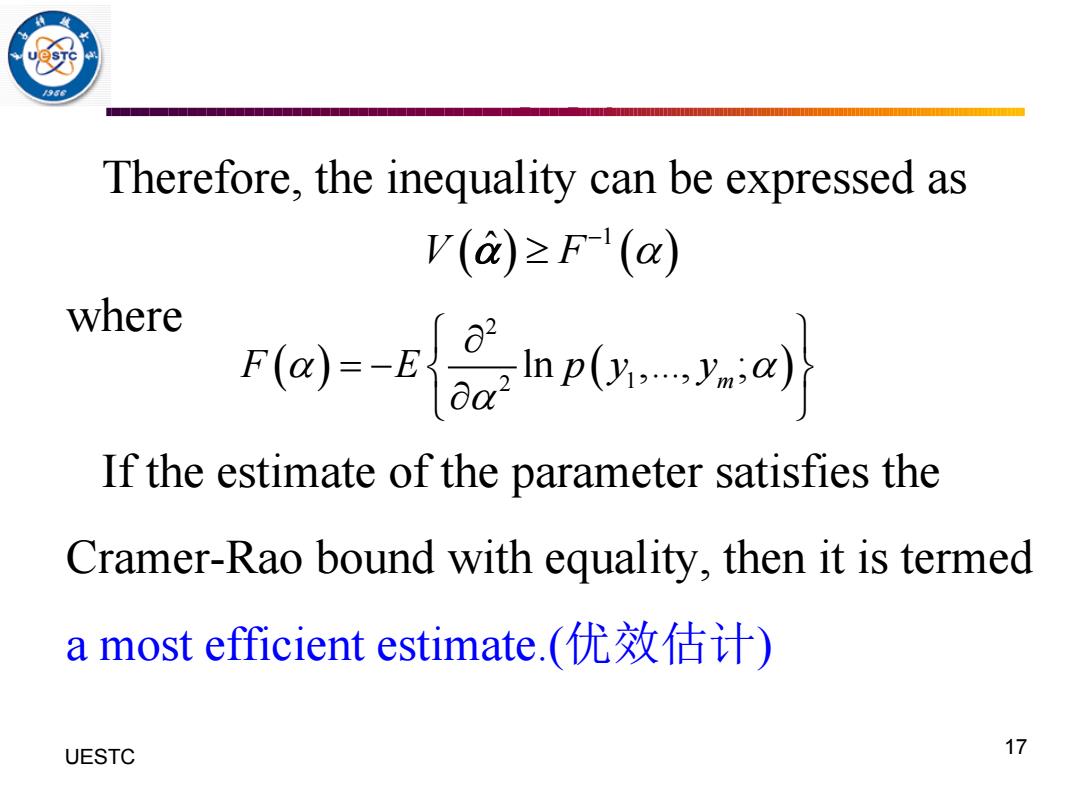
Therefore,the inequality can be expressed as V(a≥F(a) where 小品n啡 If the estimate of the parameter satisfies the Cramer-Rao bound with equality,then it is termed a most efficient estimate..(优效估计) UESTC 17
17 UESTC Therefore, the inequality can be expressed as where If the estimate of the parameter satisfies the Cramer-Rao bound with equality, then it is termed a most efficient estimate.(优效估计) ( ) ( ) 1 V F ˆ − ( ) ( ) 2 2 1 ln ,..., ; F E p y y m = −

5 An alternative form of the Cramer-Rao inequality Is 1 V(a)≥ The equality condition is obtained when alnp(yyna=F(a)[a-网个 oa where E=()p()d and F(a)is a constant that independent of y and a. UESTC 18
18 UESTC that independent of and . An alternative form of the Cramer-Rao inequality Is The equality condition is obtained when ( ) ( ) 2 1 1 ˆ ln ,..., ; m V p y y E ( ) ( ) 1 ln ,..., ; ˆ m p y y F = − where E y p y; dy ˆ ˆ ( ) ( ) − = and is a constant F ( ) y ˆ

The Proof for CRLB Proof: 「p(a)w=1 r(w.alds了2四=0 -haGro) 2@4-paw-5-0 Multiplied by a UES aad-lhg@taw-0w
19 UESTC Proof: p y dy ( ; = 1 ) ( ) ( ; ) ; = 0 p y p y d y d y = ( ) ( ) ( ) ; ln ; ln ; ( ) ; 0 p y p y p y d y p y dy E = = = ( ) ( ) ( ) ; ln ; . ; p y p y p y = ( ) ( ) ( ) ln ; ln ; ; 0 (1a) p y p y E p y dy = = The Proof for CRLB Multiplied by

Since w(a)=E{a}=∫d()p(,a=a a-jam Eq.(1b)-(1a), -ja(oeeAro=1ual -a@pw-1 [a-waano-1 UESTC 20
20 UESTC Since E y p y dy = ; ( ) = = ˆ ˆ ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ; ˆ ln ; ; =1 (1b) ˆ p y y dy p y y p y dy = = Eq.(1b)-(1a), ( ) ( ) ( ) ln ; ˆ ; 1 p y y p y dy − = ( ) ( ) ( ) ( ) 2 ln ; ˆ ; 1 p y y p y dy − =