
Review:NP theorem NP Theorem:Likelihood ratio test(LRT) .maximize Pp while a given PrA=a,decide H if L(x) P(x;H) P(x;Ho) The threshold y is determined from PEA as whxiong@uestc.edu.cn 6
whxiong@uestc.edu.cn Review: NP theorem NP Theorem: Likelihood ratio test (LRT) • maximize PD while a given PFA =α , decide H1 if 6 L(x) = P(x; H1) P(x; H0) > ° The threshold γ is determined from PFA as
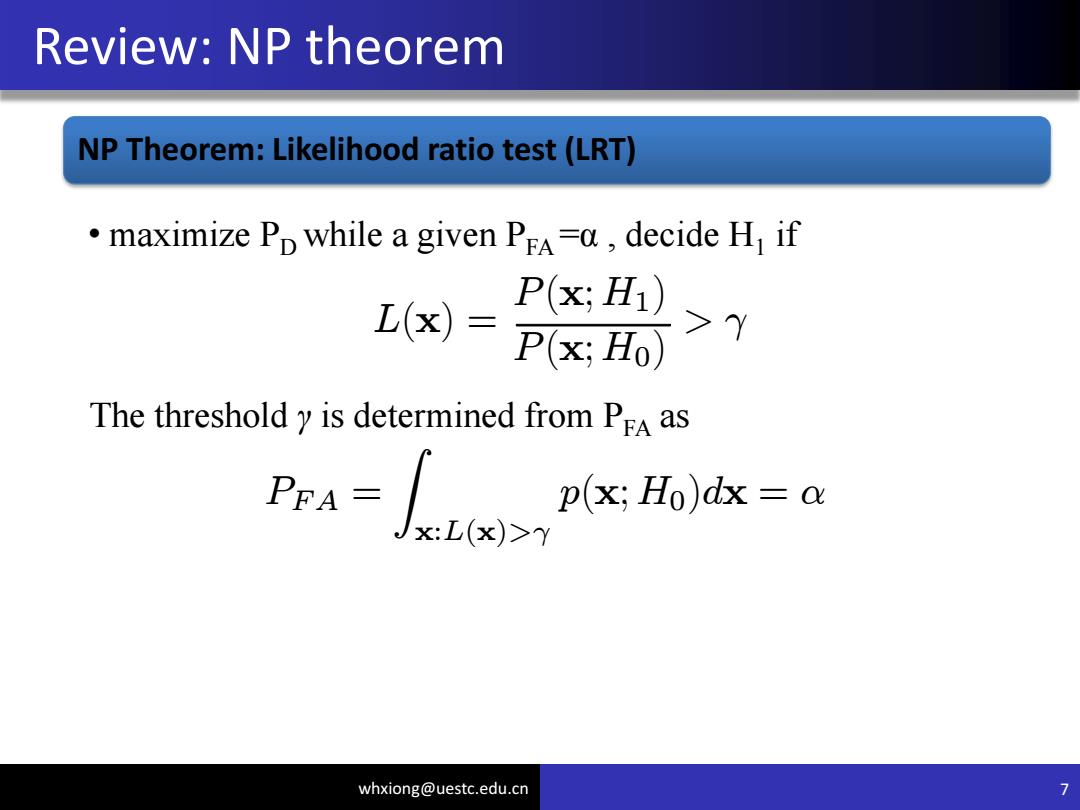
Review:NP theorem NP Theorem:Likelihood ratio test(LRT) .maximize Pp while a given PrA=a,decide H if L(x)= P(x;H) P(x;Ho) The threshold y is determined from PrA as p(x;Ho)dx =a whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Review: NP theorem NP Theorem: Likelihood ratio test (LRT) • maximize PD while a given PFA =α , decide H1 if 7 L(x) = P(x; H1) P(x; H0) > ° The threshold γ is determined from PFA as PFA = Z x:L(x)>° p(x; H0)dx = ®

Review:NP theorem NP Theorem:Likelihood ratio test(LRT) maximize Pp while a given PrA=a,decide H if L(x)= P(x;H) P(x;Ho) The threshold y is determined from PrA as p(x;Ho)dx a L(x):the likelihood ratio n-xaxH放 whxiong@uestc.edu.cn 8
whxiong@uestc.edu.cn Review: NP theorem NP Theorem: Likelihood ratio test (LRT) • maximize PD while a given PFA =α , decide H1 if 8 L(x) = P(x; H1) P(x; H0) > ° The threshold γ is determined from PFA as PFA = Z x:L(x)>° p(x; H0)dx = ® L(x): the likelihood ratio PD = Z x:L(x)>° p(x; H1)dx

Review:NP theorem { W-1 pxo)=了 N-1 p(x:H)=7 m{2w- whxiong@uestc.edu.cn
whxiong@uestc.edu.cn Review: NP theorem p(x; H1) = 1 (2¼¾2) N=2 exp ( ¡ 1 2¾2 N X¡1 n=0 [x(n) ¡ s(n)] 2 ) p(x; H0) = 1 (2¼¾2) N=2 exp ( ¡ 1 2¾2 N X¡1 n=0 [x(n)] 2 )

Review:NP theorem W-1 pxHo)=了 N-1 p(x;H)= w四{2-} Follow the NP rule W-1 L(x)=4 -{和三-ar-2可} n=0 whxiong@uestc.edu.cn 10
whxiong@uestc.edu.cn Review: NP theorem 10 p(x; H1) = 1 (2¼¾2) N=2 exp ( ¡ 1 2¾2 N X¡1 n=0 [x(n) ¡ s(n)] 2 ) p(x; H0) = 1 (2¼¾2) N=2 exp ( ¡ 1 2¾2 N X¡1 n=0 [x(n)] 2 ) Follow the NP rule L(x) = p(x; H1) p(x; H0) = exp ( ¡ 1 2¾2 N X¡1 n=0 £ (x[n] ¡ s[n]) 2 ¡ x 2 [n] ¤ )