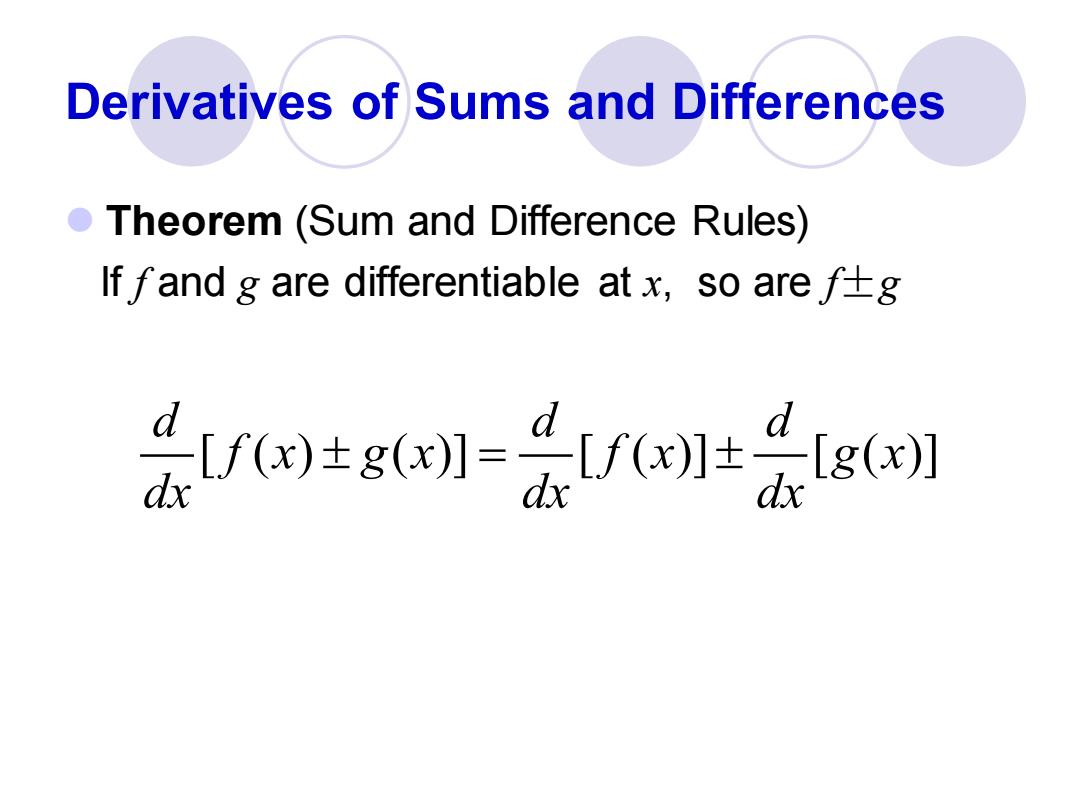
Derivatives of Sums and Differences Theorem(Sum and Difference Rules) If fand g are differentiable at x,so are fg 风L士i-云+去o
Derivatives of Sums and Differences ⚫ Theorem (Sum and Difference Rules) If f and g are differentiable at x, so are f±g [ ( ) ( )] [ ( )] [ ( )] d d d f x g x f x g x dx dx dx =
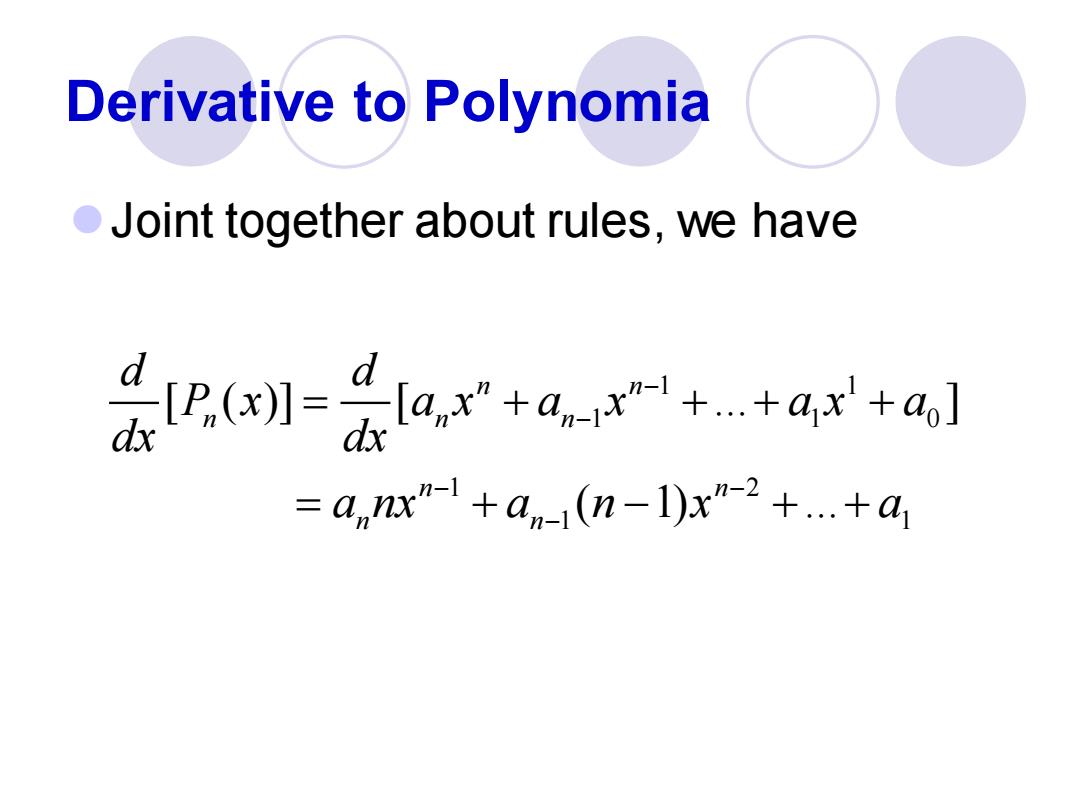
Derivative to Polynomia Joint together about rules,we have n-层.+ar+1l dx =anx"-+an-1(n-1)x"-2++a
Derivative to Polynomia ⚫Joint together about rules, we have 1 1 1 1 0 1 2 1 1 [ ( )] [ ... ] ( 1) ... n n n n n n n n n d d P x a x a x a x a dx dx a nx a n x a − − − − − = + + + + = + − + +
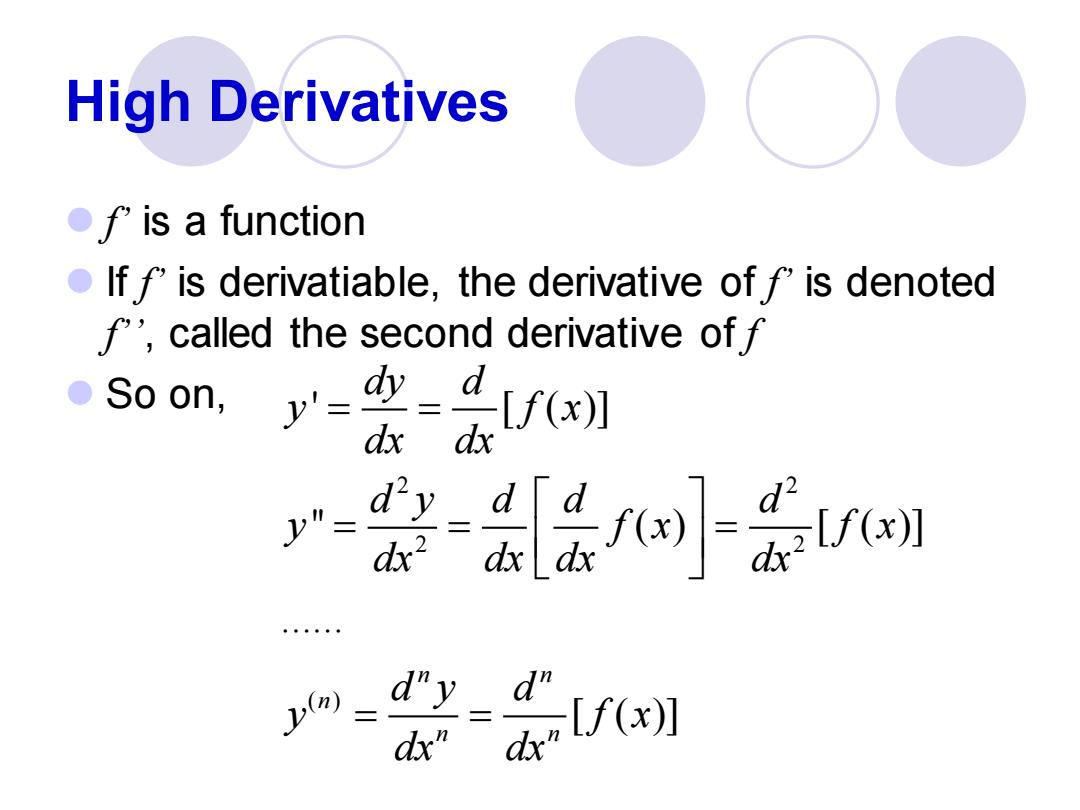
High Derivatives ●fis a function oIf f is derivatiable,the derivative of f is denoted /called the second derivative off ●S0on,y'=水=d[/x] dx dx [ d y)=d"y dn dx"dx" f(x)〗
High Derivatives ⚫ f’ is a function ⚫ If f’ is derivatiable, the derivative of f’ is denoted f’’, called the second derivative of f ⚫ So on, 2 2 2 2 ( ) ' [ ( )] '' ( ) [ ( )] ...... [ ( )] n n n n n dy d y f x dx dx d y d d d y f x f x dx dx dx dx d y d y f x dx dx = = = = = = =