
Chapter Six Intergration
Chapter Six Intergration

The Area Problem Given a function fthat is continuous and nonnegative on an interval [a,b],find the area between the graph of f and the interval [a,b]on the x-axis
The Area Problem ⚫Given a function f that is continuous and nonnegative on an interval [a,b], find the area between the graph of f and the interval [a,b] on the x-axis

Antiderivative ●Definition A function F is called an antiderivative of a functionf on a given interval Iif F(x)=fx) for all x in the interval
Antiderivative ⚫Definition A function F is called an antiderivative of a function f on a given interval I if F’(x) =f(x) for all x in the interval
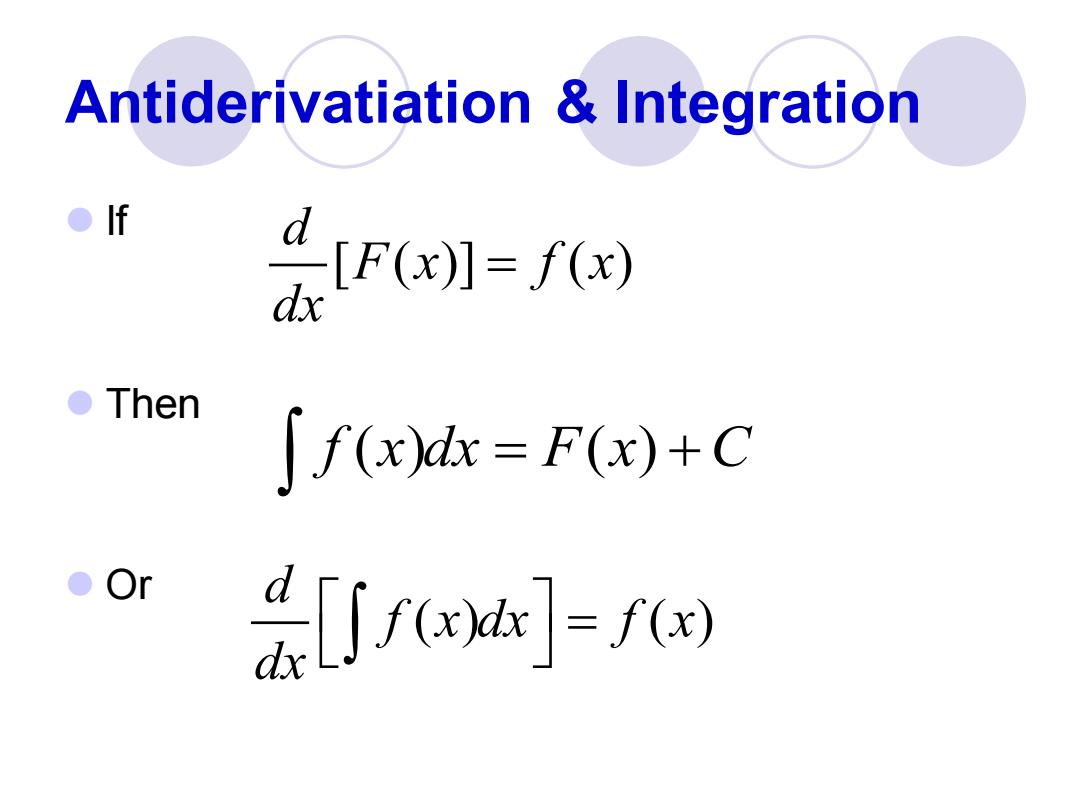
Antiderivatiation Integration ●f 2r0划0网 Then ∫f(x)dx=F(x)+C ●O ]-fo
Antiderivatiation & Integration ⚫ If ⚫ Then ⚫ Or [ ( )] ( ) d F x f x dx = f x dx F x C ( ) ( ) = + ( ) ( ) d f x dx f x dx =
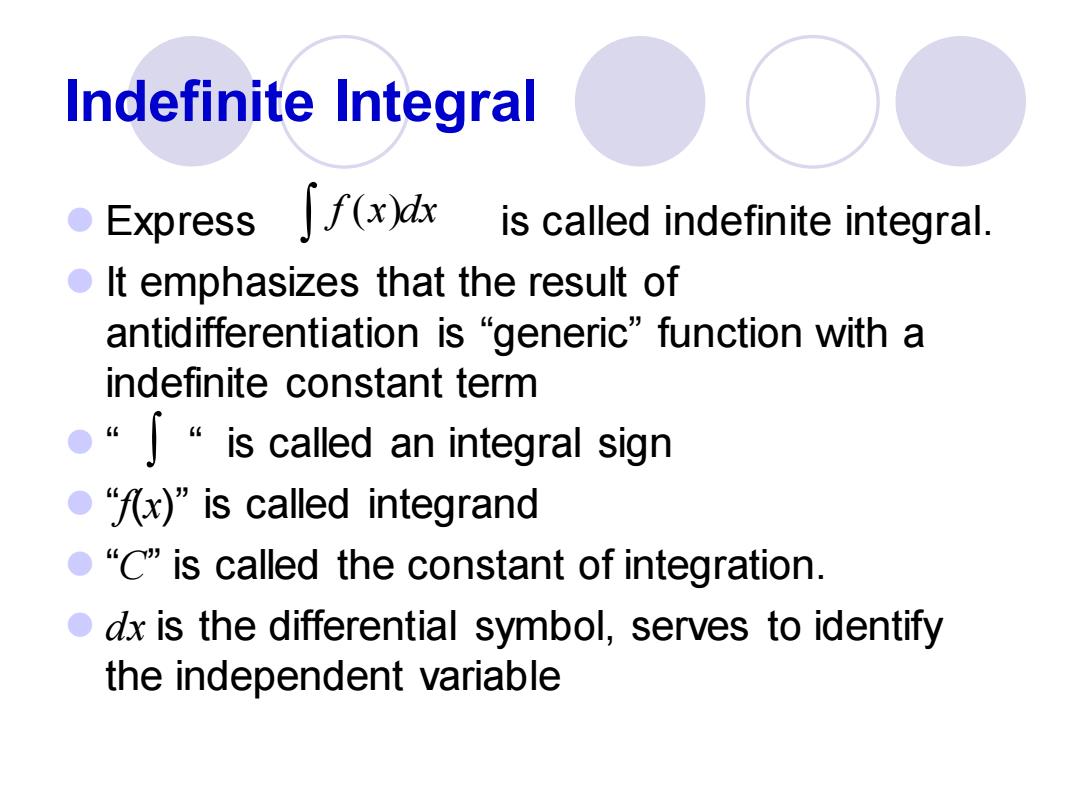
Indefinite Integral Express∫f(x)k is called indefinite integral. It emphasizes that the result of antidifferentiation is "generic"function with a indefinite constant term ●“J“is called an integral sign ●“fx)”is called integrand ●“C"is called the constant of integration. odx is the differential symbol,serves to identify the independent variable
Indefinite Integral ⚫ Express is called indefinite integral. ⚫ It emphasizes that the result of antidifferentiation is “generic” function with a indefinite constant term ⚫ “ “ is called an integral sign ⚫ “f(x)” is called integrand ⚫ “C” is called the constant of integration. ⚫ dx is the differential symbol, serves to identify the independent variable f x dx ( )