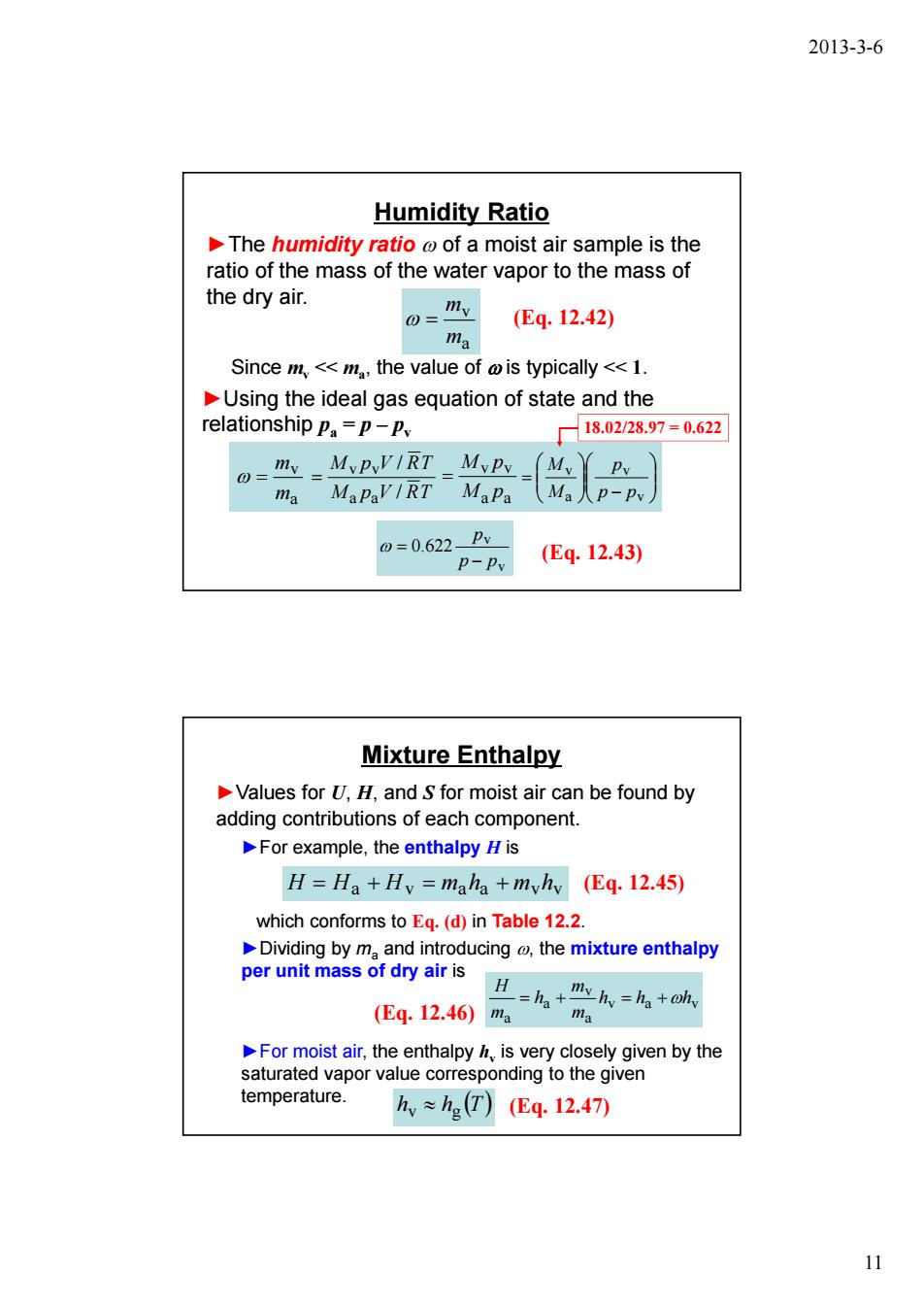
2013-3-6 Humidity Ratio The humidity ratio of a moist air sample is the ratio of the mass of the water vapor to the mass of the dry air. (Eq.12.42) ma Since m<<m,the value of is typically <<1. Using the ideal gas equation of state and the relationship p,=p-p 18.02/28.97=0.622 =MyP,y/RT Mypy ma MapaV/RT MaPa Ma八p-pw 0=0.622D p-Pv (Eq.12.43) Mixture Enthalpy Values for U,H,and S for moist air can be found by adding contributions of each component. For example,the enthalpy H is H=Ha +Hy=maha +mhy (Eq.12.45) which conforms to Eq.(d)in Table 12.2. (E4.12.46)ma For moist air,the enthalpyis very closely given by the saturated vapor value corresponding to the given temperature. hv≈he(T)(Eq.12.47) 11
2013-3-6 11 Humidity Ratio ►The humidity ratio ω of a moist air sample is the ratio of the mass of the water vapor to the mass of the dry air. a v m m ω = (Eq. 12.42) v v 0.622 p p p − ω = (Eq. 12.43) Since mv << ma, the value of ω is typically << 1. ►Using the ideal gas equation of state and the relationship pa = p – pv a v m m ω = M p V RT M p V RT / / a a v v = a a v v M p M p = ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ − ⎟ ⎟ ⎠ ⎞ ⎜ ⎜ ⎝ ⎛ = v v a v p p p M M 18.02/28.97 = 0.622 Mixture Enthalpy ►Values for U, H, and S for moist air can be found by adding contributions of each component. (Eq. 12.47) ►For example, the enthalpy H is which conforms to Eq. (d) in Table 12.2. ►Dividing by ma and introducing ω, the mixture enthalpy per unit mass of dry air is ►For moist air, the enthalpy hv is very closely given by the saturated vapor value corresponding to the given temperature. H = Ha + Hv = maha + mvhv (Eq. 12.45) v a v a v a a h h h m m h m H = + = +ω (Eq. 12.46) h h (T ) v ≈ g

2013-3-6 Heating Moist Air in a Duct Example:Moist air enters a duct at 10C,80% relative humidity,is heated as it flows through the duct,and exits at 30C.No moisture is added or removed and the mixture pressure remains constant at 1 bar.For steady-state operation and ignoring kinetic and potential energy changes,determine (a)the humidity ratioo and (b)the rate of heat transfer,in kJ per kg of dry air SOe T,=10°C 中1=80% 73=30C Boundary Heating Moist Air in a Duct Solution: (a)At steady state,mass rate balances for the dry air and water vapor read: rial =rita2 (dry air) riv=riv2 (water vapor) Since the mass flow rates of the dry air and water vapor do not change from inlet to exit,they are denoted for simplicity asi,and it.Moreover,since no moisture is added or r emoved,the humidity ratio does not change from inlet to exit:=@.The common humidity ratio is denoted by @ 0= 12
2013-3-6 12 Heating Moist Air in a Duct Example: Moist air enters a duct at 10oC, 80% relative humidity, is heated as it flows through the duct, and exits at 30oC. No moisture is added or removed and the mixture pressure remains constant at 1 bar. For steady-state operation and ignoring kinetic and potential energy changes, determine (a) the humidity ratio, ω2, and (b) the rate of heat transfer, in kJ per kg of dry air. Heating Moist Air in a Duct (a) At steady state, mass rate balances for the dry air and water vapor read: Solution: (water vapor) (dry air) v1 v2 a1 a2 m m m m & & & & = = a v m m & & ω = Since the mass flow rates of the dry air and water vapor do not change from inlet to exit, they are denoted for simplicity as ma and mv. Moreover, since no moisture is added or removed, the humidity ratio does not change from inlet to exit: ω1 = ω2. The common humidity ratio is denoted by ω. · ·