
2013-3-6 Chapter 4 Control Volume Analysis Learning Outcomes Demonstrate understanding of key concepts related to control volume analysis including distinguishing between steady-state and transient analysis,distinguishing between mass flow rate and volumetric flow rate,and the meanings of one-dimensional flow and flow work. Apply mass and energy balances to control volumes. 4-2
2013-3-6 1 Chapter 4 Control Volume Analysis Learning Outcomes ►Demonstrate understanding of key concepts related to control volume analysis including distinguishing between steady-state and transient analysis, distinguishing between mass flow rate and volumetric flow rate, and the meanings of one-dimensional flow and flow work. ►Apply mass and energy balances to control volumes. 4-2

2013-3-6 Learning Outcomes,cont. Develop appropriate engineering models for control volumes,with particular attention to analyzing components commonly encountered in engineering practice such as nozzles, diffusers,turbines,compressors,heat exchangers,throttling devices,and integrated systems that incorporate two or more components. Use property data in control volume analysis appropriately. 4-3 Conservation of mass Conservation of mass:Mass,like energy,is a conserved property,and it cannot be created or destroyed during a process. Closed systems(implicitly used):The mass of the system remain constant during a process. Control volumes:Mass can cross the boundaries and so we must keep track of the amount of mass entering and leaving the control volume. 2kg H2 + 16kg 18kg 02 H20 Mass is conserved even during chemical reactions Mass m and energy E equivalence E=mc where c=speed of light in vacuum 108
2013-3-6 2 Learning Outcomes, cont. ►Develop appropriate engineering models for control volumes, with particular attention to analyzing components commonly encountered in engineering practice such as nozzles, diffusers, turbines, compressors, heat exchangers, throttling devices, and integrated systems that incorporate two or more components. ►Use property data in control volume analysis appropriately. 4-3 Conservation of mass Mass is conserved even during chemical reactions. ►Conservation of mass: Mass, like energy, is a conserved property, and it cannot be created or destroyed during a process. ►Closed systems (implicitly used): The mass of the system remain constant during a process. ►Control volumes: Mass can cross the boundaries, and so we must keep track of the amount of mass entering and leaving the control volume. Mass m and energy E equivalence where c = speed of light in vacuum (c = 2.9979 × 108 m/s) The mass change due to energy change is negligible

2013-3-6 Conservation of Mass Principle Total mass entering Total mass leaving Net change in mass the CV during△t 、the CV during△i, (within the CV during△r 1in-1mout=△11cy mn=50kg min -mou dmcv/dt =风=20 Conservation of mass :30 kg principle for a bathtub Mass Rate Balance Conservation of mass principle time rate offlow control volume me inleti at timet exit e at timet Dashed line >Or in symbols me (Eq.4a) dt where Mass Rate Balance for a CV ri is the mass flow rate=amount of mass flowing through a cross section per unit time Dot over symbol denotes the time rate of change 4-6 3
2013-3-6 3 Conservation of Mass Principle Conservation of mass principle for a bathtub Mass Rate Balance 4-6 time rate of change of mass contained within the control volume at time t time rate of flow of mass in across inlet i at time t time rate of flow of mass out across exit e at time t mi me dt dm = & − & cv (Eq. 4a) Mass Rate Balance for a CV where is the mass flow rate = amount of mass flowing through a cross section per unit time m& Dot over symbol denotes the time rate of change Conservation of mass principle ¾ Or in symbols
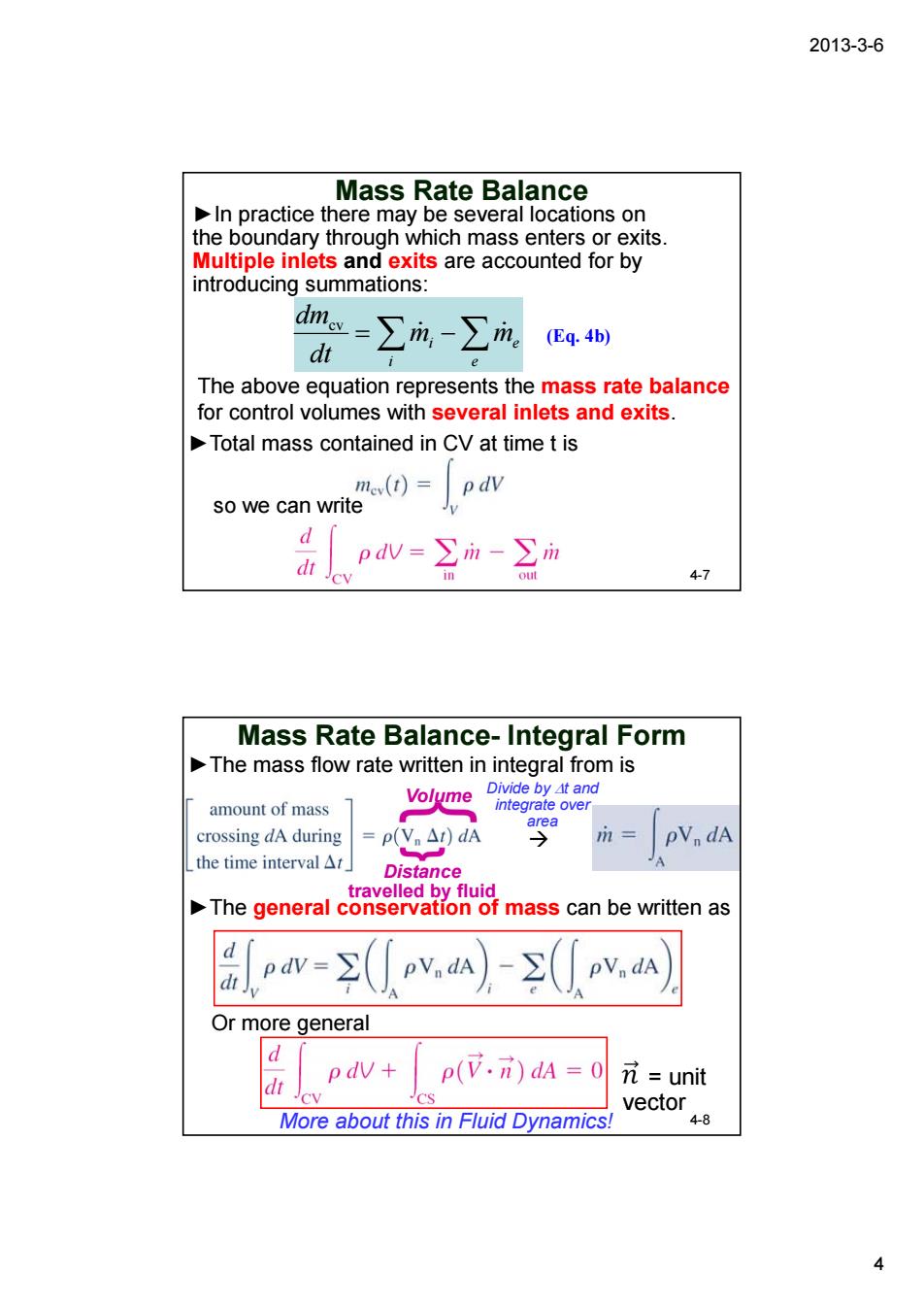
2013-3-6 Mass Rate Balance In practice there may be several locations on the boundary through which mass enters or exits. and its are accounted for by introducing summations: d=∑m-∑m44w dt The above equation represents the mass rate balance for control volumes with several inlets and exits. Total mass contained in CV at time t is so we can(v d d pdW=∑m-∑m 47 Mass Rate Balance-Integral Form The mass flow rate written in integral from is amount of mass Volyme crossing dA during p(v Ar)dA pV dA the time interval△t fluid The general comass can be written as ,pw=(pva)-(pv,dA d Or more general d p(.元)dA = d 元=unit vector More about this in Fluid Dynamics! 48 g
2013-3-6 4 Mass Rate Balance = ∑ −∑ e e i mi m dt dm & & cv (Eq. 4b) The above equation represents the mass rate balance for control volumes with several inlets and exits. ►In practice there may be several locations on the boundary through which mass enters or exits. Multiple inlets and exits are accounted for by introducing summations: 4-7 ►Total mass contained in CV at time t is so we can write Mass Rate Balance- Integral Form 4-8 ►The general conservation of mass can be written as Or more general ►The mass flow rate written in integral from is Æ Distance travelled by fluid Volume = unit vector More about this in Fluid Dynamics! Divide by Δt and integrate over area

2013-3-6 Mass Flow Rate (One-Dimensional Flow) Flow is normal to the boundary at locations where mass enters or exits the control volume All intensive properties are uniform with position over each inlet or exit area(A) through which matter flows. Mi=PAV=AV (Eq.4c) 7) where V is velocity_(not volume v is specific volume 49 Mass Rate Balance (Steady-State Form) Steady-state:all properties are unchanging in time For steady-state control volume,di/dt=0. ∑m;=∑me 1 (Eq.4d) mass rate mass rate OUT For single stream (one input one output) devices:nozzles,turbines,compressors,pumps m=m。 or VA=,A Steady Incompressible flow(single stream) most liquids VA=V24 4-10 5
2013-3-6 5 Mass Flow Rate (One-Dimensional Flow) ►Flow is normal to the boundary at locations where mass enters or exits the control volume. ►All intensive properties are uniform with position over each inlet or exit area (A) through which matter flows. v AV m& = ρAV = (Eq. 4c) where V is velocity (not volume) v is specific volume 4-9 ►For single stream (one input & one output) devices: nozzles, turbines, compressors, pumps: ►Steady Incompressible flow (single stream) Æ most liquids Mass Rate Balance (Steady-State Form) ►Steady-state: all properties are unchanging in time ►For steady-state control volume, dmcv / dt = 0. (Eq. 4d) mass rate IN mass rate OUT 4-10 or ∑ = ∑ e e i m&i m& V1A1 =V2A2 mi me & = & ρ1 V1A1 = ρ 2 V2A2