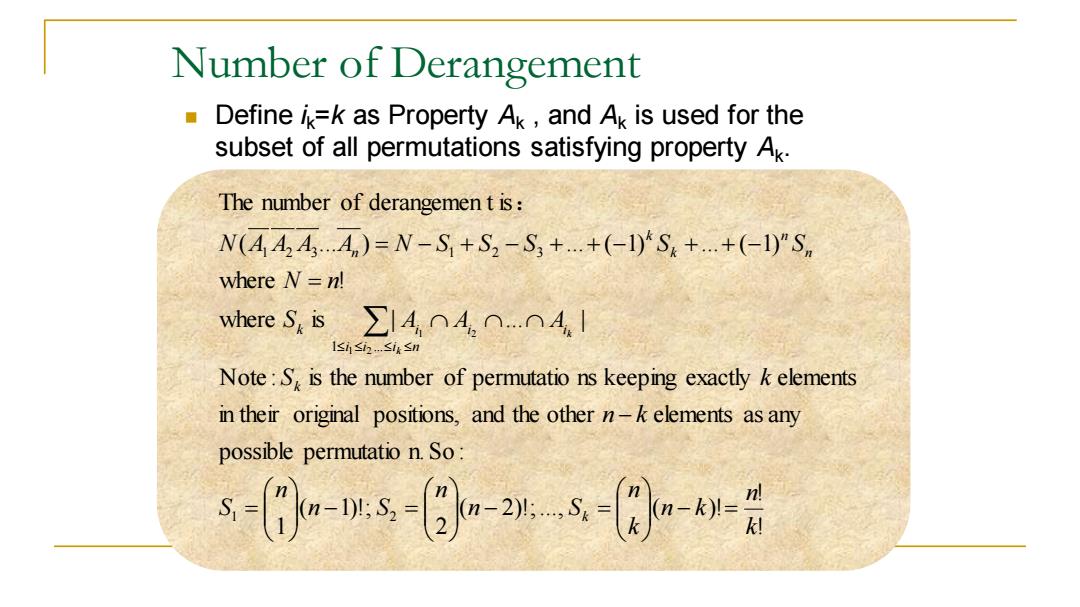
Number of Derangement Define i=k as Property Ak,and Ak is used for the subset of all permutations satisfying property Ak. The number of derangemen t is: N(AA2A.An)=N-S+S2-S3+.+(-1)S.++(-1)”S where N=n! where S is 144.4 1≤h≤i2≤ik≤n Note:S is the number of permutatio ns keeping exactly k elements in their original positions,and the other n-kelements as any possible permutatio n.So: s0s8-y--是
Number of Derangement ◼ Define ik=k as Property Ak , and Ak is used for the subset of all permutations satisfying property Ak . ! ! ( 2)!;..., ( )! 2 ( 1)!; 1 possible permutatio n. So : in their original positions, and the other elements as any Note : is the number of permutatio ns keeping exactly elements where is | ... | where ! ( ... ) ... ( 1) ... ( 1) The number of derangemen t is 1 2 1 ... 1 2 3 1 2 3 1 2 1 2 k n n k k n n S n n S n S n k S k S A A A N n N A A A A N S S S S S k k i i i n k i i i n n k k n k k − = − = − = = − = = − + − + + − + + − :
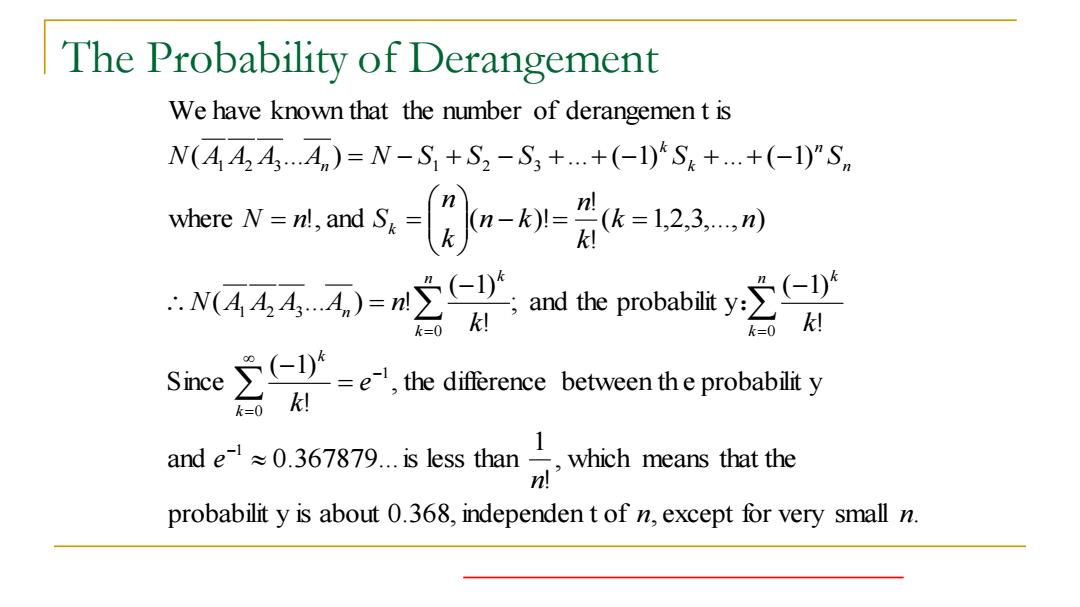
The Probability of Derangement We have known that the number of derangemen t is N(AAA,.An)=N-S+S2-S3+.+(-1)Sk+.+(-I)”Sm wtere Nn d y) and the probabit y) ∴N(444A)=n-10 k=0 Sinethe difrence between the probabilty and e0.367879...is less than which means that the nl probabilit y is about 0.368,independen t of n,except for very small n
The Probability of Derangement probabilit y is about 0.368, independen t of , except for very small . , which means that the ! 1 and 0.367879...is less than , the difference between th e probabilit y ! ( 1) Since ! ( 1) ; and the probabilit y ! ( 1) ( ... ) ! ( 1,2,3,..., ) ! ! where !, and ( )! ( ... ) ... ( 1) ... ( 1) We have known that the number of derangemen t is 1 1 0 0 0 1 2 3 1 2 3 1 2 3 n n n e e k k k N A A A A n k n k n n k k n N n S N A A A A N S S S S S k k n k n k k k n k n n k k n = − − − = − = = = = = − + − + + − + + − − − = = = :