
Analog-to-Digital Conversion Preview Measure of Information Quantization
Analog-to-Digital Conversion Preview Measure of Information Quantization

Why ADC Many sources are Analog by nature Speech,Image,Telemetry sources,. ■Digital is easier ■To process ■To communicate ■To store
Why ADC ? ◼ Many sources are Analog by nature ◼ Speech, Image, Telemetry sources, . ◼ Digital is easier ◼ To process ◼ To communicate ◼ To store
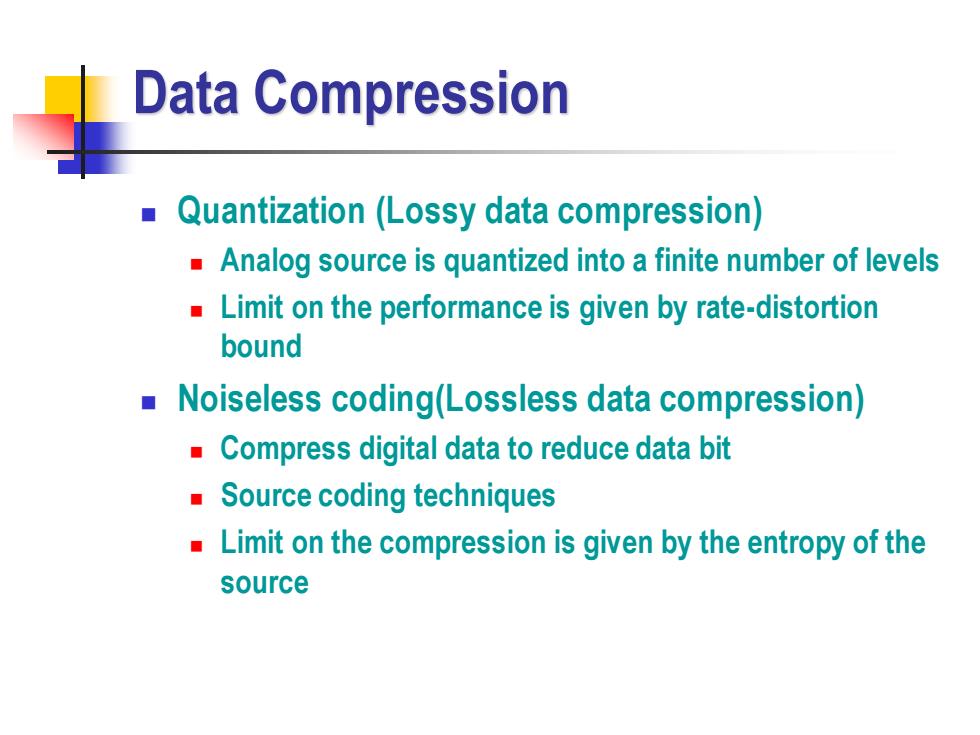
Data Compression Quantization (Lossy data compression) Analog source is quantized into a finite number of levels Limit on the performance is given by rate-distortion bound Noiseless coding(Lossless data compression) Compress digital data to reduce data bit Source coding techniques Limit on the compression is given by the entropy of the source
Data Compression ◼ Quantization (Lossy data compression) ◼ Analog source is quantized into a finite number of levels ◼ Limit on the performance is given by rate-distortion bound ◼ Noiseless coding(Lossless data compression) ◼ Compress digital data to reduce data bit ◼ Source coding techniques ◼ Limit on the compression is given by the entropy of the source
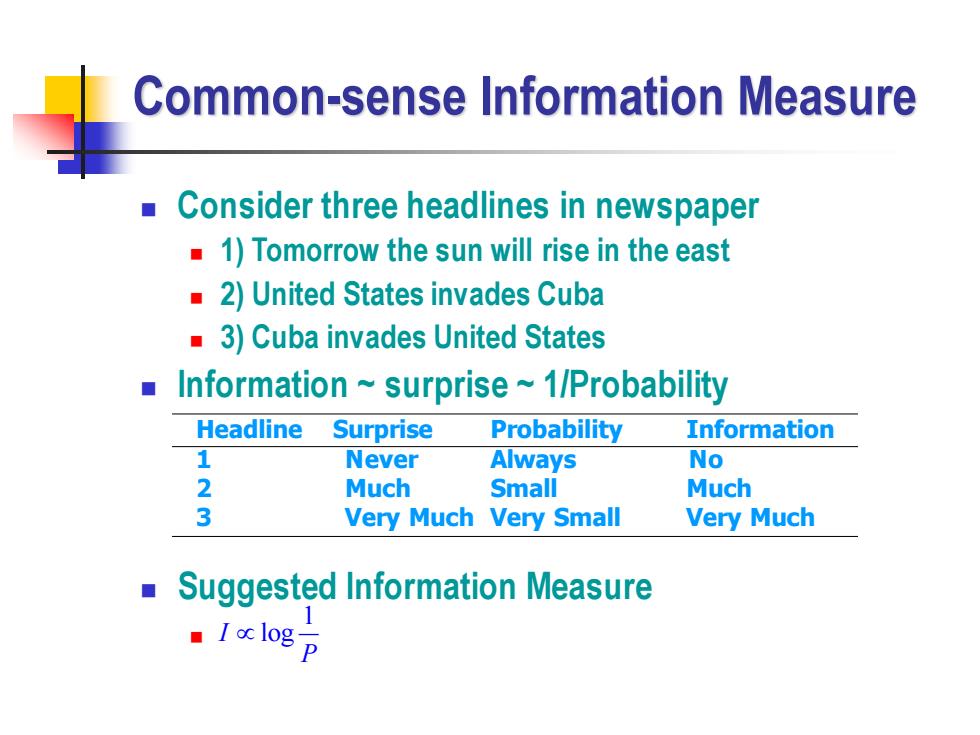
Common-sense Information Measure Consider three headlines in newspaper 1)Tomorrow the sun will rise in the east ■ 2)United States invades Cuba 3)Cuba invades United States Information surprise ~1/Probability Headline Surprise Probability Information 1 Never Always No 2 Much Small Much 3 Very Much Very Small Very Much Suggested Information Measure I oclog p
Common-sense Information Measure ◼ Consider three headlines in newspaper ◼ 1) Tomorrow the sun will rise in the east ◼ 2) United States invades Cuba ◼ 3) Cuba invades United States ◼ Information ~ surprise ~ 1/Probability ◼ Suggested Information Measure ◼ 1 I log P Headline Surprise Probability Information 1 Never Always No 2 Much Small Much 3 Very Much Very Small Very Much
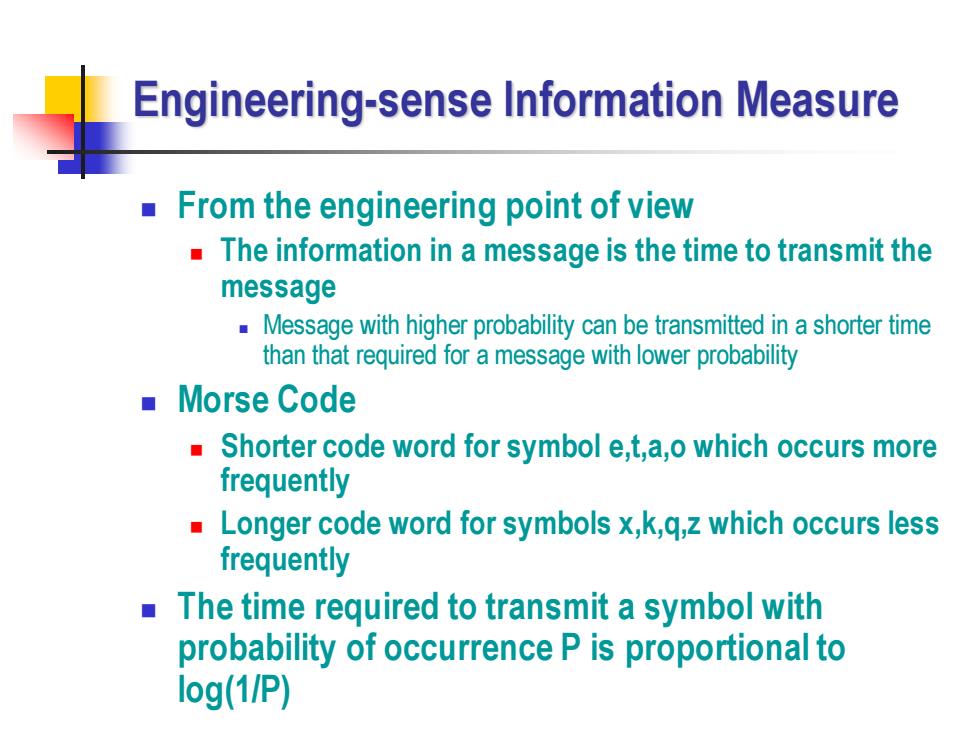
Engineering-sense Information Measure From the engineering point of view The information in a message is the time to transmit the message Message with higher probability can be transmitted in a shorter time than that required for a message with lower probability ■Morse Code Shorter code word for symbol e,t,a,o which occurs more frequently Longer code word for symbols x,k,q,z which occurs less frequently The time required to transmit a symbol with probability of occurrence P is proportional to log(1/P)
Engineering-sense Information Measure ◼ From the engineering point of view ◼ The information in a message is the time to transmit the message ◼ Message with higher probability can be transmitted in a shorter time than that required for a message with lower probability ◼ Morse Code ◼ Shorter code word for symbol e,t,a,o which occurs more frequently ◼ Longer code word for symbols x,k,q,z which occurs less frequently ◼ The time required to transmit a symbol with probability of occurrence P is proportional to log(1/P)