Continuous model ■Consider2=[0,1]. Any number in the interval is a possible outcome. Assume uniform distribution:all outcomes happen equally likely. Then what's the probability of“½”asan outcome? What if you replace 2 with any of your favorite numbers?
Continuous Model Consider Ω = 0,1 . Any number in the interval is a possible outcome. Assume uniform distribution: all outcomes happen equally likely. Then what’s the probability of “½” as an outcome? What if you replace ½ with any of your favorite numbers?
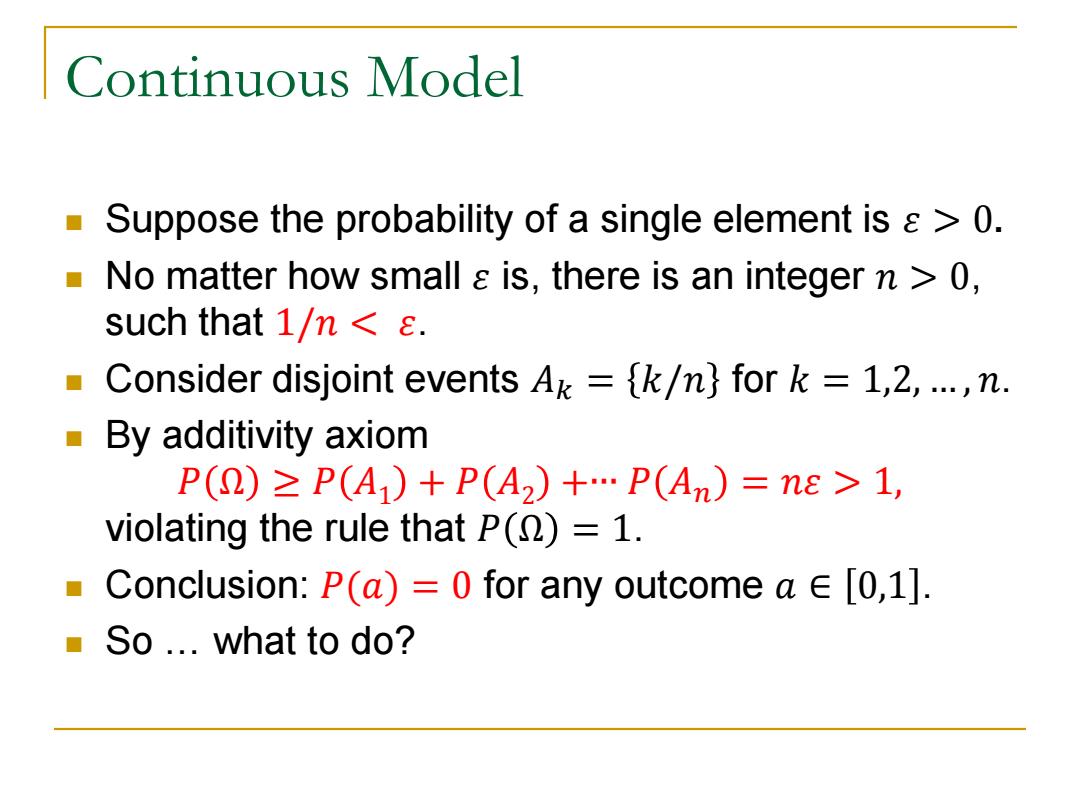
Continuous Model Suppose the probability of a single element is s>0. No matter how small s is,there is an integer n 0, such that 1/n s. Consider disjoint events A=k/n}for k 1,2,...n By additivity axiom P(2)≥P(A)+P(A2)+…P(An)=ne>1, violating the rule that P()=1. Conclusion:P(a)=0 for any outcome a e [0,1]. So...what to do?
Continuous Model Suppose the probability of a single element is 𝜀 > 0. No matter how small 𝜀 is, there is an integer 𝑛 > 0, such that 1/𝑛 < 𝜀. Consider disjoint events 𝐴𝑘 = 𝑘/𝑛 for 𝑘 = 1,2, … , 𝑛. By additivity axiom 𝑃 Ω ≥ 𝑃 𝐴1 + 𝑃 𝐴2 +∙∙∙ 𝑃 𝐴𝑛 = 𝑛𝜀 > 1, violating the rule that 𝑃 Ω = 1. Conclusion: 𝑃(𝑎) = 0 for any outcome 𝑎 ∈ 0,1 . So … what to do?
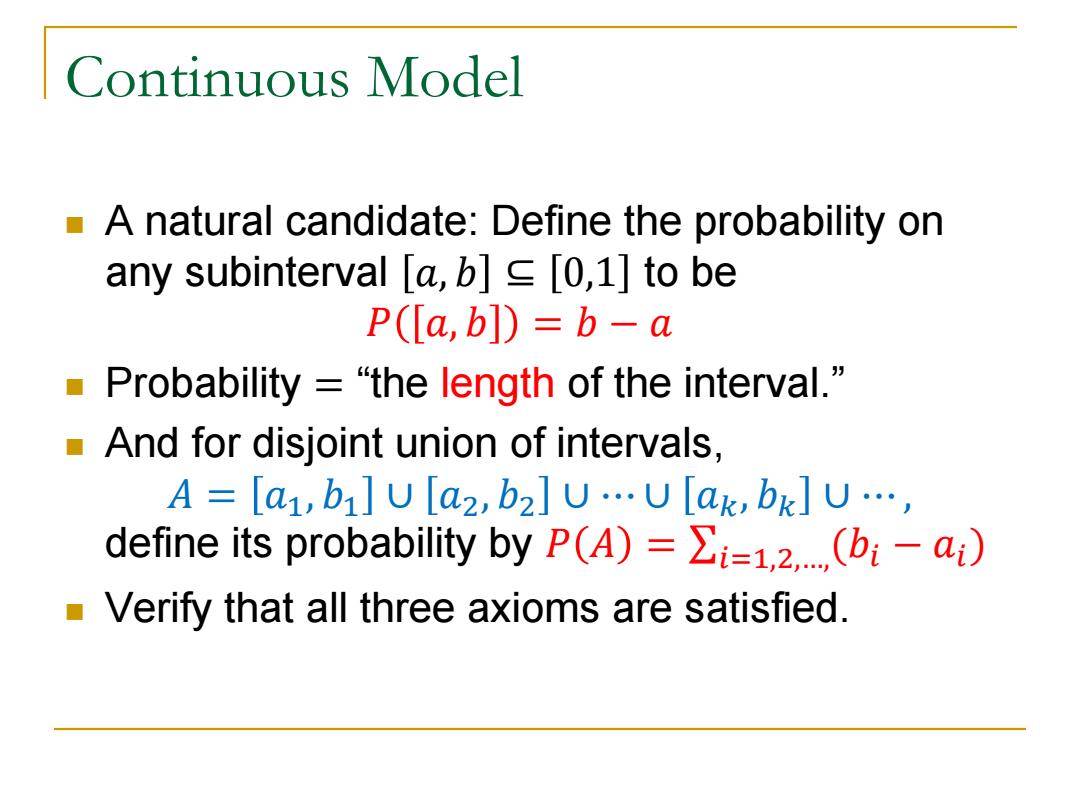
Continuous Model A natural candidate:Define the probability on any subinterval a,b0,1]to be P([a,b])=b-a Probability ="the length of the interval." And for disjoint union of intervals, A [an,bi]U [a2,b2]U...U [ak,bk]U..., define its probability by P(A)=i=1,2...(bi-ai) Verify that all three axioms are satisfied
Continuous Model A natural candidate: Define the probability on any subinterval 𝑎, 𝑏 ⊆ 0,1 to be 𝑃 𝑎, 𝑏 = 𝑏 − 𝑎 Probability = “the length of the interval.” And for disjoint union of intervals, 𝐴 = 𝑎1, 𝑏1 ∪ 𝑎2, 𝑏2 ∪ ⋯ ∪ 𝑎𝑘, 𝑏𝑘 ∪ ⋯ , define its probability by 𝑃 𝐴 = (𝑏𝑖 − 𝑎𝑖) 𝑖=1,2,…, Verify that all three axioms are satisfied

Example:Meeting Romeo and Juliet have a date. Each will arrive at the meeting place with a delay between 0 and 1 hour,with all pairs of delays being equally likely. The first to arrive will wait for 15 minutes and will leave if the other has not yet arrived. ■ Ouestion:What is the probability that they will meet?
Example: Meeting Romeo and Juliet have a date. Each will arrive at the meeting place with a delay between 0 and 1 hour, with all pairs of delays being equally likely. The first to arrive will wait for 15 minutes and will leave if the other has not yet arrived. Question: What is the probability that they will meet?
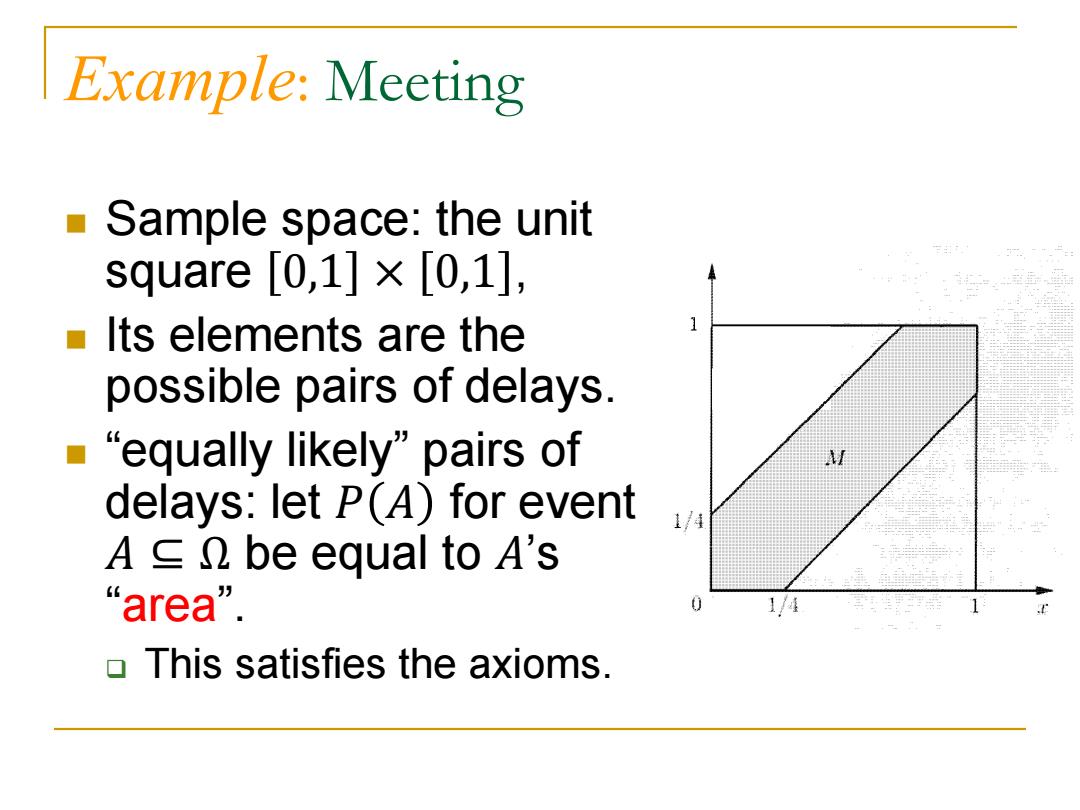
Example:Meeting Sample space:the unit square[0,1]×[0,1], Its elements are the possible pairs of delays. ■ “equally likely”pairs of delays:let P(A)for event 1/ A≤n be equal to a's “area”. 1/4 o This satisfies the axioms
Example: Meeting Sample space: the unit square 0,1 × 0,1 , Its elements are the possible pairs of delays. “equally likely” pairs of delays: let 𝑃 𝐴 for event 𝐴 ⊆ Ω be equal to 𝐴’s “area”. This satisfies the axioms