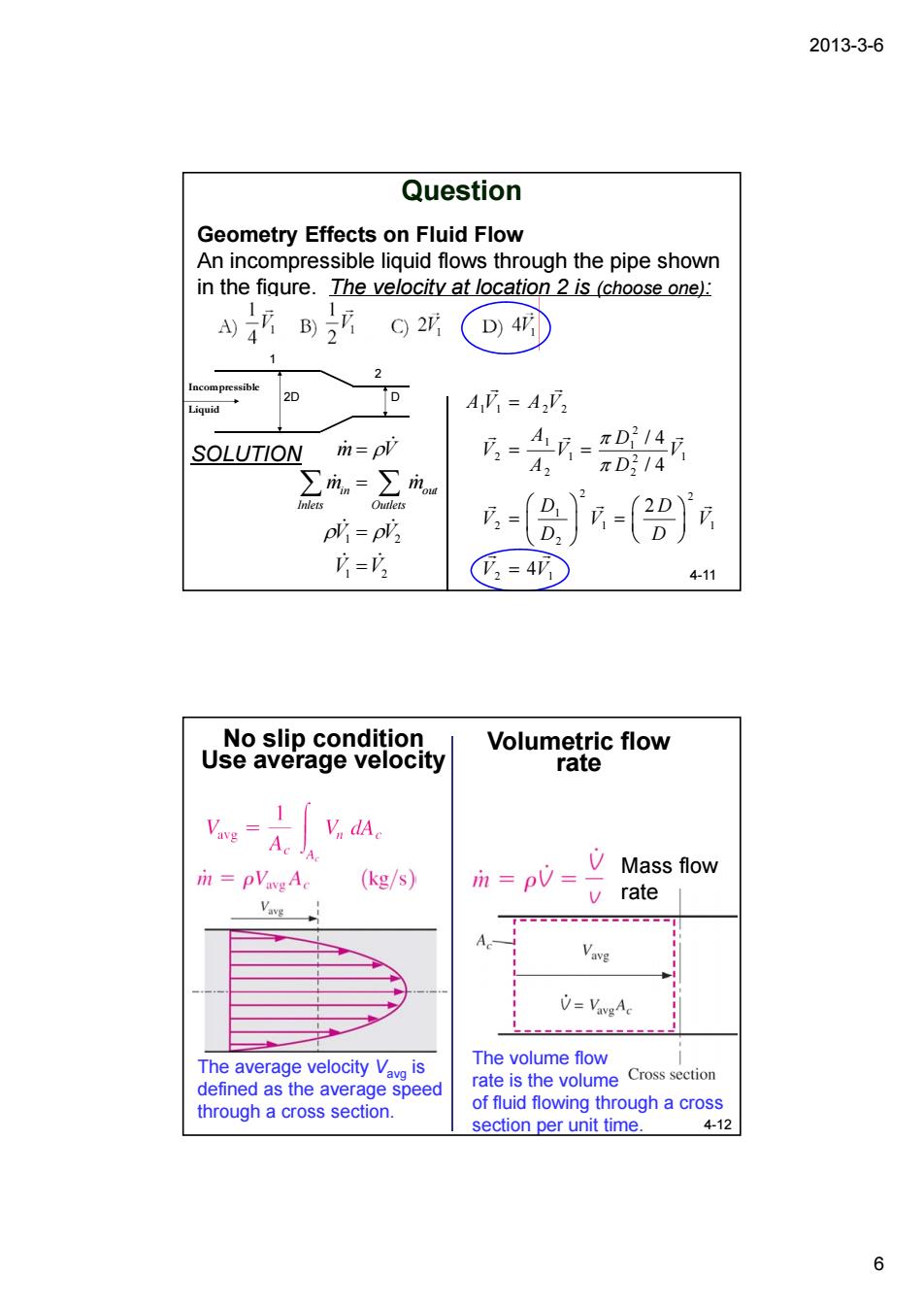
2013-3-6 Question Geometry Effects on Fluid Flow An incompressible liquid flows through the pipe shown in the fiqure.The velocity at location 2 is (choose one): 20 D iquid 1A=A,3 SOLUTION m=p护 2= pV=pV, 户= 2=4 4-11 us0oas8Pa90n8ti8iy Volumetric flow rate =A. 1 V dA. VMass flow i=pVavs Ac (kg/s) in =pV= rate The average velocity V is The volume flow defined as the average speed rate is the volume Cross section through a cross section. of fluid flowing through a cross section per u .12 6
2013-3-6 6 Question Geometry Effects on Fluid Flow An incompressible liquid flows through the pipe shown in the figure. The velocity at location 2 is (choose one): 2 1 Incompressible Liquid 2D D 1 2 1 2 in out Inlets Outlets m V m m V V V V ρ ρ ρ = = = = ∑ ∑ & & & & & & & & 11 2 2 2 1 1 21 1 2 2 2 2 2 1 21 1 2 2 1 / 4 / 4 2 4 AV A V A D VV V A D D D VVV D D V V π π = = = ⎛ ⎞ ⎛ ⎞ = = ⎜ ⎟ ⎜ ⎟ ⎝ ⎠ ⎝ ⎠ = r r r r r r r r r r 4-11 SOLUTION The average velocity Vavg is defined as the average speed through a cross section. No slip condition Use average velocity Mass flow rate Volumetric flow rate The volume flow rate is the volume of fluid flowing through a cross section per unit time. 4-12

2013-3-6 Sample problem .2 Liquid propane enters an initially empty cylindrical storage tank at a mass flow rate of 10 kg/s.Flow continues until the tank is filled with propane at 20C.9 bar.The tank is 25-m long and has a 4-m diameter.Determine the time,in minutes, to fill the tank Solution 0 dme=m- Separate variables and integrate between 0 and final time tmal -Read v for p=9 bar and T=20C 4-13 Sample problem .2 Liquid propane enters an initially empty cylindrical storage tank at a mass flow rate of 10 kg/s.Flow continues until the tank is filled with propane at 20C.9 bar.The tank is 25-m long and has a 4-m diameter.Determine the time,in minutes to fill the tank. Detailed Solution An initally-empty stereje tank ir hilled with qurd propane at a constant mars flow mtc.State data and tank dimentionr arc provided. 4-14 7
2013-3-6 7 Sample problem 4-13 = ∑ − ∑ e e i mi m dt dm & & cv 0 Solution - Separate variables and integrate between 0 and final time tfinal - Read v for p=9 bar and T = 20°C - Calculate tfinal Sample problem 4-14 Detailed Solution

2013-3-6 Solution EN长IM21W6 0DEL ANALYSIS: -4 +mcv6)=名:tf G) 4-15 Solution v66o C.99/)g EquaKmCi)jives =262wn io k3/s 4-16 8
2013-3-6 8 Solution 4-15 Solution 4-16

2013-3-6 eReview:Energy Balance for a Closed System Review:Closed Sys.Energy Balance The energy concepts introduced thus far are summarized in words as follows: change in the amount net amount of energy net amount of energy of energy contained trans rred in acros the time interval time interval Using previously defined symbols,this can be expressed as:E2-E1=Q-W Alternatively,△KE+△PE+△U=Q-W Be aware of the signs!A minus sign appears before w because energy transfer by work from the system to the surrounding is taken as positive 4-18 9
2013-3-6 9 Review: Energy Balance for a Closed System http://bcs.wiley.com/he-bcs/Books?action=index&itemId=0470918012&bcsId=6606 ►The energy concepts introduced thus far are summarized in words as follows: change in the amount of energy contained within a system during some time interval net amount of energy transferred in across the system boundary by heat transfer during the time interval net amount of energy transferred out across the system boundary by work during the time interval ►Using previously defined symbols, this can be expressed as: E2 – E1 = Q – W ►Alternatively, ΔKE + ΔPE + ΔU = Q – W Be aware of the signs! A minus sign appears before W because energy transfer by work from the system to the surrounding is taken as positive. 4-18 Review: Closed Sys. Energy Balance

2013-3-6 Review:Energy Rate Balance for CS The energy balance can be written in differential form as dE 60-8W The time rate form of the closed system energy balance is dE =Q-币 dt The rate form expressed in words is et rate contained withi transferred in the syste 4-19 Energy Rate Balance for CV, Comparina a control volume to a clos ed system: also ha ve energy that is carried in and out by the mass crossing its boundary ime rate of nge et rate at which net rate of energy of the en d ou the control volume by heat transfer by wo mass floving For one-inlet one-exit CV with one dir nsional flow =Q-巾+m,(+ g=i)-ite(ue+ +g)E4e) nergy o 10
2013-3-6 10 ►The rate form expressed in words is time rate of change of the energy contained within the system at time t net rate at which energy is being transferred in by heat transfer at time t net rate at which energy is being transferred out by work at time t ►The time rate form of the closed system energy balance is Q W dt dE & & = − 4-19 Review: Energy Rate Balance for CS ►The energy balance can be written in differential form as Energy Rate Balance for CV 4-20 ) 2 V ) ( 2 V ( 2 2 cv e e i e e i Q W mi ui gz m u gz dt dE = − + & + + − & + + & & (Eq. 4e) time rate of change of the energy contained within the control volume at time t net rate at which energy is being transferred in by heat transfer at time t net rate at which energy is being transferred out by work at time t net rate of energy transfer into the control volume accompanying mass flow ►Comparing a control volume to a closed system: we also have energy that is carried in and out by the mass crossing its boundary ►For one-inlet one-exit CV with one dimensional flow Rate of transfer of internal, kinetic and potential energy of entering/exiting streams