
善未样头导 Chapter 5 Numerical Integration
Chapter 5 Numerical Integration
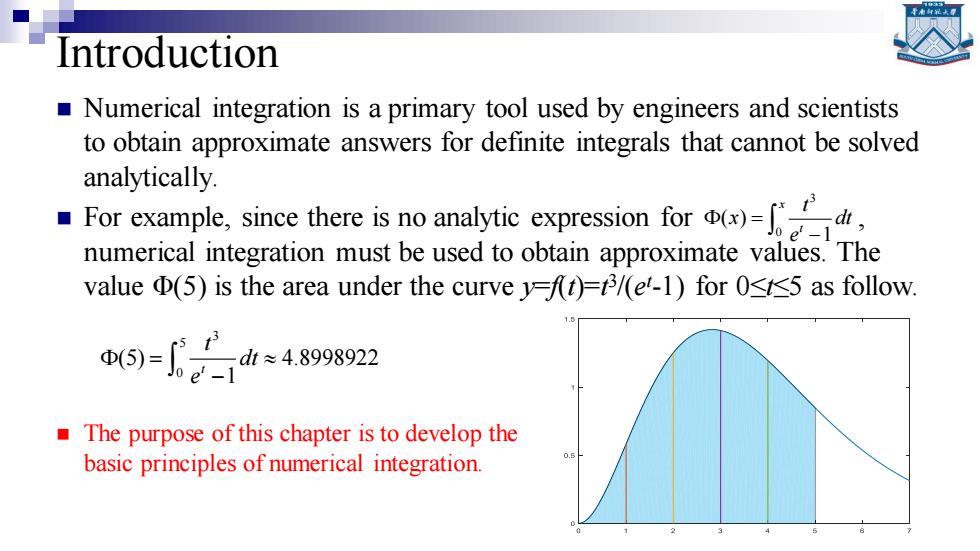
Introduction Numerical integration is a primary tool used by engineers and scientists to obtain approximate answers for definite integrals that cannot be solved analytically. For cxample.since there is no analytic exression for numerical integration must be used to obtain approximate values.The value (5)is the area under the curve )=3/(e-1)for 0t<5 as follow. 6=244s9g92 The purpose of this chapter is to develop the basic principles of numerical integration
Introduction ◼ Numerical integration is a primary tool used by engineers and scientists to obtain approximate answers for definite integrals that cannot be solved analytically. ◼ For example, since there is no analytic expression for , numerical integration must be used to obtain approximate values. The value Φ(5) is the area under the curve y=f(t)=t 3 /(e t -1) for 0≤t≤5 as follow. 3 0 ( ) 1 x t t x dt e = − 3 5 0 (5) 4.8998922 1 t t dt e = − ◼ The purpose of this chapter is to develop the basic principles of numerical integration
Some Simple Quadrature Formulas Left/Middle/Right Rectangle Rule 1=∫fx)dk≈fab-a) 1=∫fk*fab-a 1=fx)dk≈fbb-a) ■Trapezoidal Rule 1-few22Ua+f
Some Simple Quadrature Formulas ◼ Left/Middle/Right Rectangle Rule ◼ Trapezoidal Rule ( ) ( )( ) ( ) ( )( ) 2 ( ) ( )( ) b a b a b a I f x dx f a b a a b I f x dx f b a I f x dx f b b a = − + = − = − ( ) [ ( ) ( )] 2 b a b a I f x dx f a f b − = +
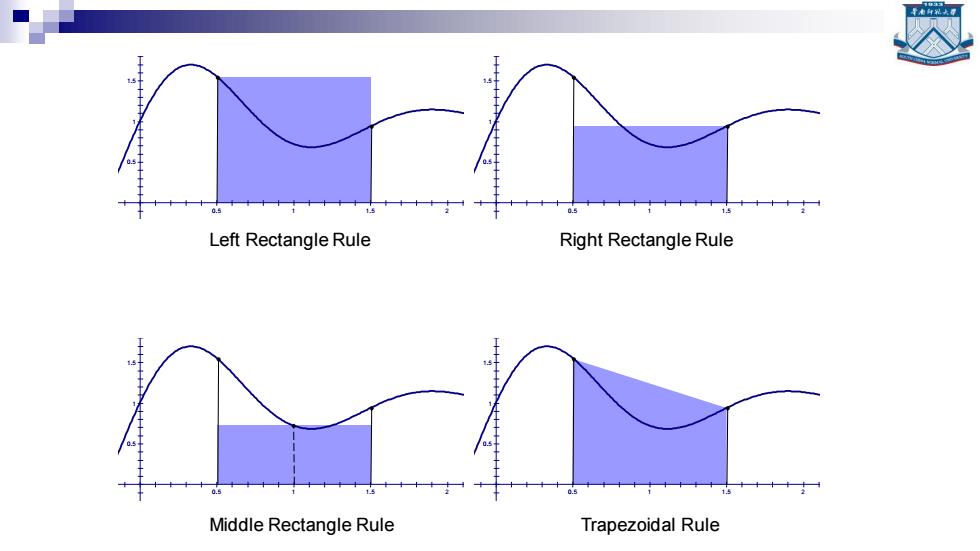
Left Rectangle Rule Right Rectangle Rule Middle Rectangle Rule Trapezoidal Rule
3.5 3 2.5 2 1.5 1 0.5 -0.5 -1 -1.5 -2 -2.5 -0.5 0.5 1 1.5 2 2.5 3 3.5 3.5 3 2.5 2 1.5 1 0.5 -0.5 -1 -1.5 -2 -2.5 -0.5 0.5 1 1.5 2 2.5 3 3.5 3.5 3 2.5 2 1.5 1 0.5 -0.5 -1 -1.5 -2 -2.5 -0.5 0.5 1 1.5 2 2.5 3 3.5 3.5 3 2.5 2 1.5 1 0.5 -0.5 -1 -1.5 -2 -2.5 -0.5 0.5 1 1.5 2 2.5 3 3.5 Left Rectangle Rule Middle Rectangle Rule Trapezoidal Rule Right Rectangle Rule
Introduction to Quadrature The goal is to approximation the definite integral offx)over the interval [a,b]by evaluatingfx)at a finite number of sample points. Def.5.1.Suppose that a=xo<x<...<xb.A formula of the form Qf1-w/(x)-w/(x)+wf(x)++wf(x) with the property that f()d+ is called a numerical integration or quadrature formula.The term E[f] is called the truncation error for integration.The values are called the quadrature nodes,and ware called the Weights
Introduction to Quadrature ◼ The goal is to approximation the definite integral of f(x) over the interval [a, b] by evaluating f(x) at a finite number of sample points. ◼ Def. 5.1. Suppose that a=x0<x1<…<xM =b. A formula of the form with the property that is called a numerical integration or quadrature formula. The term E[ f ] is called the truncation error for integration. The values are called the quadrature nodes, and are called the Weights . 0 0 1 1 0 [ ] ( ) ( ) ( ) ( ) M k k M M k Q f w f x w f x w f x w f x = = = + + + ( ) [ ] [ ] b a f x dx Q f E f = + 0 { }M k k x = 0 { }M wk k=