
Chapter 1.Solution of Nonlinear Equations f(x)=0 华南师范大学数学科学学院谢骊玲
Chapter 1. Solution of Nonlinear Equations f (x)=0 华南师范大学数学科学学院 谢骊玲

Roots of Equations Methods for solving nonlinear equations f(x)=0 Example f(x)=x2-4sin(x)=0 2 x=1.9 is a root -1 -2 -3 0.2 0.6 2.2 华南师范大学数学科学学院谢骊玲
Roots of Equations ◼ Methods for solving nonlinear equations f (x) = 0 • Example ( ) 4sin( ) 0 2 f x = x − x = -3 -2 -1 0 1 2 3 4 0.2 0.6 1 1.4 1.8 2.2 x x = 1.9 is a root f(x) 华南师范大学数学科学学院 谢骊玲

Roots of Equations ■Three Methods Fixed Point Iteration x+1=g(x) ▣Bisection f(x) ▣Newton Sequence of intervals and midpoints f(x,) X+1=X, f'(x) Ist interval 2nd y=f(x) 华南师范大学数学科学学院谢骊玲
Roots of Equations ◼ Three Methods Fixed Point Iteration Bisection Newton ( ) i 1 i x = g x + 华南师范大学数学科学学院 谢骊玲 1st interval 2nd 3rd a c x f(x) Sequence of intervals and midpoints y=f(x) '( ) ( ) 1 i i i i f x f x x + = x −
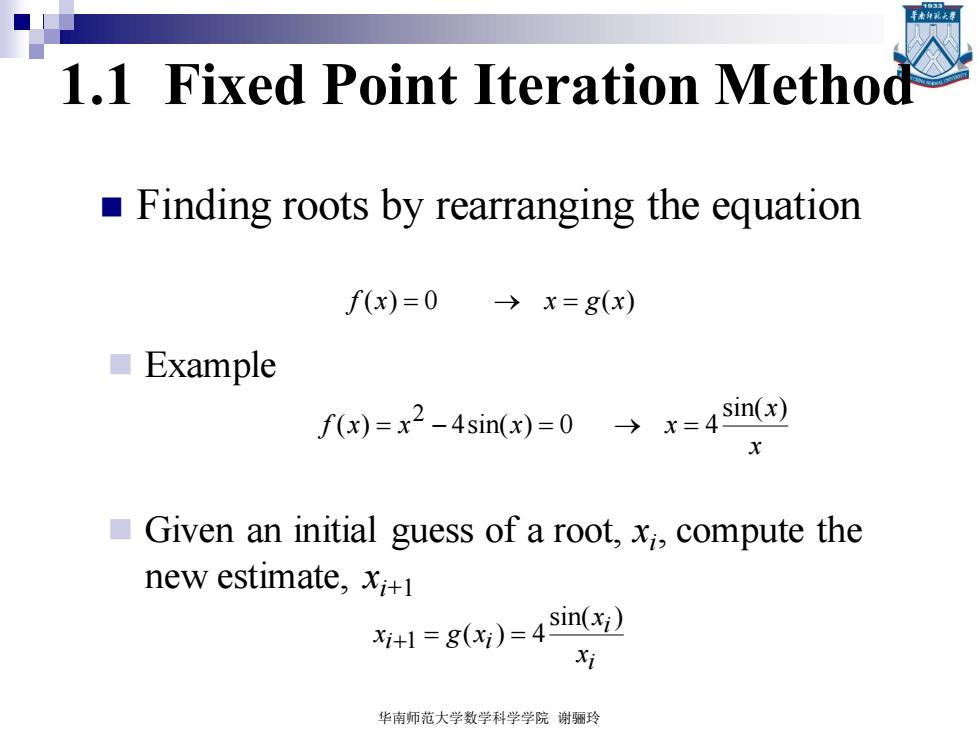
1.1 Fixed Point Iteration Method Finding roots by rearranging the equation f(x)=0 →x=g(x) ■Example f(x)=x2-4sin(x)=0x=4sin(x) Given an initial guess of a root,xi,compute the new estimate,xi+ i+1=8(x)=4sin() x 华南师范大学数学科学学院谢骊玲
1.1 Fixed Point Iteration Method ◼ Finding roots by rearranging the equation f (x) = 0 → x = g(x) x x f x x x x sin( ) ( ) 4sin( ) 0 4 2 = − = → = i i i i x x x g x sin( ) +1 = ( ) = 4 ◼ Example ◼ Given an initial guess of a root, xi , compute the new estimate, xi+1 华南师范大学数学科学学院 谢骊玲
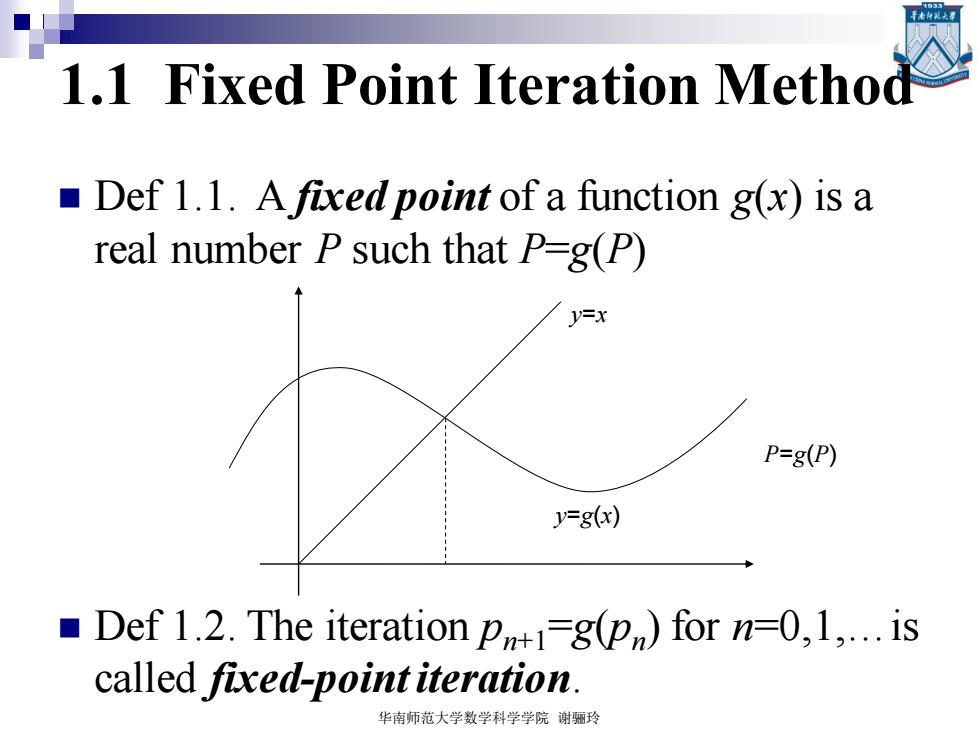
1.1 Fixed Point Iteration Method Def 1.1.A fixed point of a function g(x)is a real number P such that P-g(P) V=x P=gP) y=g(x) Def 1.2.The iteration p(p)for n-0,1,...is called fixed-point iteration. 华南师范大学数学科学学院谢骊玲
1.1 Fixed Point Iteration Method ◼ Def 1.1. A fixed point of a function g(x) is a real number P such that P=g(P) ◼ Def 1.2. The iteration pn+1 =g(pn ) for n=0,1,…is called fixed-point iteration. y=x y=g(x) P=g(P) 华南师范大学数学科学学院 谢骊玲