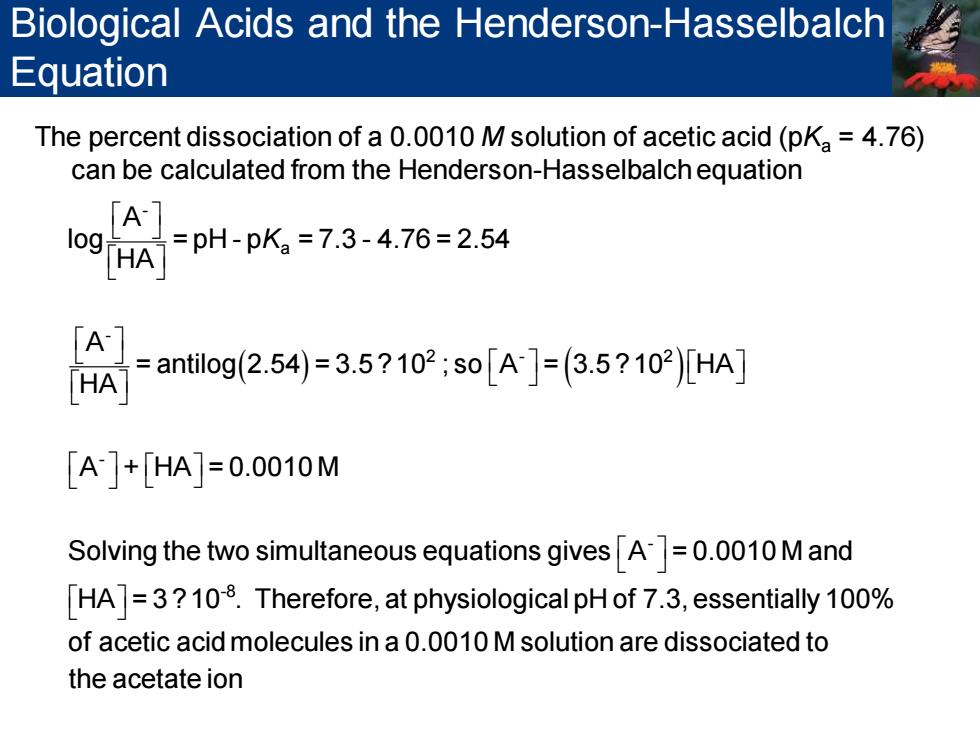
Biological Acids and the Henderson-Hasselbalch Equation The percent dissociation of a 0.0010 M solution of acetic acid(pKa=4.76) can be calculated from the Henderson-Hasselbalch equation A Iog HA- =pH-pKa=7.3-4.76=2.54 =antilog2.54)=3.5?102;so[A]=(3.5?102)[HA] [A]+[HA)]=0.0010M Solving the two simultaneous equations givesA=0.0010 M and [HA]=3?108.Therefore,at physiological pH of 7.3,essentially 100% of acetic acid molecules in a 0.0010 M solution are dissociated to the acetate ion
The percent dissociation of a 0.0010 M solution of acetic acid (pKa = 4.76) can be calculated from the Henderson-Hasselbalch equation ( ) ( ) - a - 2 - 2 - - A log = pH - p = 7.3 - 4.76 = 2.54 HA A = antilog 2.54 = 3.5 ?10 ; so A = 3.5 ? 10 HA HA A + HA = 0.0010 M Solving the two simultaneous equations gives A = 0.001 K -8 0 M and HA = 3 ?10 . Therefore, at physiological pH of 7.3, essentially 100% of acetic acid molecules in a 0.0010 M solution are dissociated to the acetate ion Biological Acids and the Henderson-Hasselbalch Equation
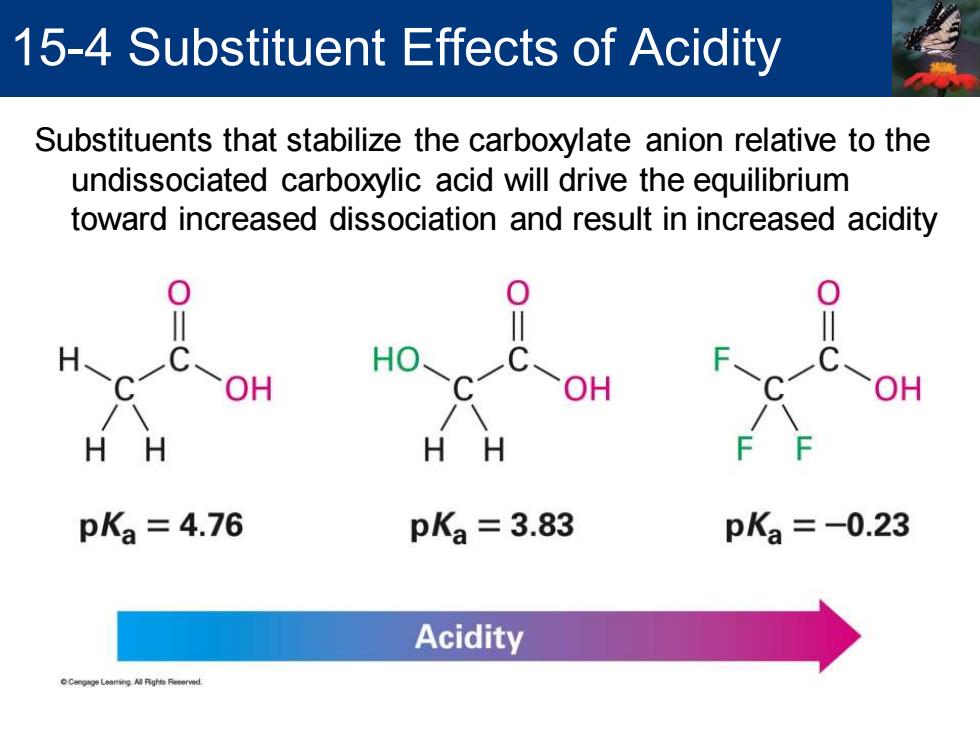
15-4 Substituent Effects of Acidity Substituents that stabilize the carboxylate anion relative to the undissociated carboxylic acid will drive the equilibrium toward increased dissociation and result in increased acidity 0 0 H-C OH HO- OH HH HH pKa=4.76 pKa=3.83 pK3=-0.23 Acidity
Substituents that stabilize the carboxylate anion relative to the undissociated carboxylic acid will drive the equilibrium toward increased dissociation and result in increased acidity 15-4 Substituent Effects of Acidity