Whole process P cuts P2 trims P3→P2→P1 PT cuts PT→P1→P choose cake 1 cake 2 choose cake 2 Cake 2 (three cases) P2 P2 P3 P3 P P2 D T P P3 P PT 11
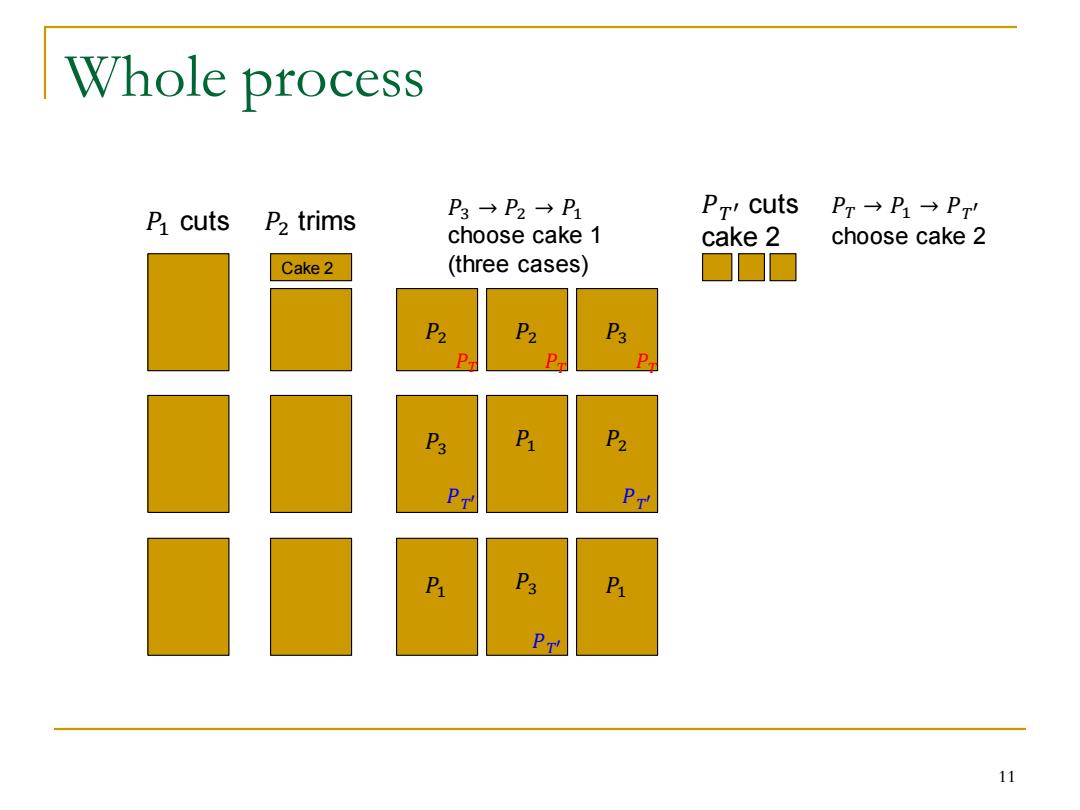
Whole process 11 𝑃1 cuts 𝑃2 trims Cake 2 𝑃3 → 𝑃2 → 𝑃1 choose cake 1 (three cases) 𝑃2 𝑃3 𝑃1 𝑃2 𝑃1 𝑃3 𝑃3 𝑃2 𝑃1 𝑃𝑇 𝑃𝑇 𝑃𝑇 𝑃𝑇 ′ 𝑃𝑇 ′ 𝑃𝑇 ′ 𝑃𝑇 → 𝑃1 → 𝑃𝑇 ′ choose cake 2 𝑃𝑇 ′ cuts cake 2

Envy-freeness The division of Cake 1 is envy-free: ■ Player 3 chooses first so he doesn't envy others. Player 2 likes the trimmed piece and another piece equally,both better than the third piece. Player 2 is guaranteed to receive one of these two pieces,thus doesn't envy others. Player 1 is indifferent judging the two untrimmed pieces and indeed receives an untrimmed piece. 12
Envy-freeness ◼ The division of Cake 1 is envy-free: ◼ Player 3 chooses first so he doesn’t envy others. ◼ Player 2 likes the trimmed piece and another piece equally, both better than the third piece. Player 2 is guaranteed to receive one of these two pieces, thus doesn’t envy others. ◼ Player 1 is indifferent judging the two untrimmed pieces and indeed receives an untrimmed piece. 12

Envy-freeness of Cake 2 Player T goes first and hence does not envy the others. Player T'is indifferent weighing the three pieces of Cake 2,so he envies no one. Player 1 does not envy T:Player 1 chooses before T' Player 1 doesn't envy T:Even if T the whole Cake 2,it's just 1/3 according to Player 1's valuation. 13
Envy-freeness of Cake 2 ◼ Player 𝑇 goes first and hence does not envy the others. ◼ Player 𝑇 ′ is indifferent weighing the three pieces of Cake 2, so he envies no one. ◼ Player 1 does not envy 𝑇 ′ : Player 1 chooses before 𝑇 ′ ◼ Player 1 doesn’t envy 𝑇: Even if T the whole Cake 2, it’s just 1/3 according to Player 1’s valuation. 13