Modeling in thermal treatment ------heating
Modeling in thermal treatment ‐‐‐‐‐‐heating

Predicting temp.distribution during hyperthermia 。Importance: -control of heating to provide sufficient thermal dose to tumor tissue but spare the surrounding healthy tissue (temp.elevation duration) -Technical difficulties of monitoring tissue temperature o Modeling: ot pc- =V.k,VT+(pc)p Wi (Ta-T)+gmb+gh t
Predicting temp. distribution during hyperthermia • Importance: – control of heating to provide sufficient thermal dose to tumor tissue but spare the surrounding healthy tissue (temp. elevation & duration) – Technical difficulties of monitoring tissue temperature • Modeling: ( ) ( ) t b b a mb h T c k T c wT T q q t

● Expression for volumetric heating(q:W/m3) - Calibration of heating apparatus in a medium with knoen physical properities. -Specific absorption rate (SAR:W/m3) g=SAR=fin(surface type,geometry,physical properties,f) =V.kWT+q pc 8t aT (SAR=g≈pcat (2)thermal model: MW analytical solution:T=fun(r,0,z,t,q) >cure fit to Tmeeusexpression for q=SAR=fin(r,,t,) medium
• Expression for volumetric heating (q:W/m3) – Calibration of heating apparatus in a medium with knoen physical properities. – Specific absorption rate (SAR: W/m3) t=0 mecus (surface type, geometry, physical properties, ) (1) (2)thermal model: analytical solution: ( , , , , ) cure fit to T expression for q SAR fun f T c kT q t T SAR q c t T fun r z t q q S AR fun r z t ( , , , , ) medium MW
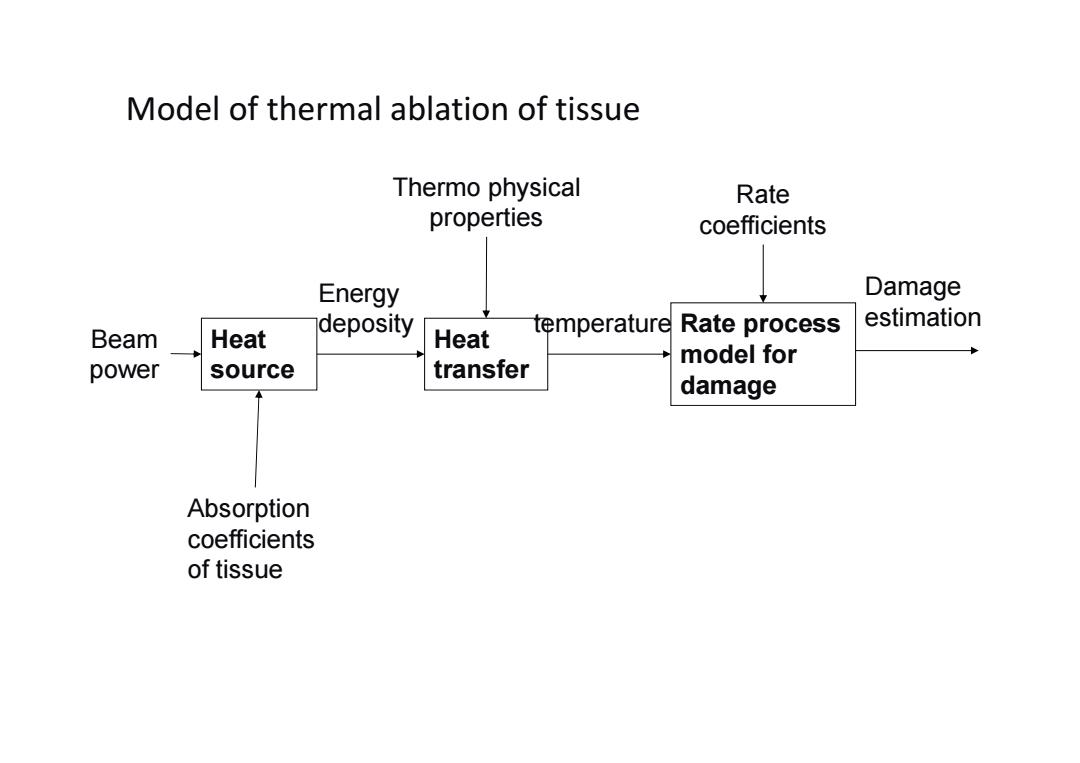
Model of thermal ablation of tissue Thermo physical Rate properties coefficients Energy Damage estimation Beam Heat deposity Heat temperature Rate process model for power source transfer damage Absorption coefficients of tissue
Model of thermal ablation of tissue Damage Rate process estimation model for q rzt n (, ,) damage Heat source Heat transfer Beam power Absorption coefficients of tissue Energy deposity Thermo physical properties temperature Rate coefficients T rzt (, ,) (, ) r z
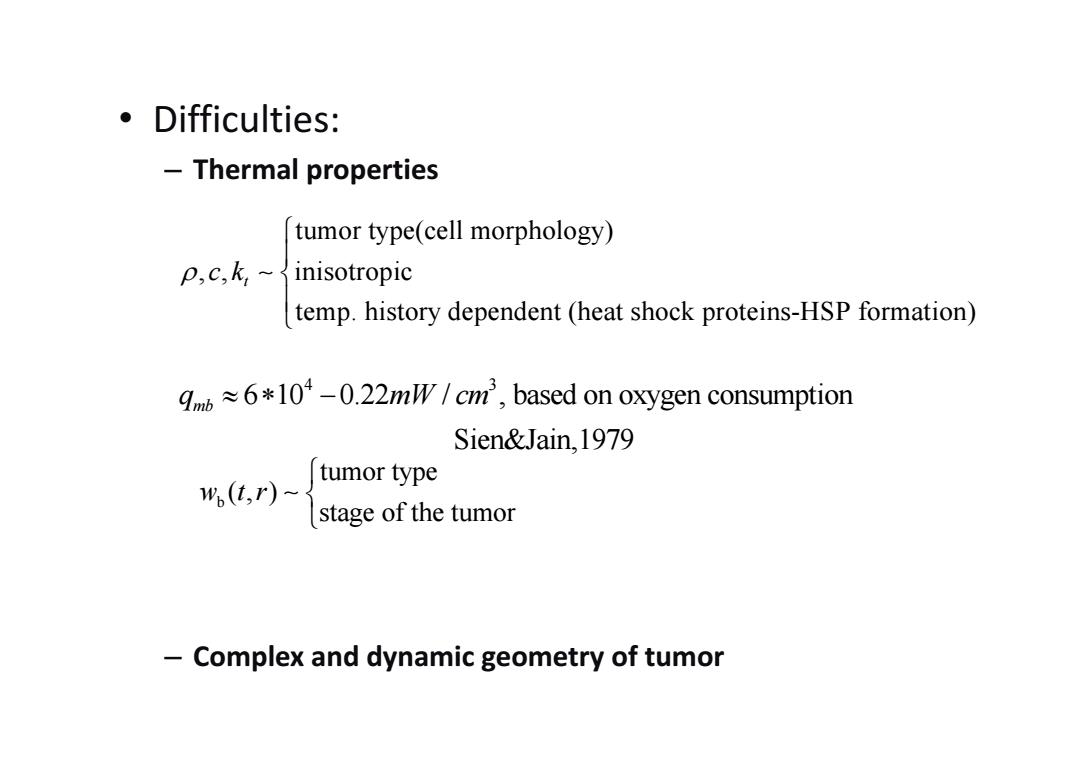
。Difficulties: Thermal properties tumor type(cell morphology) P,c,k,~inisotropic temp.history dependent (heat shock proteins-HSP formation) 6*10*-0.22mW/cm',based on oxygen consumption Sien&Jain,1979 w(t.r)-s tumor type stage of the tumor Complex and dynamic geometry of tumor
• Difficulties: – Thermal properties – Complex and dynamic geometry of tumor b tumor type (, ) stage of the tumor w tr tumor type(cell morphology) , , inisotropic temp. history dependent (heat shock proteins-HSP formation) t c k 4 3 6 10 0.22 / , based on oxygen consumption Sien&Jain,1979 mb q mW cm