
Total Variation (continued) SST=(X1-X2+(X2-X)2+…+(Xm-X2 Response,X Group 1 Group 2 Group 3
Total Variation Group 1 Group 2 Group 3 Response, X X 2 cn 2 12 2 11 SST ( X X ) ( X X ) ... ( X X ) c (continued)

Among-Group Variation SST=SSA+ SSW SSA=Σn,(X1-XP j=1 Where: SSA=Sum of squares among groups c number of groups or populations n;=sample size from group j(may be not equal for each-group) X sample mean from group j X=grand mean(mean of all data values)
Among-Group Variation Where: SSA = Sum of squares among groups c = number of groups or populations nj = sample size from group j (may be not equal for each group) Xj = sample mean from group j X = grand mean (mean of all data values) 2 j c j 1 j SSA n ( X X ) SST = SSA + SSW
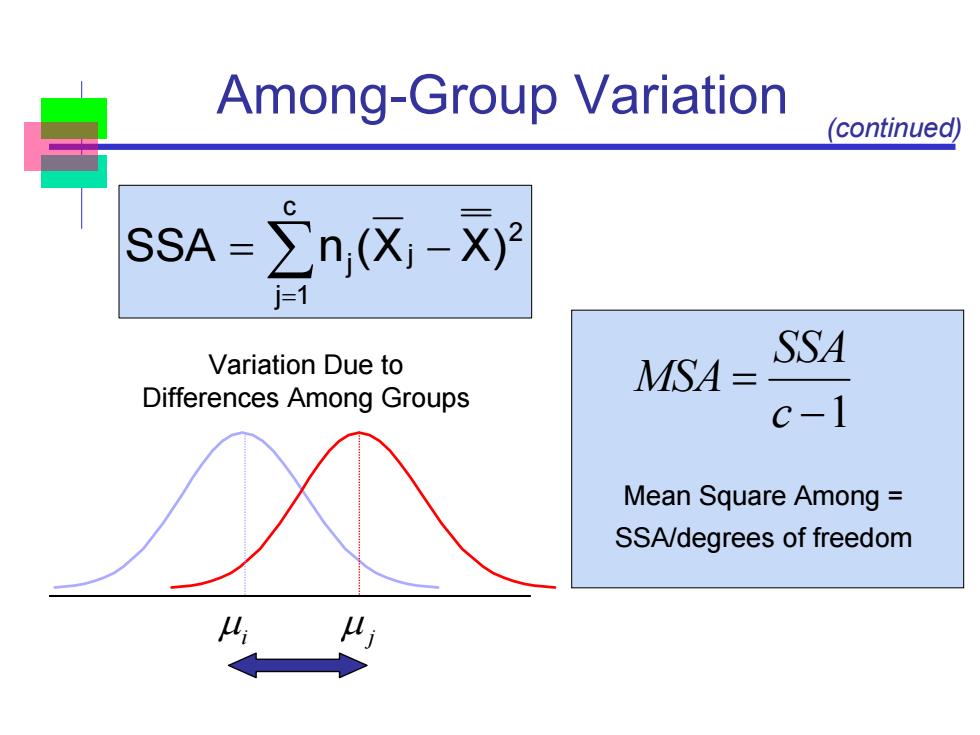
Among-Group Variation (continued) SSA=∑n,(X-X2 i=1 Variation Due to SSA MSA= Differences Among Groups c-1 Mean Square Among SSA/degrees of freedom
Among-Group Variation Variation Due to Differences Among Groups i j 2 j c j 1 j SSA n ( X X ) 1 c SSA MSA Mean Square Among = SSA/degrees of freedom (continued)
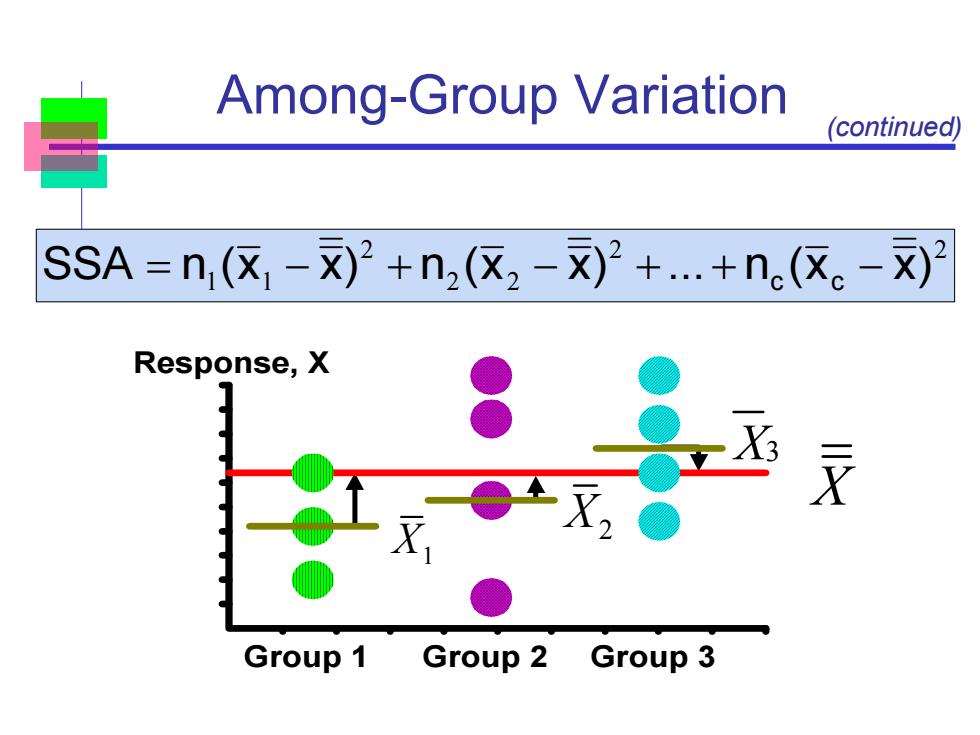
Among-Group Variation (continued) SSA=n(区1-)2+n2(风2-)2+.+n.(区。-x)2 Response,X 8 Group 1 Group 2 Group 3
Among-Group Variation Group 1 Group 2 Group 3 Response, X X X1 X2 X3 2 2 2 2 2 1 1 SSA n ( x x ) n ( x x ) ... n ( x x ) c c (continued)