
One-Factor ANOVA (continued) H0:μ1=八2=μ3=…=c H,:Not all u are the same At least one mean is different: The Null Hypothesis is NOT true (Treatment Effect is present) 1=2≠3 μ1≠2≠3
One-Factor ANOVA At least one mean is different: The Null Hypothesis is NOT true (Treatment Effect is present) 0 μ1 μ 2 μ 3 μ c H : H1 : Not all μi are the same μ1 μ 2 μ 3 μ1 μ 2 μ 3 or (continued)
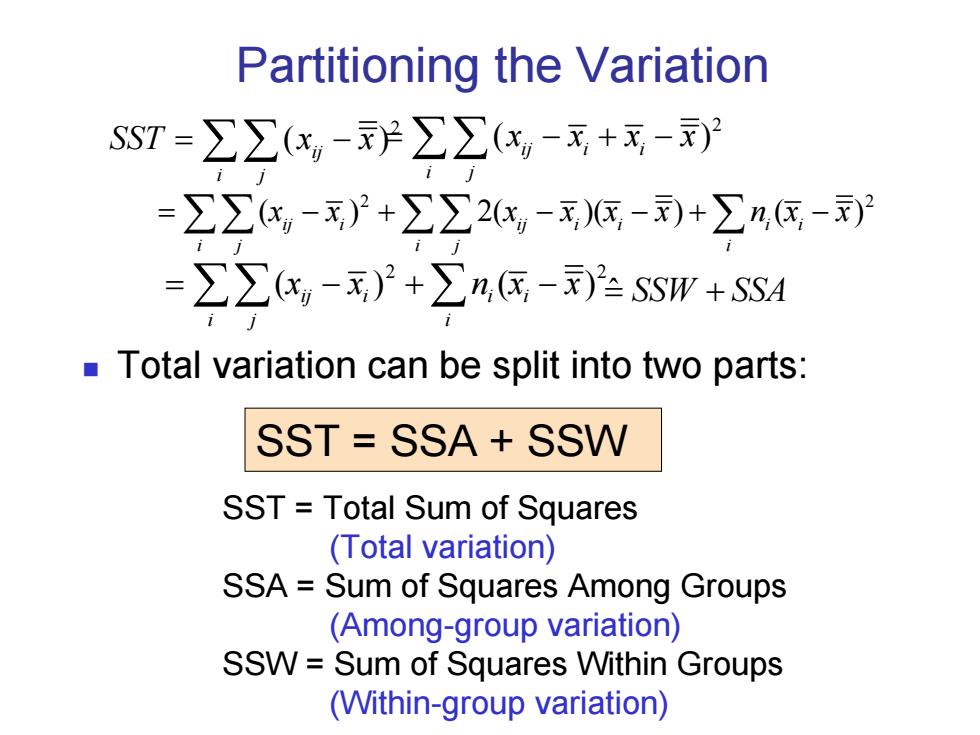
Partitioning the Variation SS7=∑∑(x,-}∑∑(,-可+-) =∑∑x,-)+∑∑2化,-元区-元+∑n,低-) =∑∑y-x)》+∑n,民-)SSW+SSA Total variation can be split into two parts: SST SSA+SSW SST Total Sum of Squares (Total variation) SSA Sum of Squares Among Groups (Among-group variation) SSW Sum of Squares Within Groups (Within-group variation)
Partitioning the Variation Total variation can be split into two parts: SST = Total Sum of Squares (Total variation) SSA = Sum of Squares Among Groups (Among-group variation) SSW = Sum of Squares Within Groups (Within-group variation) SST = SSA + SSW 2 ( ) ij i j SST x x 2 2 ( ) 2( )( ) ( ) ij i ij i i i i i j i j i x x x x x x nx x 2 2 ( ) () ij i i i i j i x x nx x 2 ( ) ij i i i j x xxx ˆ SSASSW

Partitioning the variation (continued) SST=SSA SSW Total Variation the aggregate dispersion of the individual data values across the various factor levels(SST) Among-Group Variation dispersion between the factor sample means(SSA) Within-Group Variation dispersion that exists among the data values within a particular factor level (SSW)
Partitioning the Variation Total Variation = the aggregate dispersion of the individual data values across the various factor levels (SST) Within-Group Variation = dispersion that exists among the data values within a particular factor level (SSW) Among-Group Variation = dispersion between the factor sample means (SSA) SST = SSA + SSW (continued)
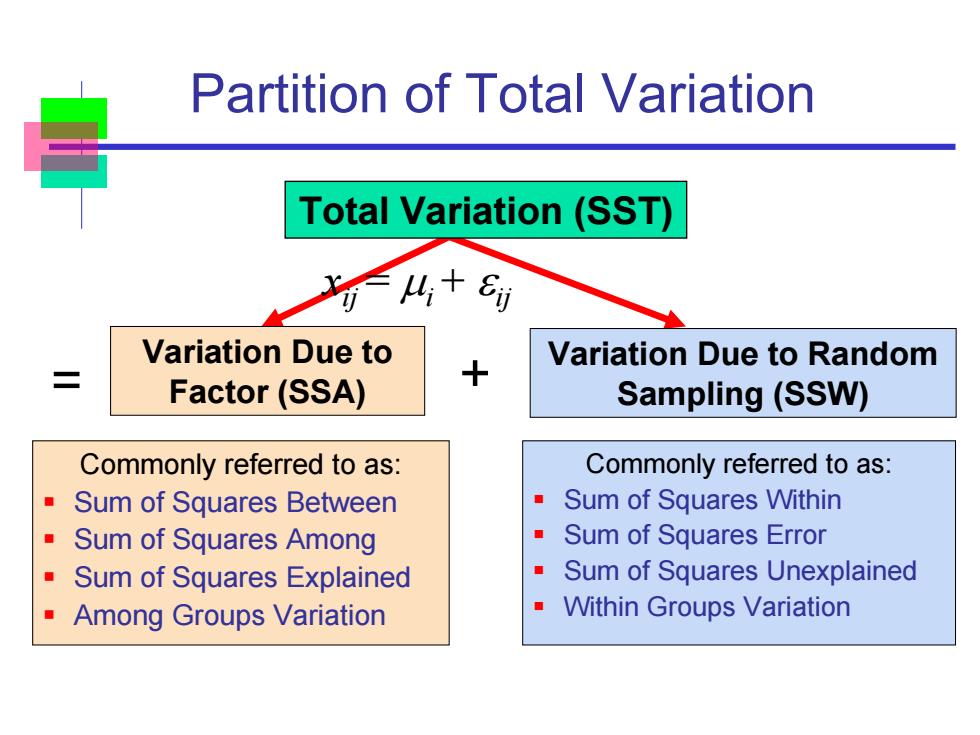
Partition of Total Variation Total Variation (SST) 4,+ Variation Due to Variation Due to Random 十 Factor(SSA) Sampling (SSW) Commonly referred to as: Commonly referred to as: Sum of Squares Between Sum of Squares Within Sum of Squares Among Sum of Squares Error Sum of Squares Explained Sum of Squares Unexplained Among Groups Variation Within Groups Variation
Partition of Total Variation Variation Due to Factor (SSA) Variation Due to Random Sampling (SSW) Total Variation (SST) Commonly referred to as: Sum of Squares Within Sum of Squares Error Sum of Squares Unexplained Within Groups Variation Commonly referred to as: Sum of Squares Between Sum of Squares Among Sum of Squares Explained Among Groups Variation = + xij = i + ij

Total Sum of Squares SST-SSA SSW sST=2∑(X,-X)2 Where: i=1i=1 SST Total sum of squares c number of groups (levels or treatments) n;number of observations in group j Xi=ith observation from group j X=grand mean(mean of all data values)
Total Sum of Squares c j 1 n i 1 2 ij j SST ( X X ) Where: SST = Total sum of squares c = number of groups (levels or treatments) nj = number of observations in group j Xij = ith observation from group j X = grand mean (mean of all data values) SST = SSA + SSW