
If &O is a Exact Differential Expression au +p 0 ot 2U2 aU arav avar )+P at 6 Q is not an exact differential expression, So Q is not a state function
If δQ is a Exact Differential Expression 2 2 ()() ( ) v T v U U P T V V T U UP TV VT T ∂ ∂ + ∂ ∂ = ∂ ∂ ∂∂∂ = + ∂∂ ∂∂ ∂ 0 ? ()() v T U U P T V V T ∂ ∂ + ∂ ∂ ≠ ∂ ∂ δQ is not an exact differential expression, So Q is not a state function ? ≠ ?
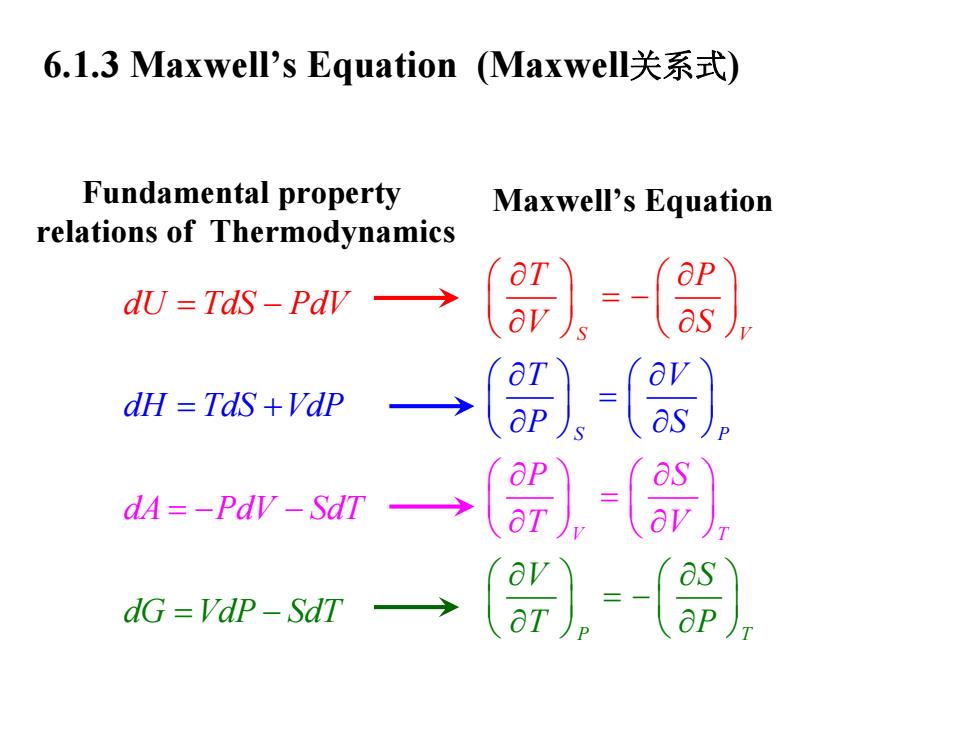
6.1.3 Maxwell's Equation(Maxwell关系式) Fundamental property Maxwell's Equation relations of Thermodynamics dU=Tds-Pdr→ dH TdS +Vdp dA=-PdV-SdT dG=Vap-SdT
6.1.3 Maxwell’s Equation (Maxwell关系式 ) Maxwell’s Equation S P T V T S P V P S V S T P T V P S V P V S T T ∂ ∂ ⎛ ⎞ ⎛⎞ ∂ ∂ = − ⎛⎞⎛ ⎞ ⎜⎟⎜ ⎟ = ⎝⎠⎝ ∂ ∂ ⎜ ⎟ ⎜⎟ ⎝ ⎠ ∂ ∂ ⎛ ⎞ ⎛⎞ ∂ ∂ ⎜ ⎟ ⎜⎟ = − ⎝ ∂ ∂ ⎛ ⎞⎛ ⎞ ∂ ∂ ⎜ ⎟⎜ ⎟ = ∂ ∂ ⎠ ⎝ ⎝ ⎠ ⎝ ⎠ ⎠ ⎠ ⎠ ⎝ dA dU dH TdS Vd dG VdP P S d dT V TdS PdV SdT P = = − − = − = + − Fundamental property relations of Thermodynamics
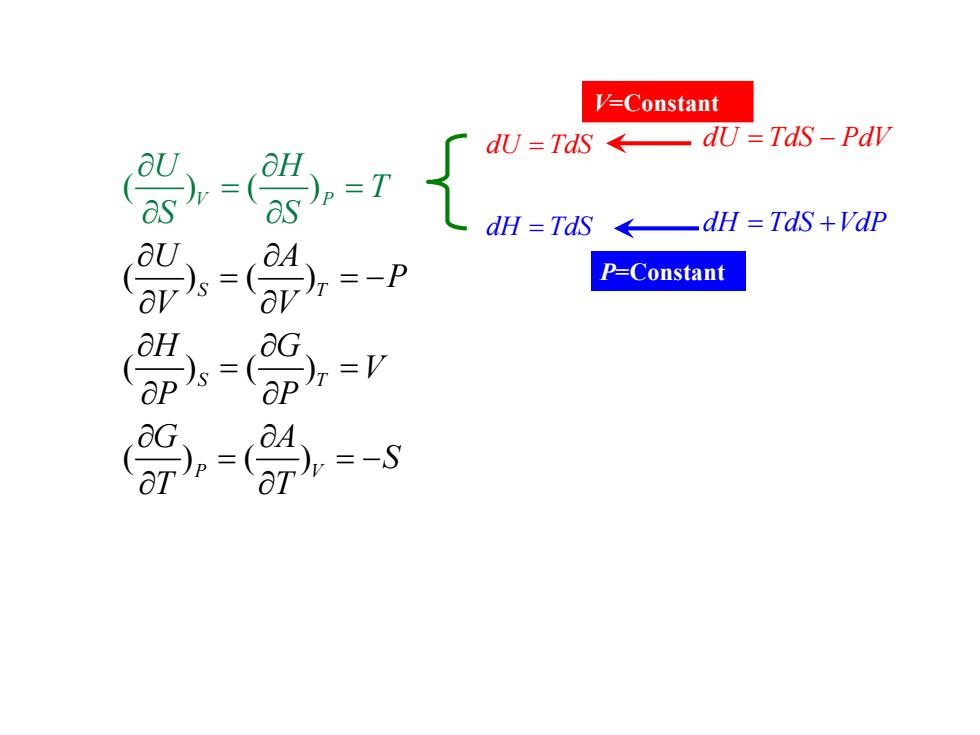
V=Constant dU=TdS←-dU=TdS-Pdw dH TdS dH TdS+Vdp -P P=Constant
()() ()() ()( ()() ) S T S T P V V P U A P V V H G V P P G A S T H T T U S S ∂ ∂ = =− ∂ ∂ ∂ ∂ = = ∂ ∂ ∂ ∂ = =− ∂ ∂ ∂ ∂ = = ∂ ∂ d d H d U TdS T S = = d d H U TdS PdV TdS VdP = = + − P=Constant V=Constant

Example 试计算在0.01013MPa下,液态汞由275K恒容加热到 277K时产生的压力 解:思路 aP (avlot)p aT (av/aP)T Volume expansivity B=108V )p=0.00018K- V aT 10那,=000385K V ap ep) 0.00018 0.0000385 =4.675 MPa/K Isothermal compressibility 4.675×(277-275)=9.35MPa
Example 试计算在0.01013MPa下,液态汞由275K恒容加热到 277K时产生的压力 P T T ∂ ×Δ ∂ ( )V (/) (/) P T P V T T VP ∂ ∂ ∂ = − ∂ ∂∂ ( )V 1 0.00018 V K T β ∂ − = ∂ P 1 =( ) V 1 0.0000385 V K P∂ − = ∂ T 1 k=- ( ) V 0.00018 4.675 / 0.0000385 P MPa K T ∂ = = ∂ ( )V 4.675 (277 275) 9.35 ×−= MPa 解:思路 Volume expansivity Isothermal compressibility

满瓶液氯压力与温度的关系 温度 0 5 10 15 20 25 30 35 ℃ 瓶压 2.7 71 131 184 240 275 325 356 kg/cm2
满瓶液氯压力与温度的关系 温度 ℃ 0 5 10 15 20 25 30 35 瓶压 kg/cm 2 2.7 71 131 184 240 275 325 356