
T Vein Artery Ta T CV qm-Metabolic heat generation do-Volumetric heat rate due to blood T:-average tissue temp.within the C.V
Artery C.V. Vein Tt Tv qm- Metabolic heat generation qb – Volumetric heat rate due to blood Tt - average tissue temp. within the C.V. Ta

4.No change in blood temp.until it reaches the capillaries where the blood-tissue thermal equilibrium takes place. 5.Blood leaves the C.V.and enters the vein at T. 96=w6P6C6(T。-T,) Wb ----blood perfusion rate, Volumetric blood flow rate per unit tissue volume [m3/s/m3] a=Tk-wm】
4. No change in blood temp. until it reaches the capillaries where the blood-tissue thermal equilibrium takes place. 5. Blood leaves the C.V. and enters the vein at Tv. ( ) b b bb a v q w cT T = − ρ w b ----blood perfusion rate, Volumetric blood flow rate per unit tissue volume [m 3/s/m 3] 3 3 3 3 / [ ][ ][ ][ ] [ / ] b m s kg J q K wm m m kg K = = ⋅
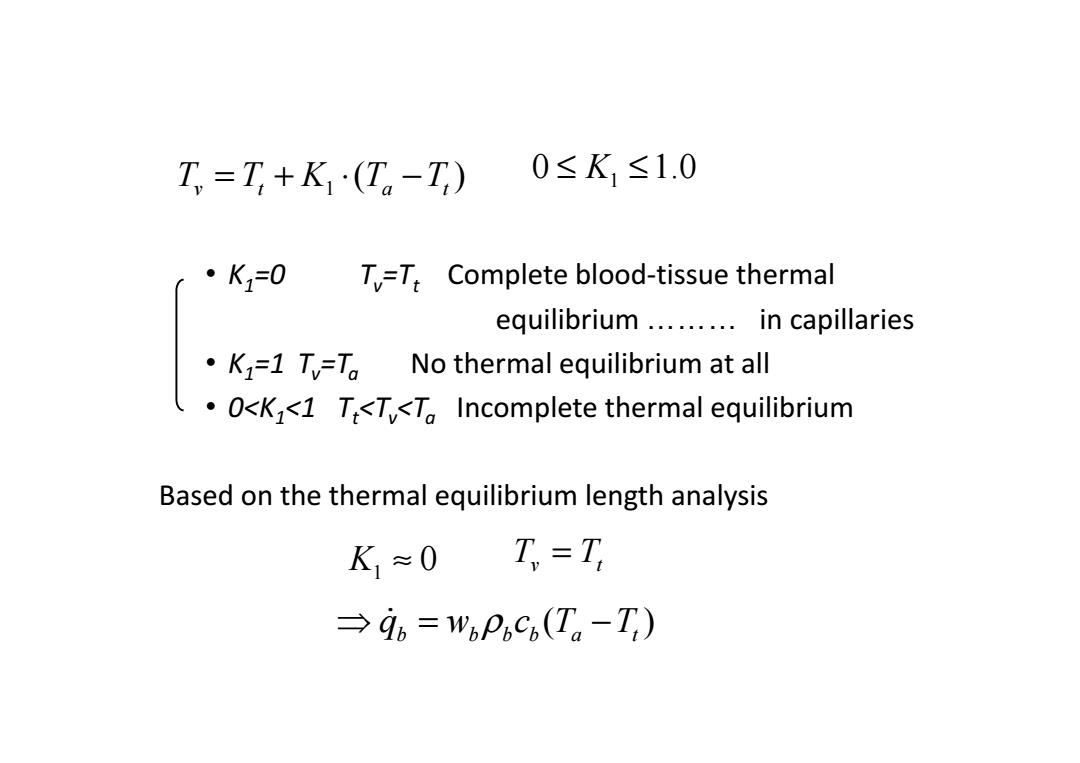
T=T,+K·(Ta-T) 0≤K,≤1.0 ·K1=0 T=T:Complete blood-tissue thermal equilibrium ........in capillaries ·K=1T,=T。No thermal equilibrium at all 、·O<K,<1T<T,<T。Incomplete thermal equilibrium Based on the thermal equilibrium length analysis K1≈0 T,=T, →96=w6PbC6(T。-T)
• K1=0 Tv=Tt Complete blood-tissue thermal equilibrium ……… in capillaries • K1=1 Tv=Ta No thermal equilibrium at all • 0<K1<1 Tt<Tv<Ta Incomplete thermal equilibrium Based on the thermal equilibrium length analysis 1 K ≈ 0 ( ) b b bb a t q w cT T = − ρ 1 ( ) T TKTT v t at =+ ⋅ − 1 0 1.0 ≤ ≤ K Tv = Tt

Ta-Cb-PbYb > 2 T,C,P1,06
r z t t t b T ,c ,ρ ,ω a b b b T ,c ,ρ ,v h Troom , ∞
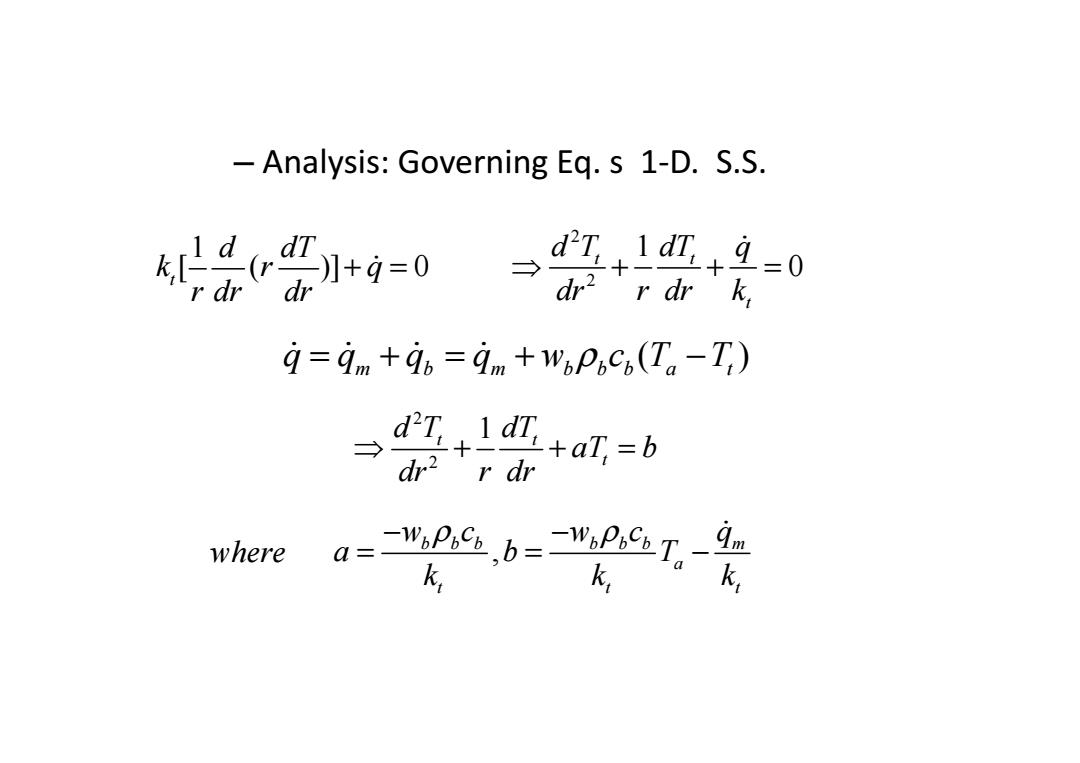
Analysis:Governing Eq.s 1-D.S.S. 41+g=0 dPT+1d0+9=0 dr2r dr k 9=9m+96=9m+w6P,Cb(Ta-T) dg+ld亚+a,=b dr2'r dr where a=06-e是 9m k
– Analysis: Governing Eq. s 1-D. S.S. 1[ ( )] 0 t d dT kr q r dr dr + = 2 2 t t 1 t d T dT aT b dr r dr + += 2 2 1 0 t t t d T dT q dr r dr k + += where ( ) m b m b bb a t q q q q w cT T = += + − ρ , b bb b bb m a t tt wc wc q a bT k kk − − ρ ρ ==−