
1.2 The birth of guantum mechanics 11 the system is equivalent to two infinite sets of independent harmoni oscillators.By taking the cavity to be of cubic form,each side of length L.the wave numbers k are found to have the discrete values (see Supplement 20.2).Since this Hamiltonian has the standard form 15).we can apply the equipartition law to compute the total energy the answer is simply U=fkT:f(=the number of degrees of freedom)=oo. and thus n otherords,the energy of electrom ordingt xwell's cory.In er to se the ten of the cavity by one der e unt of twould be ne These conclu are clearly absurd an are in clear con trast to the experiencethink of an oven at home which eats up an infinite amount of electricity to warm up by just one degree!Empirically the energy of black-body radiation is known to be given,per unit volume,by U=aT;=7.64×10-15 ergcm3K4 (1.6 result known as the Stefan-Boltzmann law. This is the oblem of black-bodu radiation for this disastrous Tee网 are" energ freedom are ively active at any given temperature Not all is lost,however.Let us consider the contribution from the modes having a restricted range of frequencies,(),instead of computing the total energy -). directly.Asyo=光,itfollows that 血s2Ldw Each oscillation mode corresponds to the set of positive integers n= (n,n,n):the number of modes having frequencies between and dv is given by N创w=2g4xm的=8P

12 Introduction ao The to eqn trequency u(v)dv=kTN(v)dv= 8k灯2dw The n (1.7) replac 3 10u) This is the classical formula for(the Rayleigh-Jeans formula).Ex. 05050 fR-J periments show that: is ma 1200C oscilla egn (I 900c 60c (2)The frequency range in which the Rayleigh-Jeans formula is valid Pla of qu 0 5 10.1510 widens towards the higher frequencies as T increases (Figure 1.5). mean esis c (3)For any fixed frequency the classical formula is good atsuf ently high T but fails at lower T:we see cleariy that the problem is T=1200 Cis plotted for comparison. of the same nature as the one which plagued the classical theory of th of specific heats.As the temperature is lowered,more degrees of freedom get frozen and cease to get the full share of energy. The first step towards the solution of the problem was taken by Wien in 1893,who observed that the experimental data satisfy the"displace- ment law”(or "scaling law") (1.8) whe with a function Fto be determined empirically. Namely,if u(v)is den known at a temperature,by (1.8)we get the distribution at any other temperature.Formula(1.8)is also consistent with the Stefan-Boltzman law.In fact the displacement law(1.8)can be theoretically derived from thermodynamics Tomonaga(1968). Fig.1.6The Even if Wien was not able to determine the functional form of F(), he was able to find the empirical behavior of F(),valid at large Th thank nce Tear F(z)kBe-=;B=const.. or,by substitution in (1.8),the formula ("Wien's formula") (1.9) a where h=k=6.626×10-27crg. (1.10) versea temperature 2.725Kto We then had the classical formula (1.7)good at low frequencies.and th Wien's for mula (19)valid at high freo encies.It was Planck in 1900 (cCMB).An the TE has beer ture Auctuatio at the tme of matter -d, (.1) e1.6)

1.2 The birth of quantum mechanics 13 The reader should verify that this formula reduces to eqn(1.7)or (1),respectively,in the region hu/or h/ moral of the story is that the Planck distribution follows if the ement (E=kT→ekr-1 (1.12) ismade in the quipartition lawfor the y share of the the meaning of Planck's fundamental contribution to phys cs,which signaled the birth of quantum physics,was the realization t meant the "energy quantum".Let us briefly review how the hypoth esis of the energy quantum gives the energy share(1.12)instead of the equipartition law.For each frequency and for each of the polarizations, the Hamiltonian of the electromagnetic fluctuations is a simple oscillator y of the form H=ag2+bp2 In polar coordinates E0=tan-1 y/r,where x=vag:y= bp.the mean energy is (=-oeN=aBe where the trivial angular integration has been cancelled between the denominator and numerator.If we perform the integration over Ethe result is,of course,the equipartition law,T. Let us instead assume that the energy comes in (for some reason) multiples of a unit quantum,, En =ne, n=0,1,2,3. The integration must then be replaced by a summation aB→eL (1.13) and we get (份=-TgW =e∑er=1- that is If the energy unit is chosen as e=hy. sgivea pred phat forded (oom.l》to get Planck色omt一 1。: The meaning thu 9u4 ization of magnetic energy:light of wavelength=c/is made up of quanta each
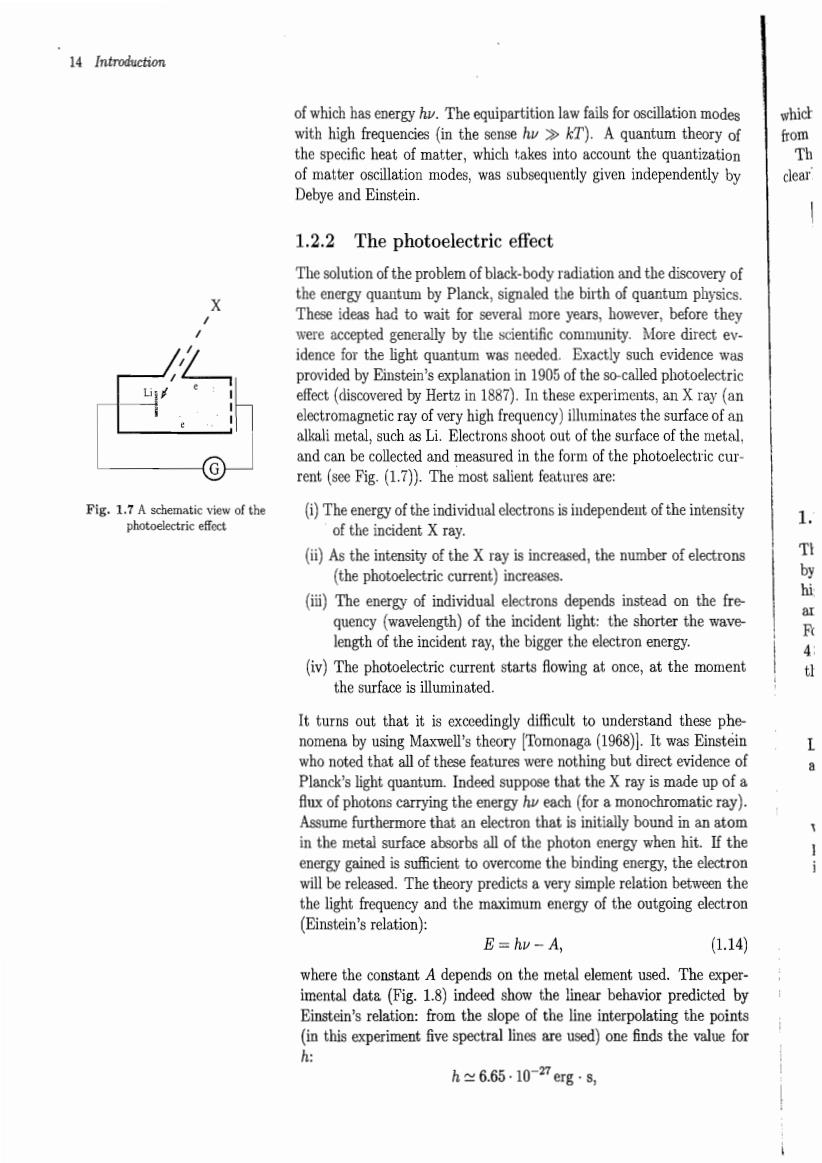
14 Introduction of which haseergyThequipartition w fails for oscillation modes which with high frequencies (in the sensehT).A quantum theory of from the specific heat of matter,which takes into account the quantization of matter oscillation modes,was subsequently given independently by clear Debye and Einstein. 1.2.2 The photoelectric effect The solution of the problem of black-body radiation and the discovery of the energy quantum by Planck,signaled the birth of quantum physics. These ideas had to wait for several more years,however,before they were accepted generally by the scientific community.More direct ev. idence for the light quantum was needed.Exactly such evidence was provided by Einstein's explanation in 1905 of the so-called photoelectric effect (discovered by Hertz in 1887).In these experiments.an X ray (an electroma tic ray of very high f rons shoe ency)iluminates the and can be and me ed in the f the rent (see Fig.(17)). .The most e features ar (i)The energy of the individual electronsisindependet of the intesity of the incident X rav. number of electrons by hi (iii)The energy of individual electrons depends instead on the fre r Raeeoe (iv)The photoelectric current starts flowing at once,at the moment th the surface is illuminated. It turns out that it is exceedingly difficult to understand these phe nomena by using Maxwell's theory Tomonaga (1968).It was Einstein who noted that all of these features were nothing but direct evidence of Planck's light quantum.Indeed sup e that the X ray is made up of a x of photon th hy each (for furthe in the m that is initially bo nd in an a s all of the If th come the bind g e the ased.The theory predictsa very simpl th the light frequency and the maximum energy of the outgoing electron Einstein's relation): E=hv-A, (1.140 where the constant A depends on the metal element used.The exper imental data (Fig.1.8)indeed show the linear behavior predicted by Einstein's relation:from the slope of the line interpolating the points (thexpermet five spectaare ed)one inds the h≈6.65107erg8

1.2 The birth of quantum mechanics 15 which is ingoo ement with the value found by Planck,eqn(1.10), from the analysis gfblack.bodyradiation ()isanother phenomen whichshows Fig.1.8 Millikan's experimental re 1.2.3 Bohr's atomic model by Bohr in 1913.At the tim e of Bohr wn that agas heated to ctrum.(Fo ample,the sodum lamp make es light with a For hydrogen,a sere dthe wave 6562.8 4861.3,4340.5,4101.7,(A).Balmer had di these lines could be summarized by an empirical formula, A=-4b=36456A n=3,4,5, 2,n)=T2(nz)-T(m) (1.15 terizing a series.In in a very simple form, R R (1.16) ) where Risa universal constant (ie,independent of the element), R=109678cm1, ts depe (called the Rydrg)o are some cotan nding on the element.F ra o ng,n1)=v(ng,n2)+(n2,n1)