
16 Introduction (Ritz's combination rule).It remained to explain Rydberg's formulas 1.2.4 Bohr's idea was that the energy of the bound electrons could take onlysome qantized values,just like the energy of the electromagneti fields in a cavity.More precisely,Bohr formulated the following et 0tanL山 (Planc .(1)The possible values of the bound electrons in an atom are Cinste ation quantized.EE (1925 cal im .(2)The atom emits light when an electron makes a transition from man one state(n)to another(m);the light emitted or absorbed in such Bo transitions has a frequency such that hw=E。-Em 117) it is equal to the energy of the photon created or destroyed. .(3)An electron that is in a stationary state moves according to classical mechanics (4)For very high orbits (n)the result of the new mechanics must be c nsistent with that of classical hysics.This hypothesis isknown as Bor'sorrespondence principle Note that Bohr's of ed in Section 112.Th firs lead atural m attri to the spectr 免 e energy rence empiric rmula (1.1), th the h the hydrogen atom: (known as Bohr's levels)as well as to determine the Rydberg constant in terms of the known constants,m,e,ce h: R=2'me ch3 1.09×10cm- in agreement with its empirical value (see [Tomonaga(1968)).More- over,Bohr managed to find the size of the hydrogen atom B=mC≈052917-10-8cm (known as the Bohr radius)where a constant h related to h (pronounced "h-bar")is also known as the Planck constant. The existence of the stationary states in atoms was demonstrated in aserie of elegant experiments by Franck and

1.2 The birth of quantum mechanics 17 1.2.4 The Bohr-Sommerfeld quantization condition; de Broglie's wave 0 Quantization was thus shown to hold for electromagnetic field energy (Planck),for atomic (or molecular)oscillation energy in matter(Debye Einstein),and for atomic binding energies (Bohr),but a proper formu lation of quantum mechanics had to wait for the work of Heisenberg (1925).Schrodinger(1926).and others.It is of historical and pedag tum mechanics itself wpoireandSonmnertedatemptedtoformlaitetheideaofquatizn tion in a finite mo ion e D mme on rule state classical motions only tho satisfying the condition (writing the rule for one-dimensional motio. for simplicity) pdg=nh (n=0,1.2...) (1.18 where a and p refer to a pair of canonical variables and the integration is over a full period of classica motion,are allowed. Remarks .The limitation to quasi-periodic motions is important.Further development by Einstein covered the case of systems which are classically integrable.Some much more recent developments on eqn(1.18)deals with general systems.See Supplement 21.5. .For harmonic oscillators,(1.18)gives,in agreement with Planck's hypothesis,En=nh=nhv,which,to be rigorous,differs from the correct quantum mechanical result by an additive constant(see Section 3.4). .For the hydrogen atom,eqn(1.18)leads correctly to Bohr's energy levels. .It is important for the consistency of the formalism that the in tegral appearing in eq(1.18)is e theerne prodr a pa er appearingi the Hamiltonian is varied sowy The Bohr omerfldtio tus ouobe The last missing piece of the puzzle,which was needed to complete n the formulation of quantum mechanics.was the realization that wa ave artice duality,iscoveredfirst for the for the electron,was
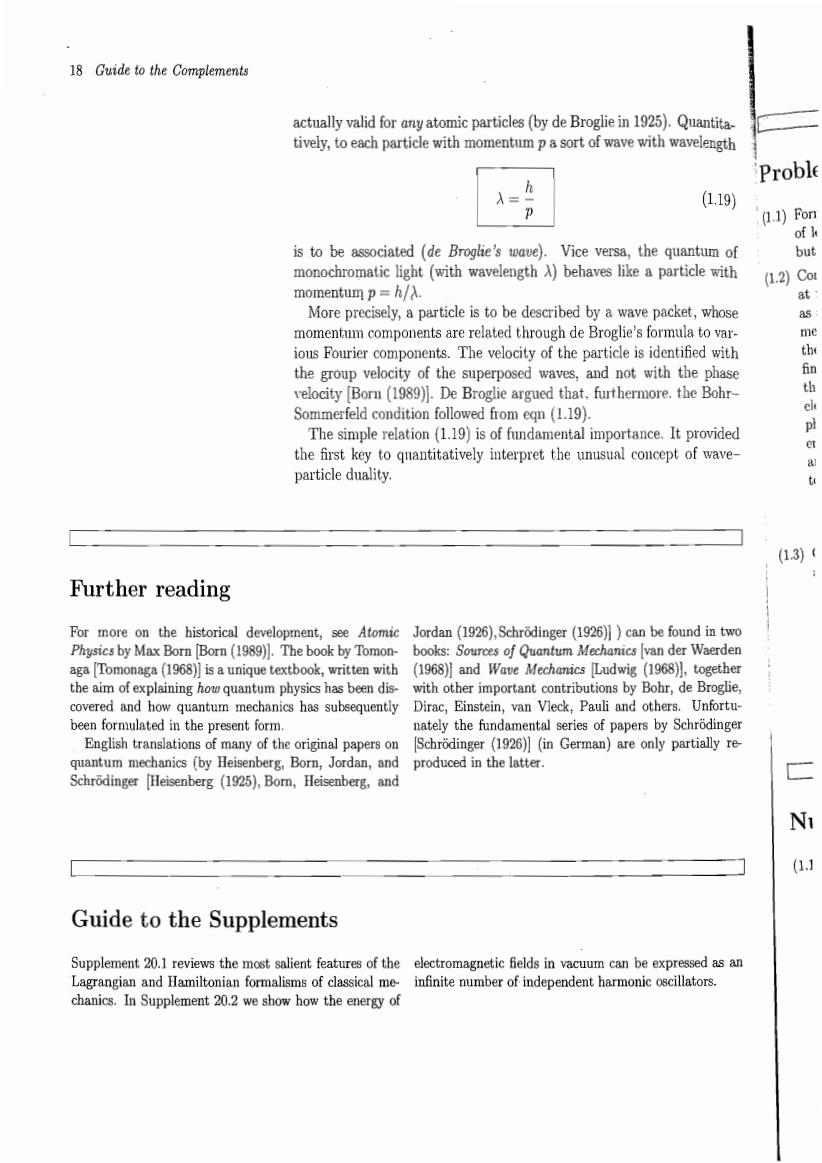
18 Guide to the Complements actually valid particles(by de Broglie n1925).Quntita tively,to each particle with momentum p a sort of wave with wavelength Proble (1.19y p is to be associated(de).Vice versa,the quantum of but monochromatic light (with wavelength A)behaves like a particle with (1)Cor momentump=h/λ at More precisely.a particle is to be described by a wave packet,whose as momentum components are related through de Broglie's formula to var. ious Fourier components.The velocity of the particle is identified with the group velocity of the superposed waves,and not with the phase velocity [Born (1989).De Broglie argued that.furthermore.the Bohr- Sommerfeld condition followed from eqn (1.19). The simple relation (1.19)is of fund lamental importance.It provided the first key to quantitatively interpret the unusual coucept of wave- (13) Further reading For more on the historical development,see Atomie Jordan(1926).Schrodinger(1926))can be found in two Physics by Max BornBorn (19)The book by Tomon-books Souesftchanic der Waerden aga [Tomonaga(1968)]is a unique textbook,written with (1968)and Wave Mechanics Ludwig (1968),together the aim of explainingoquntm physics has been dis- with other important contributions by Bohr,de Brogle covered and w quantum mechanics has subsequently Dirac,instein,van Vleck,Pauliand others ate d in the present fo the es ot papers (in German)are only par Schrodinger Heisnberg (195)Bom,Heisenberg and Ni Guide to the Supplements ures of the ele Ias an ho ho th ssical me t20 2 we e energy o

Problems the (1Consider the cttavelengthsregard eoata at rest ray with aa日 p=hvlc.where H()+9 fnal state and let and be the angks between follows from the Lagrangian the directions of the final photon momentum and electron momentum.with respect to the incident L=m号+rA-q photon momentum.The final state photon has en Coupare the Euler-Lagrange equations and Hamil- ton's equations for such a particle. (15)Showthan caca echc the volume in e occup ween(a,P s the sys m) (1.3)Consider a hydrogen atom (composed of an proton and an electron;m1836m).Calculate the re (1.6)Compute the Planck distribution for an observer Solve the moving with velocity V. dicedmas4and as variable in the form ()=Comp in a cavity filled with radiation at forr=5x 10-cm.Compute h and compare this temperatureT.Compute the frictional force on the energy with a thermal energy kT for T=290 K.k mirror. Numerical analyses (1.1)Study the temperature dependence of the Planck distribution
Quantum mechanical laws In this chapter,the main concepts of quantum mechanics are introduced. 2.1 Quantum states 21 2.2 The uncertainty principle 26 2.1 Quantum states 2.3 The fundamental postulate 29 2.4 The Schrodinger equation 37 The experiment by Tonomra e disc in Chapter1 confirins the de Broglie rel 1.19).This s us to de 2.6 Completeness 45 of a system,not in terms of simultaneous values p,)as in classical mechanics,but as a sort of a wave.According to Chapter summary Problems the fundamental law of quantum mechanics Numerical analyses the a given system is described by fuction )called the wave function quantum state(),t)1. (2.1) It depends on the canonical coordinates and on time but not on the conjugate momenta1 ISuch a des e to intr Knowledge of the wave function amounts to complete knowledge of a duce the loss of an elegant symm state It alld ne the Hamilto n ot class ty,there is an essential differ ence be of the and tha of a classical wa mechanical laws do have an analogo t as some of a space-time propagation of matter or of energy,as the case with clas rill be ex. sical waves.The interpretation of is of a probabilistic nature. For instance,the quantum mechanical probability of finding the sys tem in the coordinate interval ,+d)is given by aP =l(g),t) 2.2 (dd)For aparticle moving in ordinary three-dimensional space,the probability of observing it in the vicinity of the point r is ,2r (2.3) In a three-dimensional system with s degrees of freedom,)is a function ofs variables (e..=6for a system of two particles):