
a.The lattice and its unit in 1D: T=ma(m=0,±1,±2,.…) 5 )⊙⊙ ⊙ ,· ● Figure 1.13 A one-dimensional lattice. unit cell and its choice for one-dimensional lattice One dimensional lattice
a. The lattice and its unit in 1D: T = ma (m = 0, ±1, ± 2,…) unit cell and its choice for one-dimensional lattice One dimensional lattice
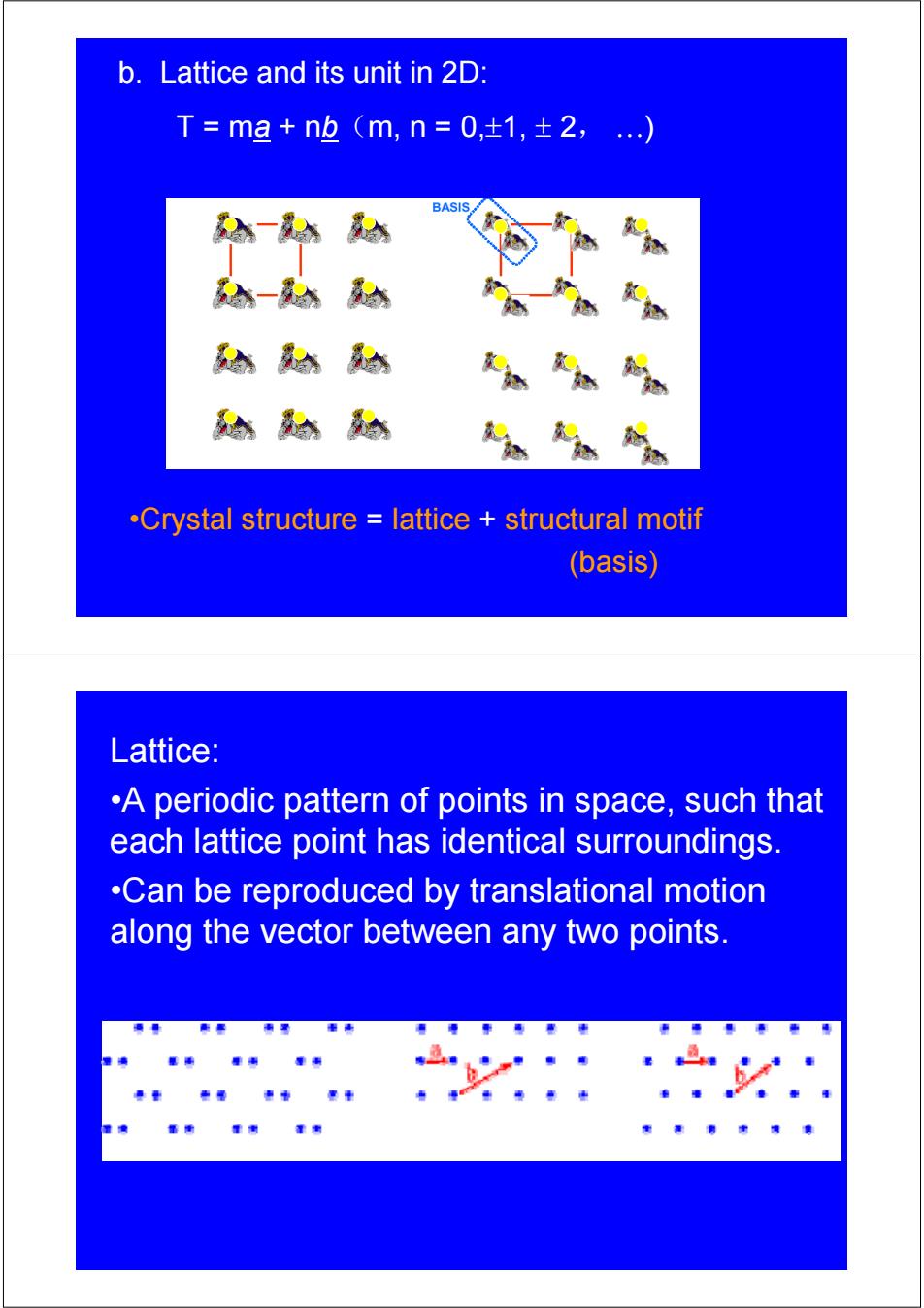
b.Lattice and its unit in 2D: T=ma+nb(m,n=0,±1,±2,.…) BASIS .Crystal structure lattice structural motif (basis) Lattice: .A periodic pattern of points in space,such that each lattice point has identical surroundings. .Can be reproduced by translational motion along the vector between any two points
b. Lattice and its unit in 2D: T = ma + nb(m, n = 0,±1, ± 2, …) •Crystal structure = lattice + structural motif (basis) Lattice: •A periodic pattern of points in space, such that each lattice point has identical surroundings. •Can be reproduced by translational motion along the vector between any two points
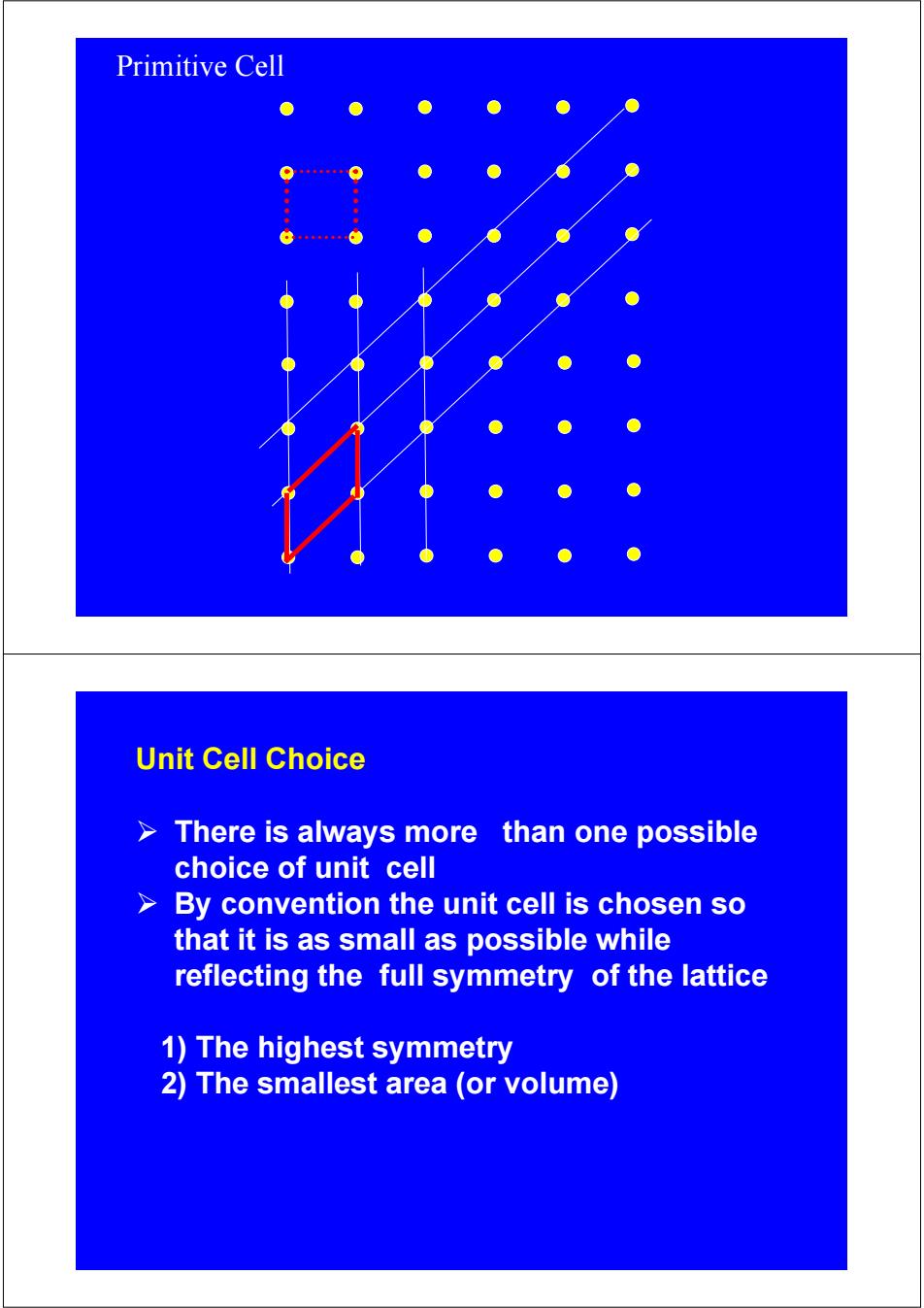
Primitive Cell Unit Cell Choice There is always more than one possible choice of unit cell > By convention the unit cell is chosen so that it is as small as possible while reflecting the full symmetry of the lattice 1)The highest symmetry 2)The smallest area (or volume)
Primitive Cell Unit Cell Choice ¾ There is always more than one possible choice of unit cell ¾ By convention the unit cell is chosen so that it is as small as possible while reflecting the full symmetry of the lattice 1) The highest symmetry 2) The smallest area (or volume)
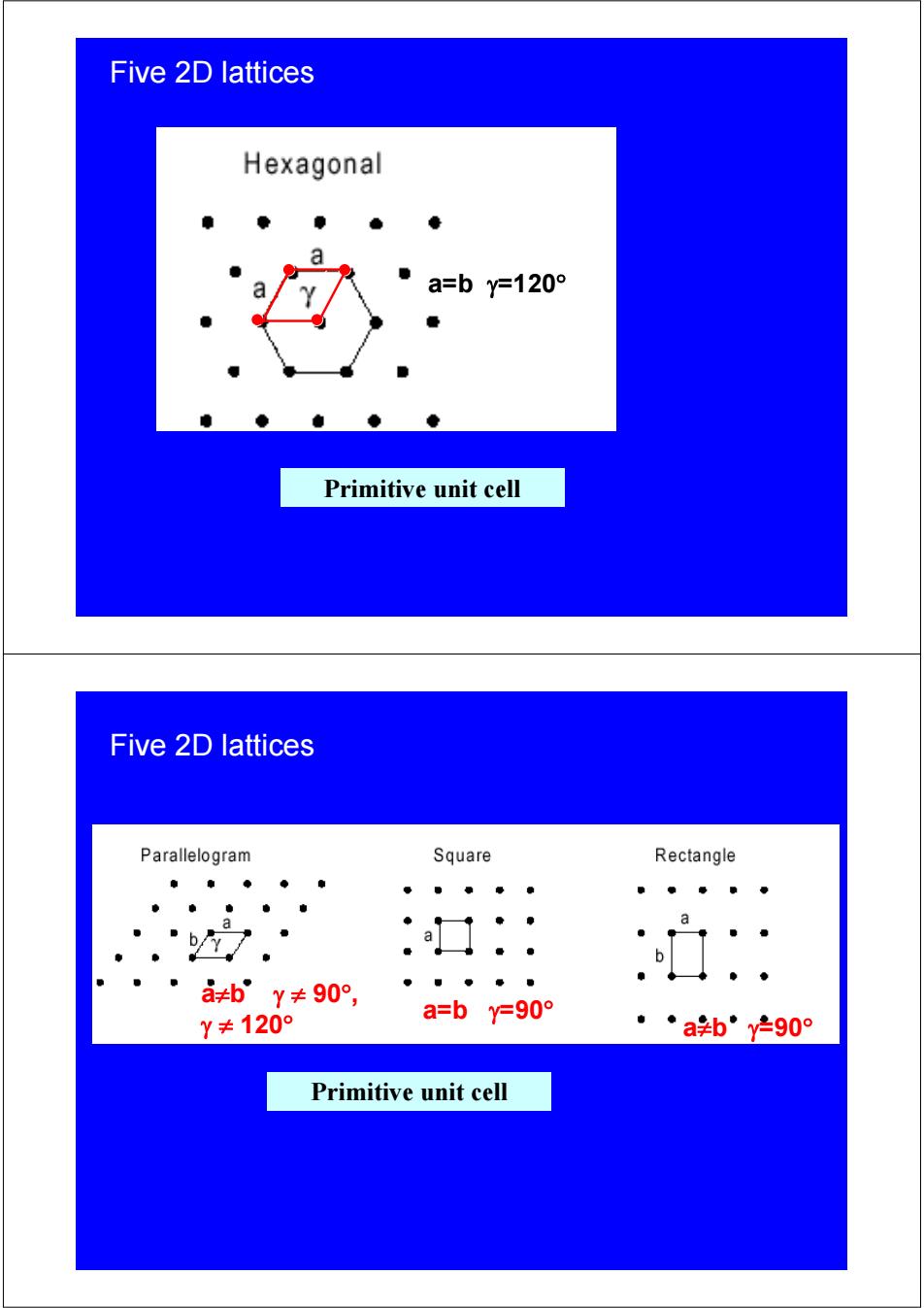
Five 2D lattices Hexagonal a=by=120° Primitive unit cell Five 2D lattices Parallelogram Square Rectangle a a≠bY≠90°, Y≠120° a=by=90° ◆ab”y-90° Primitive unit cell
Five 2D lattices a=b γ=120° Primitive unit cell Five 2D lattices a=b γ=90° a≠b γ=90° a≠b γ ≠ 90° , γ ≠ 120° Primitive unit cell
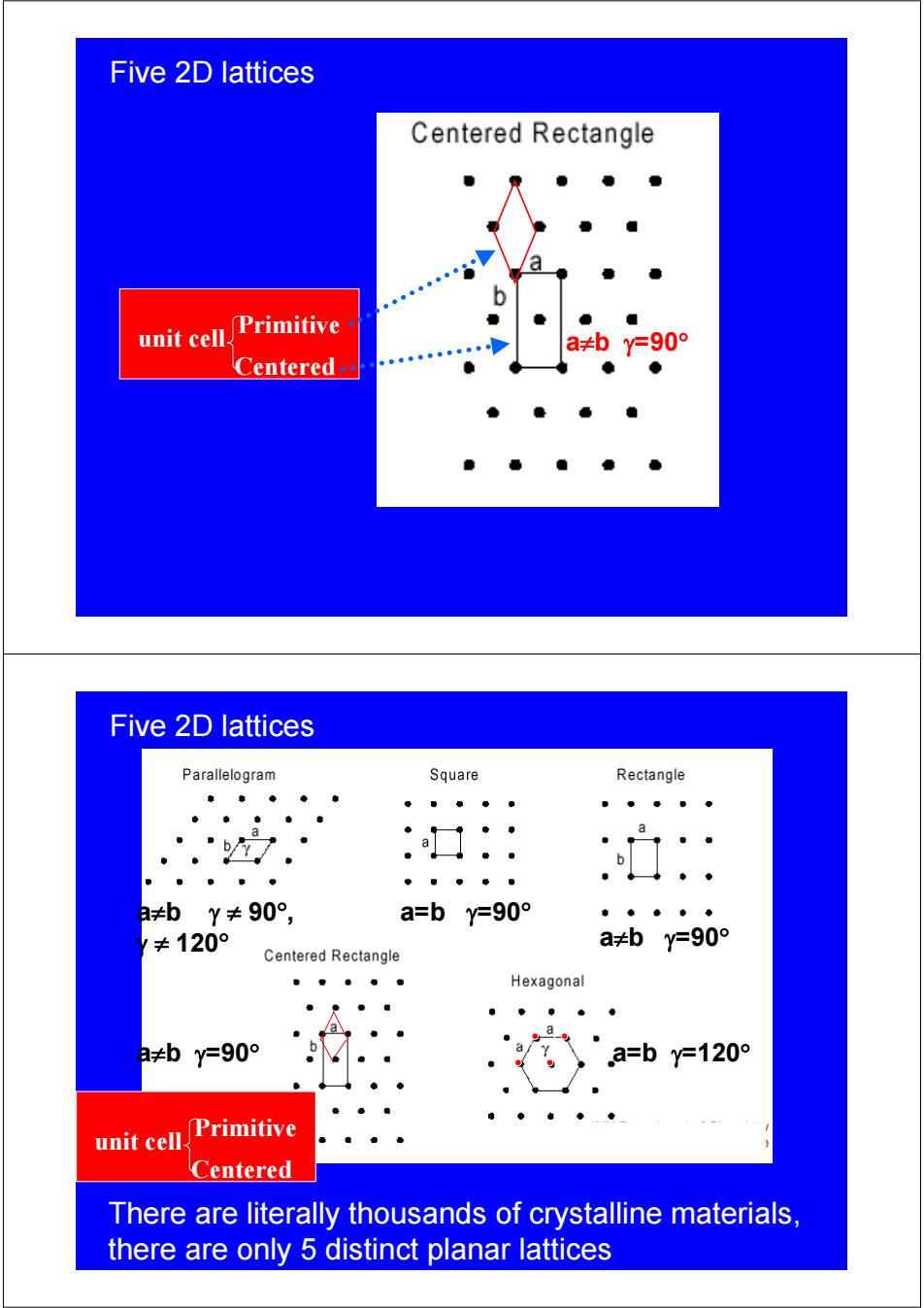
Five 2D lattices Centered Rectangle unit cell. Primitive a≠by=90° Centered Five 2D lattices Parallelogram Square Rectangle a b 日≠bY≠90°, a=by=90° ≠120° a≠by=90° Centered Rectangle Hexagonal a 2≠by=90° a=by=120° unit cell. Primitive Centered There are literally thousands of crystalline materials, there are only 5 distinct planar lattices
Five 2D lattices unit cell a≠b γ=90° Centered Primitive Five 2D lattices a=b γ=90° a≠b γ=90° a≠b γ=90° a=b γ=120° a≠b γ ≠ 90° , γ ≠ 120° unit cell Centered Primitive There are literally thousands of crystalline materials, there are only 5 distinct planar lattices