
【p-esom9 s'do 五-SNOILOHS‘五HHLdVHO SWHLIHOSTV HOHVHS IVOOT
algorithms ch sear Local 3–4 Sections 4, Chapter 1 3–4 Sections 4, Chapter
乙-tso9stmO (1μq人ua)s3 eds snonu!4uo)uI4 oueas jeoo7◇ (K9μq)swylo1eIn3u39◇ auIe3uuep34 ejnwlS◇ Suiqwip-HH◇ au!inO
Outline Hill-climbing ♦ annealing Simulated ♦ riefly) (b rithms algo Genetic ♦ riefly) b (very spaces continuous in rch sea cal Lo ♦ 2 3–4 Sections 4, Chapter

8 suonos'p ype3 s aulygo se Il3Mse∂ujuo oj∂1qe!ns‘3eds1 ue]suo 1 anoudw!on 'anens quauno,aBuis e daay :swylloe quawanodwl anneJanl asn ueo 'saseo yons ul alqeawn'squlensuo 3usies uoneunyuo puy o dSL 'uonein3yuo Tewrado puy :suoneinyuoadwo,,o as =aoeds aens uayL uolinjos ayl si gjasl!anens jeog ay :quena s!yed 'swalqod uoneziwndo Kuew ul swqqlIoSe quowanoidwr aalqeoI
algorithms t emen v impro e Iterativ irrelevant; is path roblems, p optimization many In solution the is itself state goal the configurations; “complete” of set = space state Then TSP e.g., configuration, optimal find timetable e.g., constraints, satisfying configuration find r, o rithms; algo rovement imp iterative use can cases, such In it rove imp to try state, “current” single a eep k rch sea offline as ell w as online r fo suitable space, Constant 3 3–4 Sections 4, Chapter
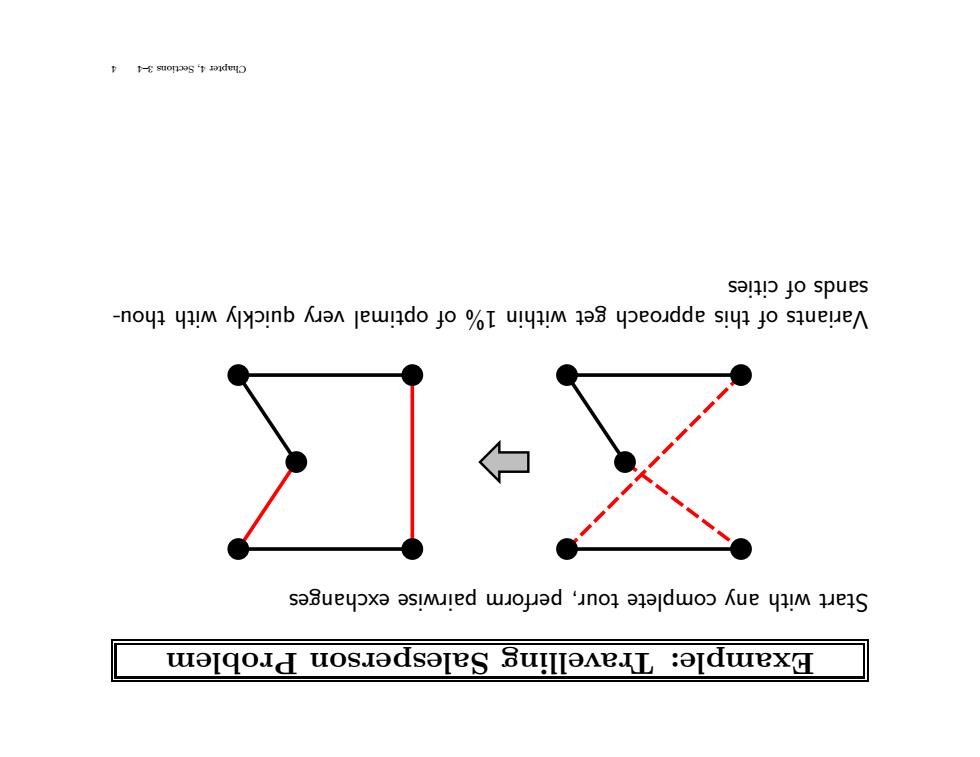
)-全so9 S'dmo sallo jo spues -noy yim Kpyinb Ken jewndo jo %I u!ylm 1 ypeoidde sy Jo squeue seBueyoxa asiMled wJojad 'non aajdwoo Kue yhIM jens walqoId uosIadsoles SurlleneIL :oldwexH
Problem erson Salesp elling v ra T Example: exchanges pairwise rm erfo p tour, complete any with rt Sta thou- with quickly very optimal of 1% within get roach app this of riants aV cities of sands 4 3–4 Sections 4, Chapter
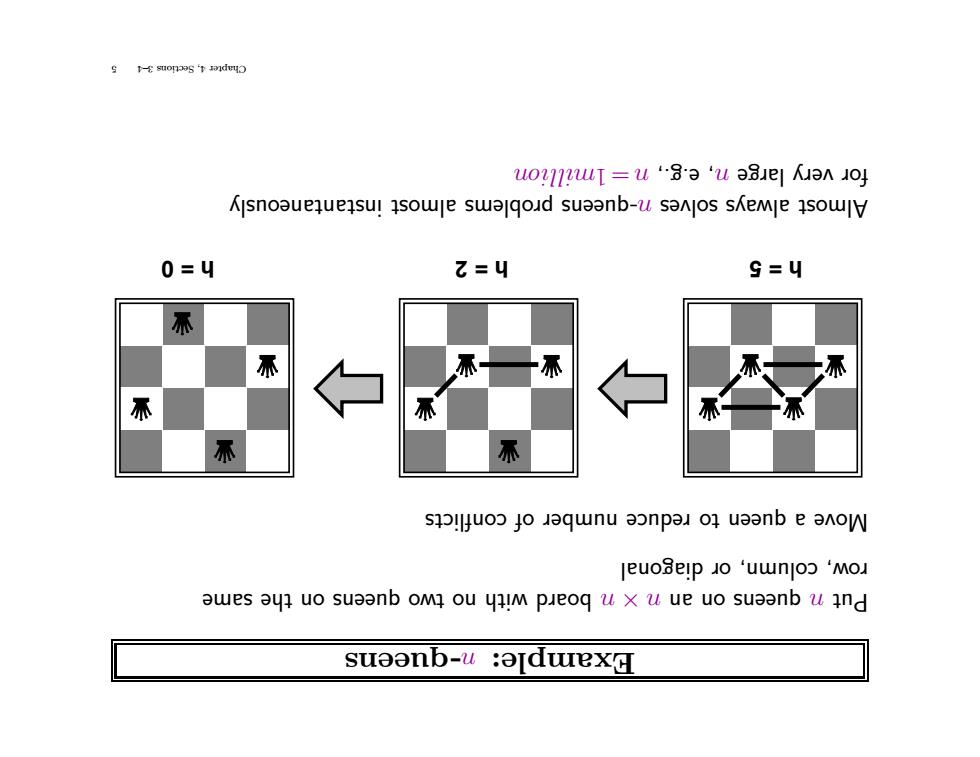
g suonos't uonpput=u "8e 'u e Kon 10 Kjsnoauenuensul asowje swajqod suaanb-u sanjos sKemje nsowly 0=4 Z=4 9=4 不 不 不 不 不 不 不 sipIHuoo o Jaqunu aonpal on uaanb e anoW leuoelp o 'uwnjoo 'Mo awes ayi uo suaanb om ou yhm pueoq u x u ue uo suaanb u ind suoonb-u :oldwexH
-queens n Example: same the on queens owt no with rd oa b n ×n an on queens n Put diagonal r o column, w, ro conflicts of er numb reduce to queen a Move h = 0 h = 2 h = 5 instantaneously almost roblems p -queens n solves ys a alw Almost ion l mil 1 =n e.g., , n rge la very r fo 5 3–4 Sections 4, Chapter