
9 derivations force on the element must equal the sum of the forces acting on the fiber tudinal modulus,E1.Static equilibrium requires that the total resultant as shown in figure 3.5(b),the response is governed by the effective longi- If the RVE in figure 3.5(a)is subjected to a longitudinal normal stress,e 3.2.1 Longitudinal Modulus ters will be identified by using subscripts as defined in the following posite lamina,the fiber,or the matrix,and the corresponding parame- The volume averaging(or area averaging)may occur over the com- which loading is applied. 8=displacement,V=volume,and A=area associated with the face on where the overbar denotes an averaged quantity,and o=stress,g=strain, E-Jedv-iJedA do not change along the length,we can just use area averages: assumed that the stresses,strains,displacements,and RVE dimensions the corresponding volume-averaged quantities.Finally,since it is the stresses,strains,and displacements at each point,we only deal with the mechanics of materials approach does not require the specification of strains in the RVE that has been subjected to a simple state of stress.Since or compatibility relationships and assumptions about either stresses or Micromechanics equations will be developed from either equilibrium elastic,and orthotropic. RVE,the lamina is assumed to be macroscopically homogeneous,linearly fiber can be either isotropic or orthotropic.Following the concept of the elastic and homogeneous.The matrix is assumed to be isotropic,but the matrix materials.The fiber and matrix materials are assumed to be linearly ing at the interface is assumed,so that no slip occurs between fiber and element,the area fractions must equal the volume fractions.Perfect bond- parallel and that the dimensions do not change along the length of the as those in the actual composite.Since it is assumed that the fibers remain The constituent volume fractions in the RVE are assumed to be the same Principles of Composite Material Mechanics e.10 910 3.15 approach. 8·150We8et leading to this equation will now be examined by using a strain energy and is a useful design equation.The validity of the key assumptions equation (3.23)agrees well with experimental data from ref.[7](fig.3.6[bl) fiber volume fraction,as shown in figure 3.6.Although simple in form, This equation predicts a linear variation of the longitudinal modulus with mixtures for the longitudinal modulus: Substitution of equation(3.22)in equation(3.21)then yields the rule of strains in the composite,fiber,and matrix along the 1 direction are equal: Finally,the key assumption is that,due to perfect bonding the average modulus,Em does not need a second subscript. to let En =Er.Since the matrix is assumed to be isotropic,the matrix boron are practically isotropic.For the isotropic case,it is a simple matter carbon and aramid fibers exhibit orthotropic behavior,whereas glass and not necessarily equal to the transverse fiber modulus,En2.For example, assumed to be orthotropic.That is,the longitudinal fiber modulus,En,is Double subscripts are used for the fiber modulus since the fiber is and equation (3.19)becomes strains are neglected), EEe=ErEn Ur+EmEmIvm orthotropic,and that all materials follow a 1-D Hooke's law (i.e.,Poisson Under the assumptions that the matrix is isotropic,that the fiber is Ge=Gnvr+miVm rearranged to give a"rule of mixtures"for longitudinal stress: are equal to the corresponding volume fractions,equation (3.18)can be tively,and the second subscript refers to the direction.Since area fractions where subscripts c,f,and m refer to composite,fiber,and matrix,respec- GcA=OnAf+miAm and matrix.Combining the static equilibrium condition with equation Effective Moduli of a Continuous Fiber-Reinforced Lamina 823) 9.22 621 620 819 318

Composite modulus energy terms can be simplified as and strains are uniform over the RVE and using equations(3.20),the strain Again making the mechanics of materials assumption that the stresses energy in the matrix,U: FIGURE 3.6 sented as the sum of the strain energy in the fibers,U,and the strain stress,the total strain energy stored in the composite,U can be repre- is possible by using a strain energy approach.Under the given state of Composites,206-217.) Further insight into the micromechanics of the longitudinal loading case for B-glass/polyester.(From Adams,R.D.,1987.Engineered Materials Handbook,Vol.1, elementary mechanics of materials models,(b)Comparison of predicted and measured E Variation of composite moduli with fiber volume fraction(a)Predicted E and E2 from 包 Fiber volume fraction Y28a6 E.3.23 E,GPa Fiber volume fraction,vr 010203040.506 Rule of mixtures A3:504m A d=20 jm .d=30 um Principles of Composite Material Mechanics 0.5 8350 G355) 25a 3.2 E 105ps Thus,the assumption of equal strains,which led to equation (3.23).is valid we find that a=2.0884,b1=0.1093,a1/b1=Gn/Gm =19.1,and Ea/Em =1.00 equation(3.24)leads to Em =0.55x10 psi (3.79 GPa) En =Er2 =10.5x10 psi (72.4 GPa) E8-1.53X100P81G0.55GPa1:-04B E-5.05X100p614.82Gpa2n-D.55 measured properties of an E-glass/epoxy composite [9], fiber strain to the matrix strain can then be found.For example,using the when composite,fiber,and matrix properties are known.The ratio of the tion(3.27)and equation (3.28)can be solved simultaneously for a and b to derive these equations.To check the strain distribution,however,equa- Note that we did not assume equal strains in fibers and matrix in order Substitution of equation(3.26),equation (3.20),and equation (3.25)in avr+biVm =1 of mixtures for stress,equation (3.19),leads to where a and bi are constants.Substitution of equation(3.26)in the rule the matrix be defined in terms of the composite stress as follows: strains is not made?"In order to proceed,let the stresses in the fibers and approach also allows us to ask,"What happens if the assumption of equal the rule of mixtures given by equation (3.23).But the strain energy with the assumption of equal strains from equation(3.22),again leads to is easily shown that substitution of equation(3.25)in equation(3.24),along ratios of the fiber and the matrix,so the approximation is justified [8].It to be of the order of the square of the difference between the Poisson's tions leading to equations [3.201).This neglected term has been shown strains at the fiber/matrix interface has been neglected(recall the assump- In this approximation,the strain energy due to the mismatch in Poisson Effective Moduli of a Continuous Fiber-Reinforced Lamina 929 328 C26
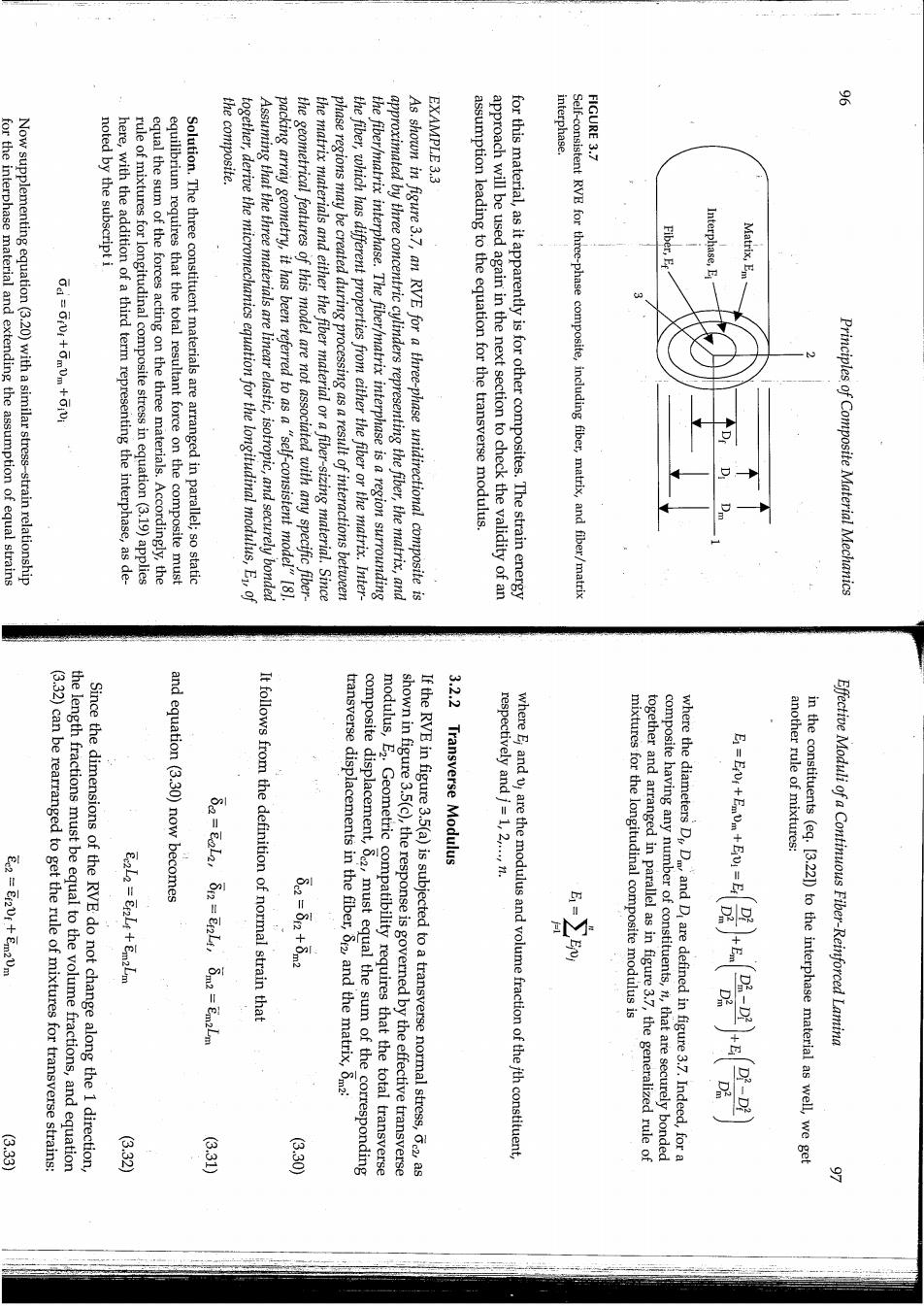
the composite. EXAMPLE 3.3 interphase. FIGURE 3.7 for the interphase material and extending the assumption of equal strains Now supplementing equation(3.20)with a similar stress-strain relationship noted by the subscript i here,with the addition of a third term representing the interphase,as de- rule of mixtures for longitudinal composite stress in equation(3.19)applies equal the sum of the forces acting on the three materials.Accordingly,the equilibrium requires that the total resultant force on the composite must Solution.The three constituent materials are arranged in parallel;so static together,derive the micromechanics equation for the longitudinal modulus,Eof Assuming that the three materials are linear elastic,isotropic,and securely bonded packing array geometry,it has been referred to as a "self-consistent model"[8] the geometrical features of this model are not associated with any specific fiber- the matrix materials and either the fiber material or a fiber-sizing material.Since phase regions may be created during processing as a result of interactions between the fiber,which has different properties from either the fiber or the matrix.Inter- the fiber/matrix interphase.The fiber/matrix interphase is a region surrounding approximated by three concentric cylinders representing the fiber,the matrix,and As shown in figure 3.7,an RVE for a three-phase unidirectional composite is assumption leading to the equation for the transverse modulus. approach will be used again in the next section to check the validity of an for this material,as it apparently is for other composites.The strain energy Self-consistent RVE for three-phase composite,including fiber,matrix,and fiber/matrix Fiber,Ee Interphase,E Matrix,Em Ga=GrVr+GmUm+Givi Principles,of Composite Material Mechanics 83-8217+2m2um (3.32)can be rearranged to get the rule of mixtures for transverse strains: the length fractions must be equal to the volume fractions,and equation Since the dimensions of the RVE do not change along the 1 direction, and equation(3.30)now becomes 83-01839+2mam It follows from the definition of normal strain that transverse displacements in the fiber,6r2,and the matrix,8m2: composite displacement,82,must equal the sum of the corresponding modulus,E2.Geometric compatibility requires that the total transverse shown in figure 3.5(c),the response is governed by the effective transverse If the RVE in figure 3.5(a)is subjected to a transverse normal stress,2,as 3.2.2 Transverse Modulus respectively and j=1,2,....n. where E and v;are the modulus and volume fraction of the jth constituent, mixtures for the longitudinal composite modulus is together and arranged in parallel as in figure 3.7,the generalized rule of where the diameters DDand D are defined in figure 3.7.Indeed,for a composite having any number of constituents,n,that are securely bonded in the constituents(eq.[3.221)to the interphase material as well,we get another rule of mixtures: Effective Moduli of a Continuous Fiber-Reinforced Lamina 3.33 8·32 830