
The homoskedastic normal distribution with a single explanatory variable y ↑fyx) E(ylx)=Bo+Bx {Normal distributions 1 X2 Econometrics 15-Zhuxi@SJTU 6
Econometrics 15 - Zhuxi@SJTU 6 . . x1 x2 The homoskedastic normal distribution with a single explanatory variable E(y|x) = b0 + b1 x y f(y|x) Normal distributions
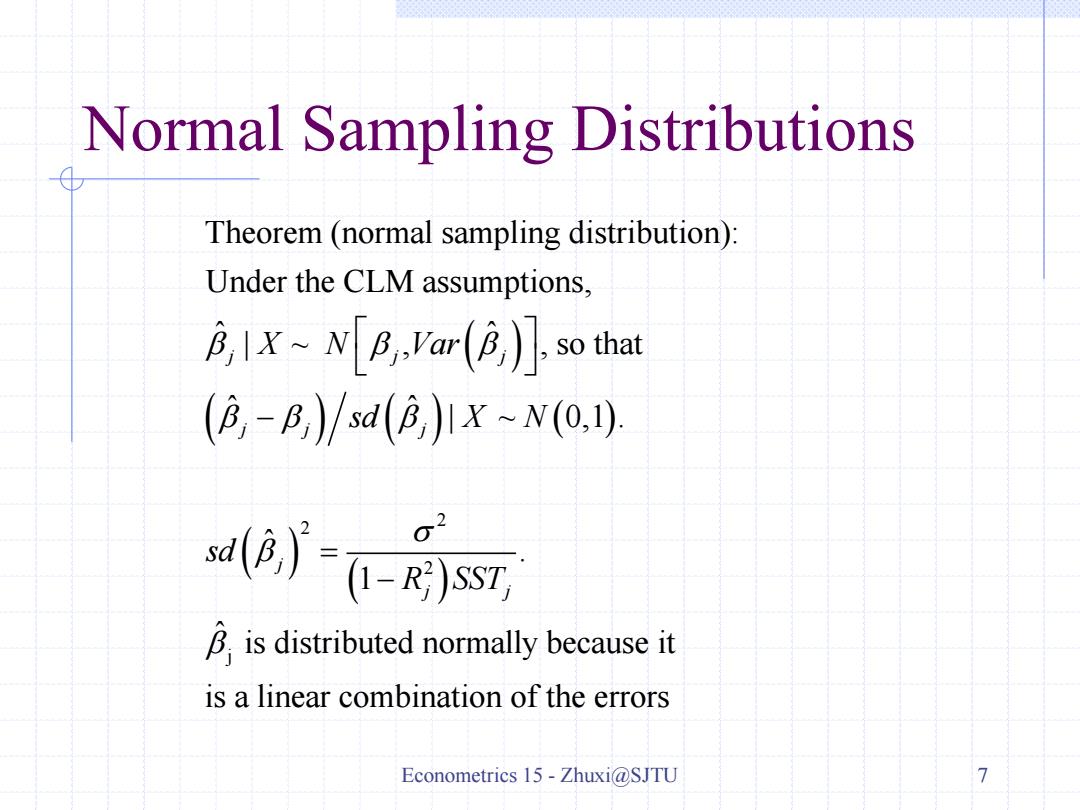
Normal Sampling Distributions Theorem (normal sampling distribution): Under the CLM assumptions, 月1x~N[B,ar(a小 so that (B-e,)/sd(B,)1X~N(o,) -周s B is distributed normally because it is a linear combination of the errors Econometrics 15-Zhuxi@SJTU 7
Econometrics 15 - Zhuxi@SJTU 7 Normal Sampling Distributions 2 2 2 j Theorem (normal sampling distribution): Under the CLM assumptions, ˆ ˆ | ~ , , so that ˆ ˆ | ~ 0,1 . ˆ . 1 ˆ is distributed normally because it is a linear combination of j j j j j j j j j X N Var sd X N sd R SST b b b b b b s b b the errors

Normal Sampling Distribution Proof: B=(XX)XY=B°+(XX)Xu =B°+Cu=B+∑Ca4, By assumption A6,we have N(,2) and u,4,u..is mutually independent, thus we have B-B°1X0N(0,o2(XX)) Econometrics 15-Zhuxi@SJTU 8
Econometrics 15 - Zhuxi@SJTU 8 Normal Sampling Distribution 1 1 0 0 0 2 1 2 3 1 0 2 Proof: ˆ . By assumption A6, we have | 0, , and , , ... is mutually independent, thus we have ˆ | 0, . ki i i i X X X Y X X X u C u C u u X N u u u X N X X b b b b s b b s
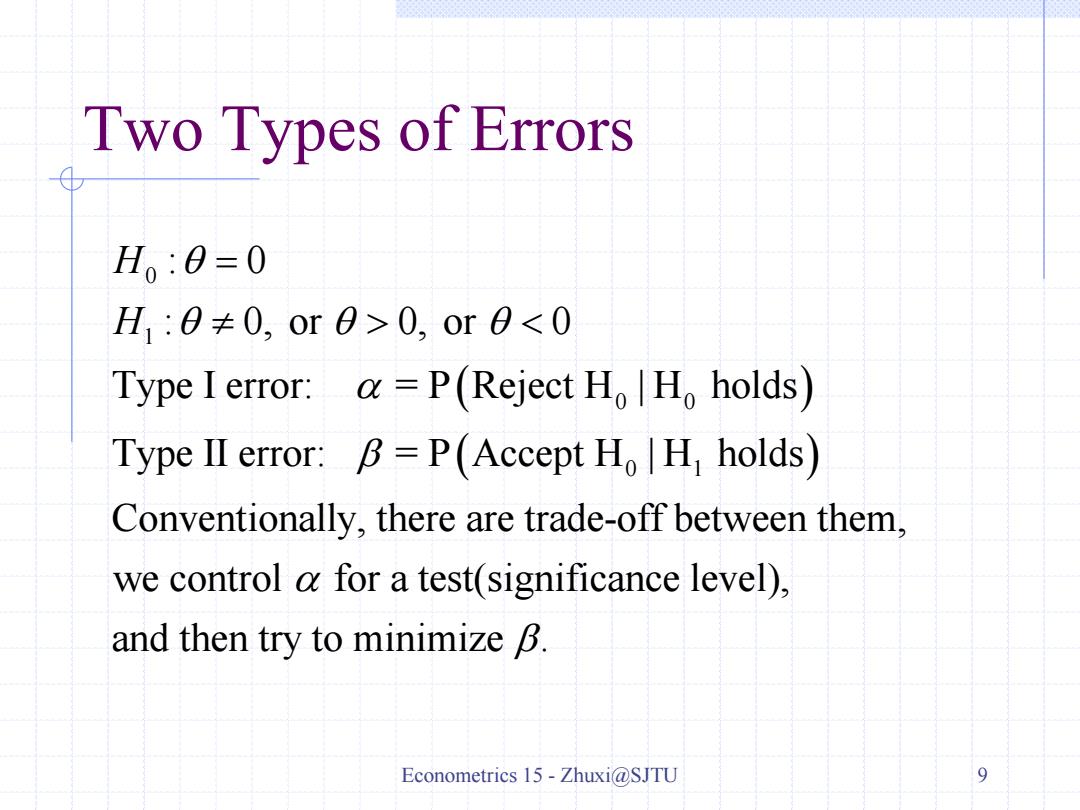
Two Types of Errors H:0=0 H:0≠0,or0>0,or0<0 Type I error:=P(Reject HoHo holds) Type II error:B=P(Accept Ho|H holds) Conventionally,there are trade-off between them, we control a for a test(significance level). and then try to minimize B. Econometrics 15-Zhuxi@SJTU 9
Econometrics 15 - Zhuxi@SJTU 9 Two Types of Errors 0 1 0 0 0 1 : 0 : 0, or 0, or 0 Type I error: = P Reject H | H holds Type II error: = P Accept H | H holds Conventionally, there are trade-off between them, we control for a test(significance level) H H b , and then try to minimize . b
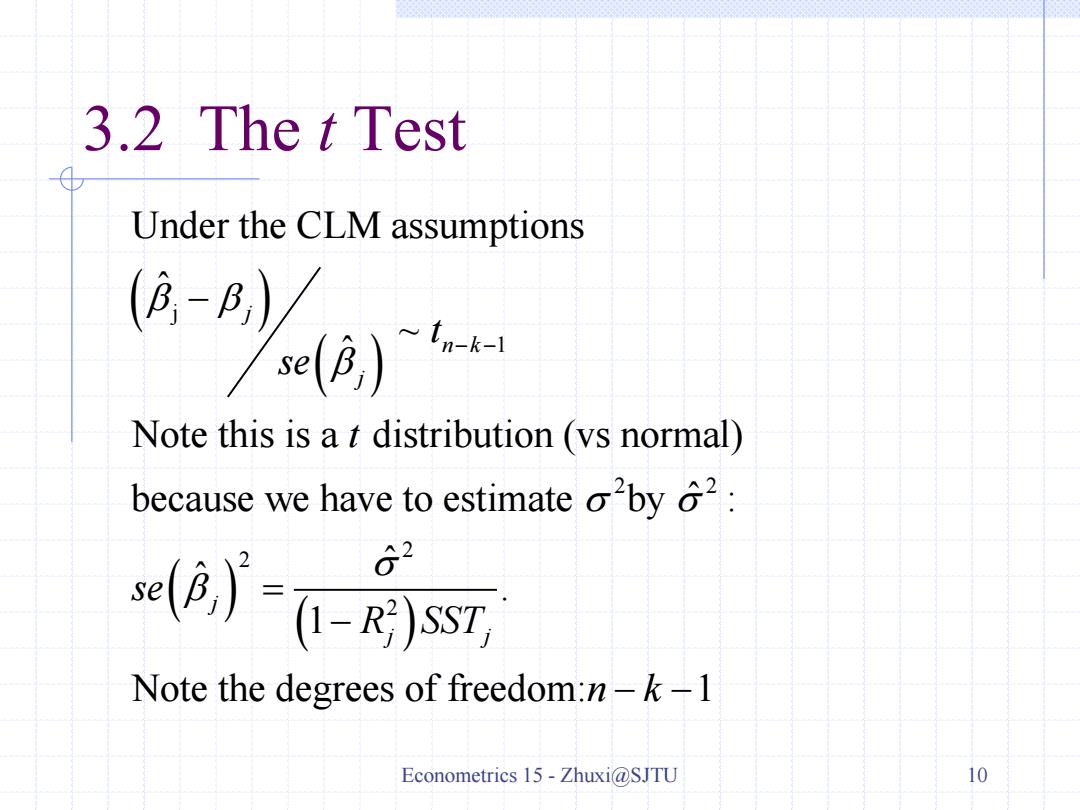
3.2 The t Test Under the CLM assumptions (B-p,)/ e(a)- Note this is a t distribution (vs normal) because we have to estimate oby 2: j (-R)Ss7, Note the degrees of freedom:n-k-1 Econometrics 15-Zhuxi@SJTU 10
Econometrics 15 - Zhuxi@SJTU 10 3.2 The t Test j 1 2 2 2 2 2 Under the CLM assumptions ˆ ~ ˆ Note this is a distribution (vs normal) because we have to estimate by : ˆ ˆ ˆ . 1 Note the degrees of freedom: 1 j n k j j j j t se t se R SST n k b b b s s s b