
Part A.Answer all eight questions(5 marks each). 1.Reaction A has a rate constant k=100s.Reaction B has a rate constant k=200 s?What can be said about the relative values of AG for these two reactions? Nothing.(The rate of the reaction is not related to its free energy change.) 2.Name a salt that could be added to a solution of the weak acid HOCl to make an acidic buffer system Anything containing the OCI ion,namely NaOCl,KOCI,etc. 3.Vehicles in the future might use hydrogen to power fuel cells which produce electricity.Name three ways that hydrogen might be stored in such a vehicle. 1.As a chilled liquid. 2.As a compresed gas 3.Dissolved in a metal as a hydride. 4.Suppose for the reaction SOSO)we wish to maximize the equilibrium concentration of SO3(g) (a)Should the temperature be high or low? Low.This is an oxidation which must therefore be exothermic.Lowering the temperature moves the equilibrium in the exothermic direction,i.e.towards more SO in this case. (b)Should the pressure be high or low? High.In any reaction,raising the pressure will move the reaction towards the side with a lower number of moles of gas,ie.towards more O in this case. 1
1 Part A. Answer all eight questions (5 marks each). 1. Reaction A has a rate constant k = 100 s-1. Reaction B has a rate constant k = 200 s-1? What can be said about the relative values of ∆Go for these two reactions? Nothing. (The rate of the reaction is not related to its free energy change.) 2. Name a salt that could be added to a solution of the weak acid HOCl(aq) to make an acidic buffer system. Anything containing the OCl- ion, namely NaOCl, KOCl, etc. 3. Vehicles in the future might use hydrogen to power fuel cells which produce electricity. Name three ways that hydrogen might be stored in such a vehicle. 1. As a chilled liquid. 2. As a compresed gas 3. Dissolved in a metal as a hydride. 4. Suppose for the reaction SO2(g) + ½ O2(g) ∏ SO3(g), we wish to maximize the equilibrium concentration of SO3(g). (a) Should the temperature be high or low? Low. This is an oxidation which must therefore be exothermic. Lowering the temperature moves the equilibrium in the exothermic direction, i.e. towards more SO3(g) in this case. (b) Should the pressure be high or low? High. In any reaction, raising the pressure will move the reaction towards the side with a lower number of moles of gas, i.e. towards more SO3(g) in this case
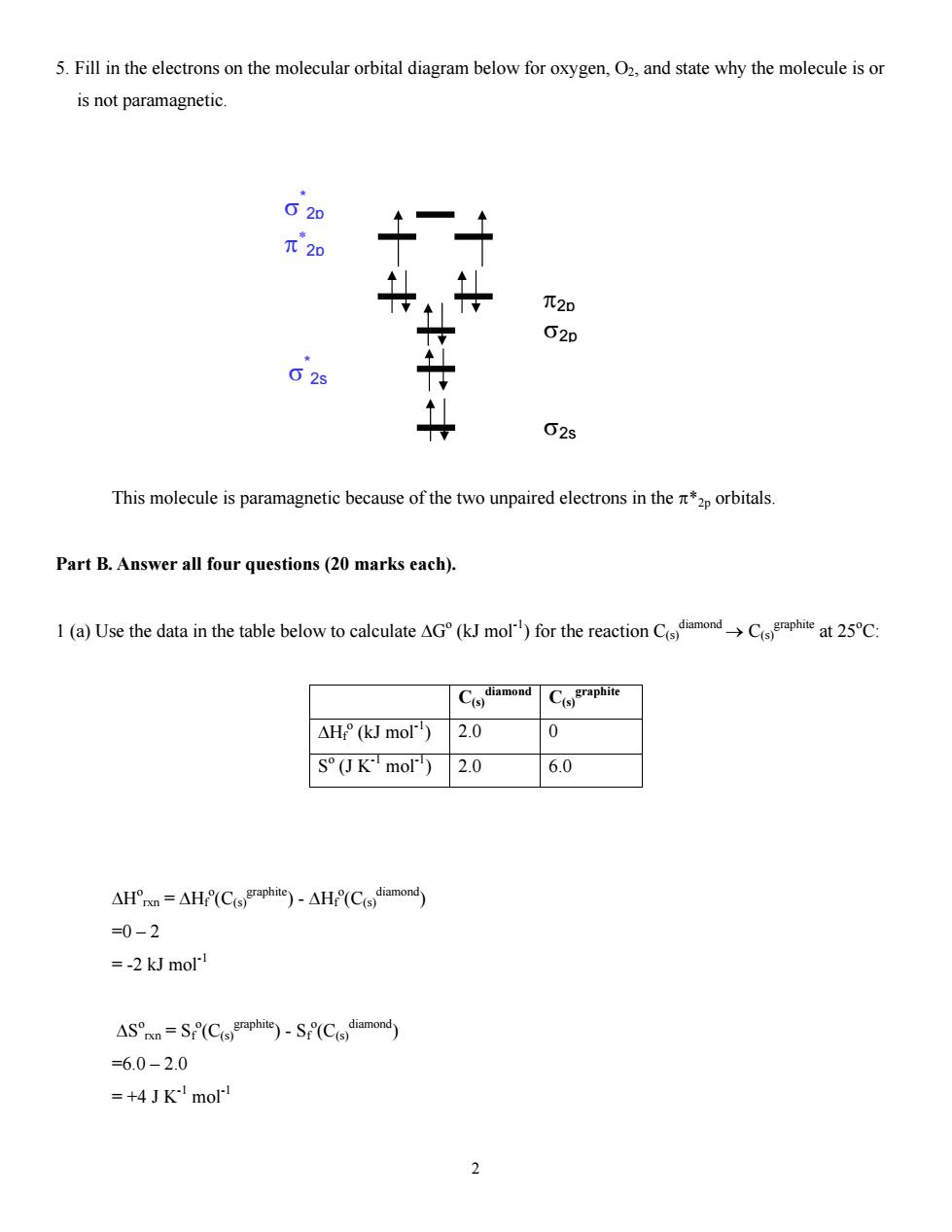
5.Fill in the electrons on the molecular orbital diagram below for oxygen,O2,and state why the molecule is or is not paramagnetic. 620 π20 T2p 620 62s 4 02s This molecule is paramagnetic because of the two unpaired electrons in theorbitals. Part B.Answer all four questions(20 marks each). 1(a)Use the data in the table below to calculate AG(kJ mo)for the reaction 25C △H°(kJmo 2.0 0 s°UKmo)2.0 6.0 △H°an=AH(Cseraphie)-AHCsdiamon时 =0-2 =-2 kJ mol AS=S(Csphite)-S(Cdiamond) =6.0-2.0 =+4 JK mol
5. Fill in the electrons on the molecular orbital diagram below for oxygen, O2, and state why the molecule is or is not paramagnetic. π2p σ2p π∗ 2p σ* 2p σ* 2s σ2s This molecule is paramagnetic because of the two unpaired electrons in the π*2p orbitals. Part B. Answer all four questions (20 marks each). 1 (a) Use the data in the table below to calculate ΔGo (kJ mol-1) for the reaction C(s) diamond → C(s)graphite at 25o C: C(s) diamond C(s)graphite ΔHf o (kJ mol-1) 2.0 0 So (J K-1 mol-1) 2.0 6.0 ΔHo rxn = ΔHf o (C(s)graphite) - ΔHf o (C(s) diamond) =0 – 2 = -2 kJ mol-1 ΔSo rxn = Sf o (C(s)graphite) - Sf o (C(s) diamond) =6.0 – 2.0 = +4 J K-1 mol-1 2

AG°m=AHm-TASm =-2000Jmo-(25+273)K(+4JKmo) =-3,192Jmol =-3.19 kJ mol (b)Is this reaction spontaneous?If so,why do diamonds not appear to spontaneously change to graphite? The reaction is spontaneous,but it is so slow that it can not be observed. Part C.Answer any three of the six questions.If you answer more than three,the best three will be used to calculate your mark(20 marks each). 1. For a Galvanic cell using the half-reactions: VO2(+2Hg+e>VO*ag+H2Od) E°=1.00V Zn2am+2e→Zng E°=-0.76V (a)Determine the overall balanced cell reaction. 2 VO2'(+4H'(ag)+22 VO(ag)+2 H2O0 Zng→Zn2am+2e 2 VO2(ag)+4 H'(ag)+Zn(s)>2 VO(ag)+2 H2Od)+Zn(ag (We know the reaction is in this direction because it must be a Galvanic cell,i.e.it must have E) (b)Calculate the standard cell potential (V). E°el=1.00+0.76=1.76V
3 ΔGo rxn = ΔHo rxn - TΔSo rxn = -2000 J mol-1 – (25+273)K(+4 J K-1 mol-1) = -3,192 J mol-1 = -3.19 kJ mol-1 (b) Is this reaction spontaneous? If so, why do diamonds not appear to spontaneously change to graphite? The reaction is spontaneous, but it is so slow that it can not be observed. Part C. Answer any three of the six questions. If you answer more than three, the best three will be used to calculate your mark (20 marks each). 1. For a Galvanic cell using the half-reactions: VO2 + (aq) + 2 H+ (aq) + e− → VO+2 (aq) + H2O(l) Eo = 1.00 V Zn+2 (aq) + 2 e− → Zn(s) Eo = −0.76 V (a) Determine the overall balanced cell reaction. 2 VO2 + (aq) + 4 H+ (aq) + 2 e− → 2 VO+2 (aq) + 2 H2O(l) Zn(s) → Zn+2 (aq) + 2 e− ________________________________________________ 2 VO2 + (aq) + 4 H+ (aq) + Zn(s) → 2 VO+2 (aq) + 2 H2O(l) + Zn+2 (aq) (We know the reaction is in this direction because it must be a Galvanic cell, i.e. it must have Eo cell>0) (b) Calculate the standard cell potential (V). Eo cell = 1.00 + 0.76 = 1.76 V

(c)At 10C,suppose [VO2]=2.0 M,[VO]=0.010 M,[H']=0.50 M and [Zn2]=0.10 M Calculate the cell potential under these conditions(V). Q=0✉Zn-0o10010=40x10 [V02aHa广(2.0'(0.50) E=E-RTIn(Q) nF -1.76J-83141K-mor273+10K1n40x10 c2(96487Cmol) =1.76J 1s82 =1.88V 2.Calculate the pH and the concentrations(mol L)ofall species present(except water)in a 1.0 M solution of carbonic acid,H2CO)For this acid,Ka=4.3x 107 and K2=5.6x 10 H2CO3a)+H2O)I HCO3)+H3O(aQ) [HCO,H,O'] H,C0】 H2CO3)HCO3()H30(a) Initial 10 0 0 Change +x +x Equilibrium 1.0-x Thus at equilibrium
(c) At 10o C, suppose [VO2 + (aq)] = 2.0 M, [VO+2 (aq)] = 0.010 M, [H+ (aq)] = 0.50 M and [Zn+2] = 0.10 M. Calculate the cell potential under these conditions (V). 22 2 2 (aq) (aq) 5 2 4 24 2 (aq) (aq) 0 1 1 5 1 [VO ] [Zn ] (0.010) (0.10) Q 4.0 10 [VO ] [H ] (2.0) (0.50) RT E E ln(Q) nF J 8.314J K mol (273 10)K 1.76 ln(4.0 10 ) C 2(96487 C mol ) J J 1.76 ( 0.12 ) C C J 1.88 C 1.88 V + + − + + − − − − = == = − + = − × = −− = = × 2. Calculate the pH and the concentrations (mol L-1) of all species present (except water) in a 1.0 M solution of carbonic acid, H2CO3(aq). For this acid, Ka1 = 4.3 x 10-7 and Ka2 = 5.6 x 10-11. H2CO3(aq) + H2O(l) ∏ HCO3 - (aq) + H3O+ (aq) 1 3 (aq) 3 (aq) a 2 3(aq) [HCO ][H O ] K [H CO ] − + = H2CO3(aq) HCO3 - (aq) H3O+ (aq) Initial 1.0 0 0 Change -x +x +x Equilibrium 1.0-x x x Thus at equilibrium, 4

x=K=4.3x10 1.0-x x2=4.3×10-1.0-x) x2+4.3×10-7x-4.3×10-7=0 a=1b=4.3×10-7:c=-4.3×10-7 x=-b±v6-4ac 2a -4.3x10'±V85x109-40-43x10 21) -4.3×107±1.31×10-3 =-6.55×104or6.55×10 Thus,x=[HCO3(ao]=[H3O'(g]=6.55x 10mol L pH=-log10H,0aml=-log10(6.55x10)=3.18 HCO3(+H2OdIICO3+H3O K.-CO.H [HCO,(m】 or.CO5.6x10molL [H,0】 K 1.0×10-4 [oH✉小FH,0】6.55x10 =1.53×10-1"molL 3.For the reaction 2 NON+)the value of Kp at 1000C is 1.78x 10.If 1.00 atm of NO is placed in a flask at 1000C.calculate the equilibrium partial pressures(atm)of all three gases p(NO(g),atm p(N2(g)),atm p(O2(g)),atm Initial 1.00 0 0 Change -2X +x 5
1 7 a 2 7 27 7 7 7 2 7 13 7 3 4 4 x(x) K 4.3 10 1.0 x x 4.3 10 (1.0 x) x 4.3 10 x 4.3 10 0 a 1; b 4.3 10 ; c 4.3 10 b b 4ac x 2a 4.3 10 1.85 10 4(1)( 4.3 10 ) 2(1) 4.3 10 1.31 10 2 6.55 10 or 6.55 10 − − − − − − − − − − − − = =× − =× − +× −× = = = × =− × −± − = −× ± × − −× = −× ± × = =− × × −7 Thus, x = [HCO3 - (aq)] = [H3O+ (aq)] = 6.55 x 10-4 mol L-1 pH = -log10[H3O+ (aq)] = -log10(6.55 x 10-4) = 3.18 HCO3 - (aq) + H2O(l) ∏ CO3 -2 (aq) + H3O+ (aq) 2 2 2 2 3 (aq) 3 (aq) a 3 (aq) 2 1 a 3 (aq) 3 (aq) a 3 (aq) [CO ][H O ] K [HCO ] K [HCO ] or, [CO ] K 5.6 10 mol L [H O ] − + − − − − + = = == × 1 −1 14 w 11 1 (aq) 4 3 (aq) K 1.0 10 [OH ] 1.53 10 mol L [H O ] 6.55 10 − − − + − × = = =× × − 3. For the reaction 2 NO(g) ∏ N2(g) + O2(g), the value of Kp at 1000o C is 1.78 x 107 . If 1.00 atm of NO(g) is placed in a flask at 1000o C, calculate the equilibrium partial pressures (atm) of all three gases. p(NO(g)), atm p(N2(g)), atm p(O2(g)), atm Initial 1.00 0 0 Change -2x +x +x 5