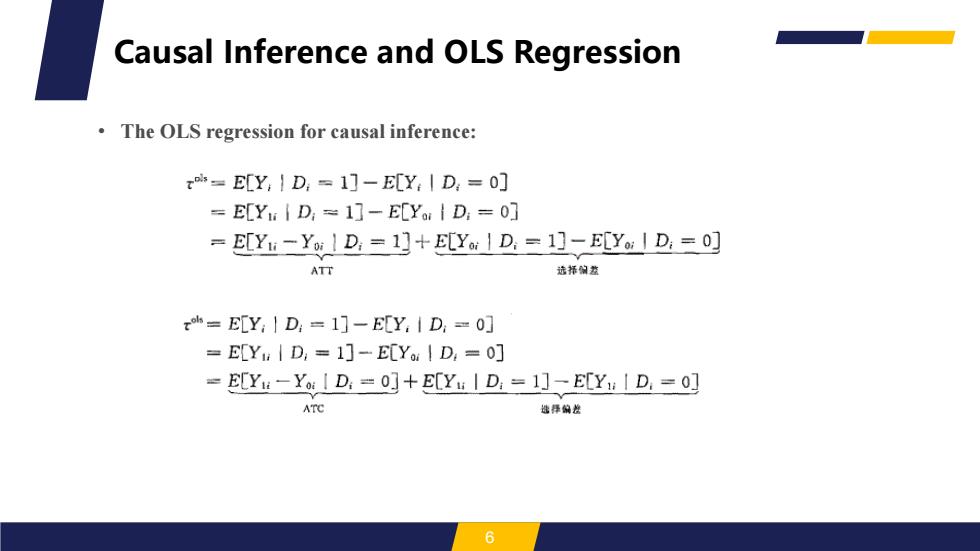
Causal Inference and OLS Regression The OLS regression for causal inference: t E[Y,D:=1]-ECY:D.=0] =ECY D,≈1]-ECY1D=0] =E[Y:-Y1D,=1]+E[Yi|D.=1]-E[Y:ID:=o] ATT 远择纳盐 E[Y;D;1]-ECY,I D=0] =E[Y D,1]-ECYor D,=0] ECYu:-Yoi I D:=0]+ECYu I D:1]-ECY1:I D.=0] ATC 进保编丝 6
• The OLS regression for causal inference: 6 Causal Inference and OLS Regression

Outline Causal Inference and OLS Regression 。Control Variable ·Fixed Effect 7
• Causal Inference and OLS Regression • Control Variable • Fixed Effect 7 Outline
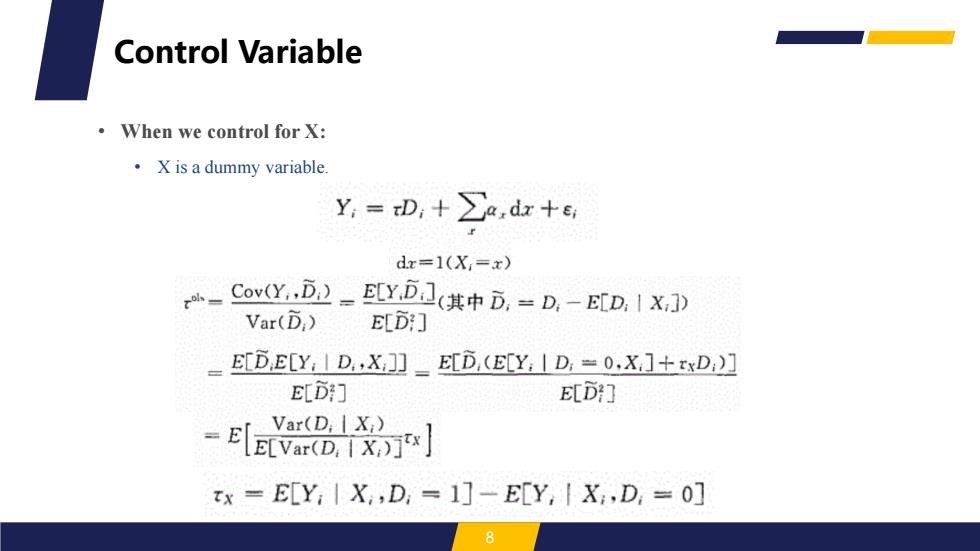
Control Variable ·When we control for X: ·X is a dummy variable Y:=tD,+∑adr+e, dx=1(X,=x) -CovY.D2-EY.Dl(其中D.=D-ED,1X]) Var(D;) E[D:] E[D.E[Y:I D.,X:]]E[D.(ECY:I D:=0.X.]+rxD ) ELD] E[D] ELVar(D0 Var(D,Xi) xx=E[Y,IX,D,=1]-E[Y,|X:,D,=0] 8
• When we control for X: • X is a dummy variable. 8 Control Variable

Control Variable ·When we control for X: If ty indicates a causal effect,we can infer that Tols has a causal effect: cx=E[Y,|X,D,=1]-EY,|X,D,=0] =E[Y X,D;1]-E[Yoi Xi,D=0] =E[Yi -YoiI X.D 1]+E[Yo.I X,D.1]-ECYo I X,D =0] TATTIX,】 选轻编差 =E[Y-Yo X:]+E[Yo I X:,D:=1]-E[Yo I X,D =0] FATE(X) 选韩编忍 +(1-(X))(ECYu-Yo i X,D 1]-ECYi-Yoi I X,D=0]) 两国网果效应编左 E[Y:|X,D=1]=E[Yu|X,D,=0] (Y,Yi)ILD,Xi
• When we control for X: • If 𝜏𝑋 indicates a causal effect, we can infer that 𝜏𝑜𝑙𝑠 has a causal effect: 9 Control Variable

Control Variable When we control for X: Selection bias equals 0: EYo Xi,D;=1]=E[Yoi Xi,D:=0 The difference in treatment effect is 0: E[Y:IX,D:=1]=E[Y|X:,D,=0] ·Unconfoundedness(非混杂性) (Yoi,Yi)IL D,X: 10
• When we control for X: • Selection bias equals 0: • The difference in treatment effect is 0: • Unconfoundedness (非混杂性): 10 Control Variable