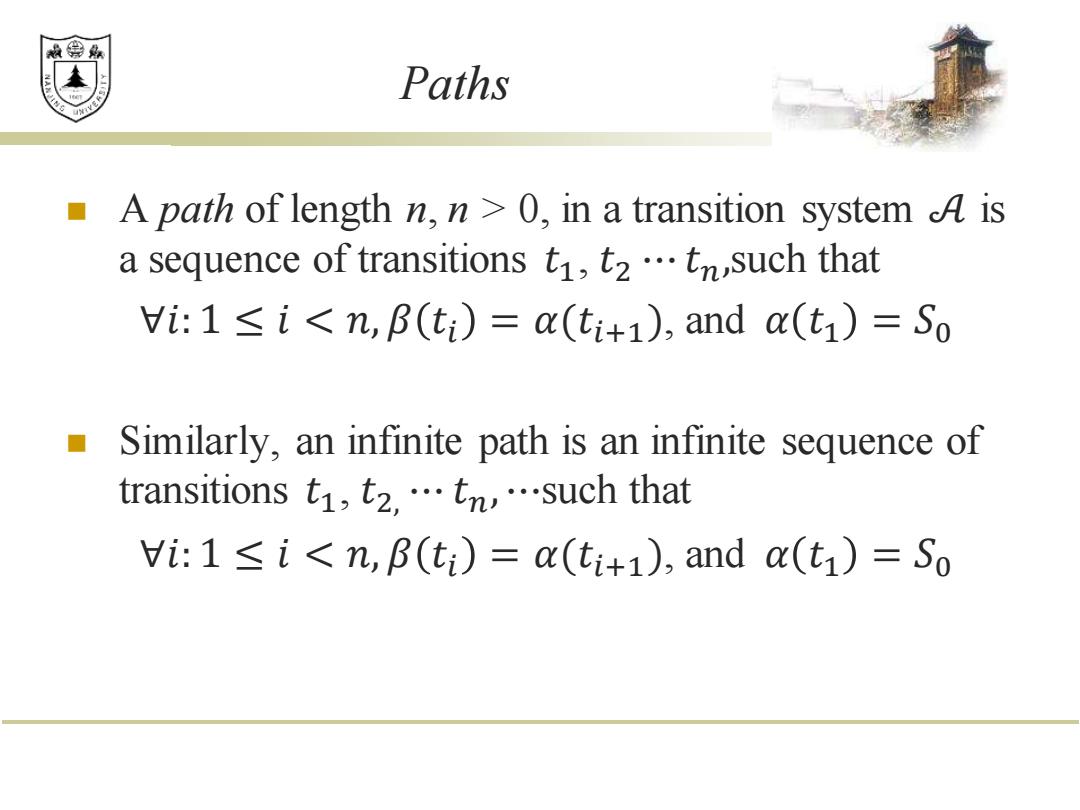
效绵鼎 Paths A path of length n,n>0,in a transition system A is a sequence of transitions ti,t2.tn,such that Vi:1<i<n,B(ti)=a(ti+1),and a(t)=So Similarly,an infinite path is an infinite sequence of transitions t,t2,…tn…such that Vi:1<i<n,B(ti)=a(ti+1),and a(t)=So
Paths ◼ A path of length n, n > 0, in a transition system 𝒜 is a sequence of transitions 𝑡1 , 𝑡2 ⋯ 𝑡𝑛,such that ∀𝑖: 1 ≤ 𝑖 < 𝑛, 𝛽 𝑡𝑖 = 𝛼(𝑡𝑖+1 ), and 𝛼 𝑡1 = 𝑆0 ◼ Similarly, an infinite path is an infinite sequence of transitions 𝑡1 , 𝑡2, ⋯ 𝑡𝑛, ⋯such that ∀𝑖: 1 ≤ 𝑖 < 𝑛, 𝛽 𝑡𝑖 = 𝛼(𝑡𝑖+1 ), and 𝛼 𝑡1 = 𝑆0
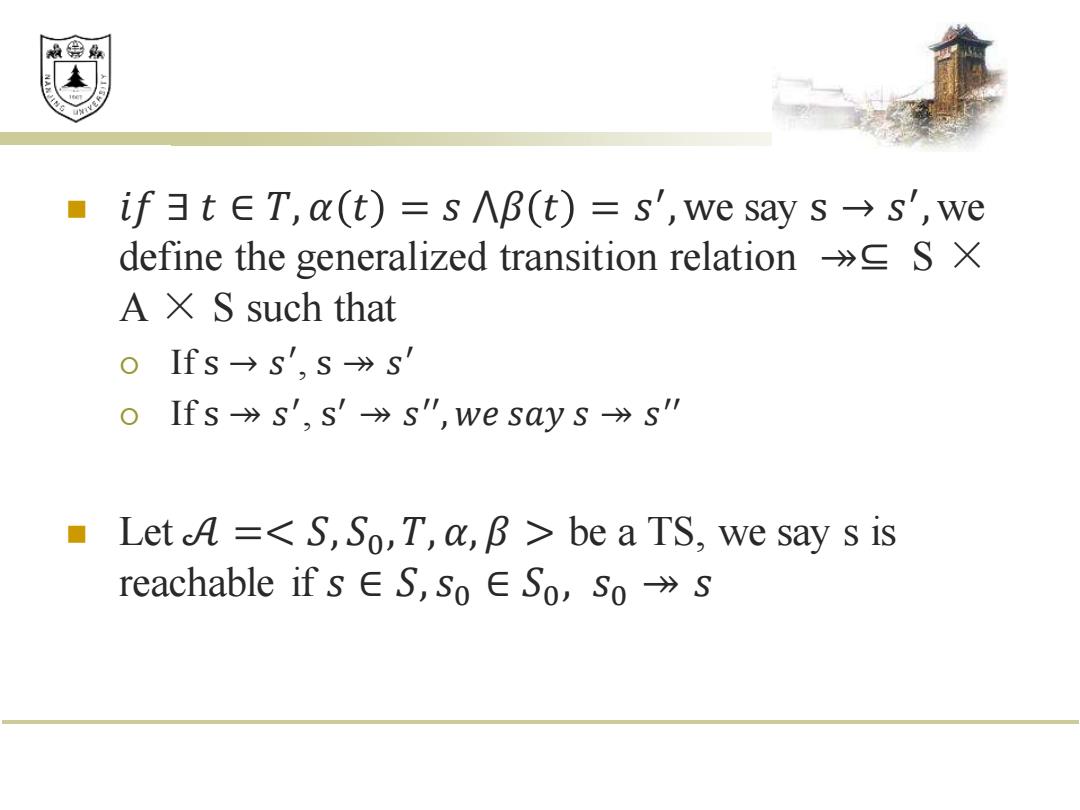
效绵鼎 if3tET,(t)=s∧B(t)=s',we say s→s',we define the generalized transition relation S X A X S such that 0IfS→s',S》s' Ifss',s'-》s",we say s》s" ■ Let A=<S,So,T,a,B be a TS,we say s is reachable if s∈S,So∈So,SoS
◼ 𝑖𝑓 ∃ 𝑡 ∈ 𝑇, 𝛼 𝑡 = 𝑠 ⋀𝛽 𝑡 = 𝑠 ′ ,we say s → 𝑠 ′ , we define the generalized transition relation ↠⊆ S × A × S such that If s → 𝑠 ′ , s ↠ 𝑠 ′ If s ↠ 𝑠 ′ , s ′ ↠ 𝑠 ′′ , 𝑤𝑒 𝑠𝑎𝑦 𝑠 ↠ 𝑠 ′′ ◼ Let 𝒜 =< 𝑆, 𝑆0 ,𝑇, 𝛼, 𝛽 > be a TS, we say s is reachable if 𝑠 ∈ 𝑆, 𝑠0 ∈ 𝑆0 , 𝑠0 ↠ 𝑠

效绵县 Let T be a transition system.A state s is a terminal state of T if there are no state s'such that s->s'. A state s is a deadlock state of T if s is reachable and terminal. 2 3
◼ Let T be a transition system. A state s is a terminal state of T if there are no state s’ such that s → 𝑠 ′ . ◼ A state s is a deadlock state of T if s is reachable and terminal
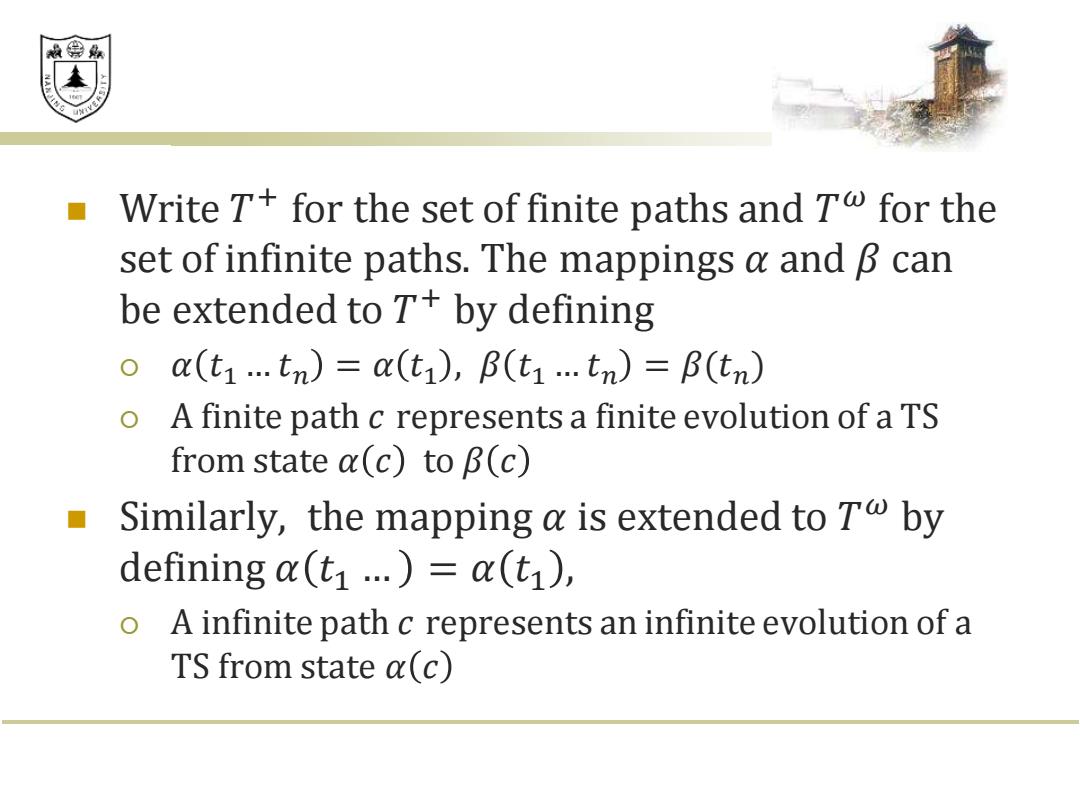
效绵鼎 Write T+for the set of finite paths and T for the set of infinite paths.The mappings a and B can be extended to T+by defining oa(ti...tn)=a(t),B(ti...tn)=B(tn) o A finite path c represents a finite evolution of a TS from state a(c)to B(c) Similarly,the mapping a is extended to T by defining a(t..)=a(t), 0 A infinite path c represents an infinite evolution of a TS from state a(c)
◼ Write 𝑇 + for the set of finite paths and 𝑇 𝜔 for the set of infinite paths. The mappings 𝛼 and 𝛽 can be extended to 𝑇 + by defining 𝛼 𝑡1 … 𝑡𝑛 = 𝛼 𝑡1 , 𝛽 𝑡1 … 𝑡𝑛 = 𝛽(𝑡𝑛) A finite path 𝑐 represents a finite evolution of a TS from state 𝛼 𝑐 to 𝛽 𝑐 ◼ Similarly, the mapping 𝛼 is extended to 𝑇 𝜔 by defining 𝛼 𝑡1 … = 𝛼 𝑡1 , A infinite path 𝑐 represents an infinite evolution of a TS from state 𝛼 𝑐
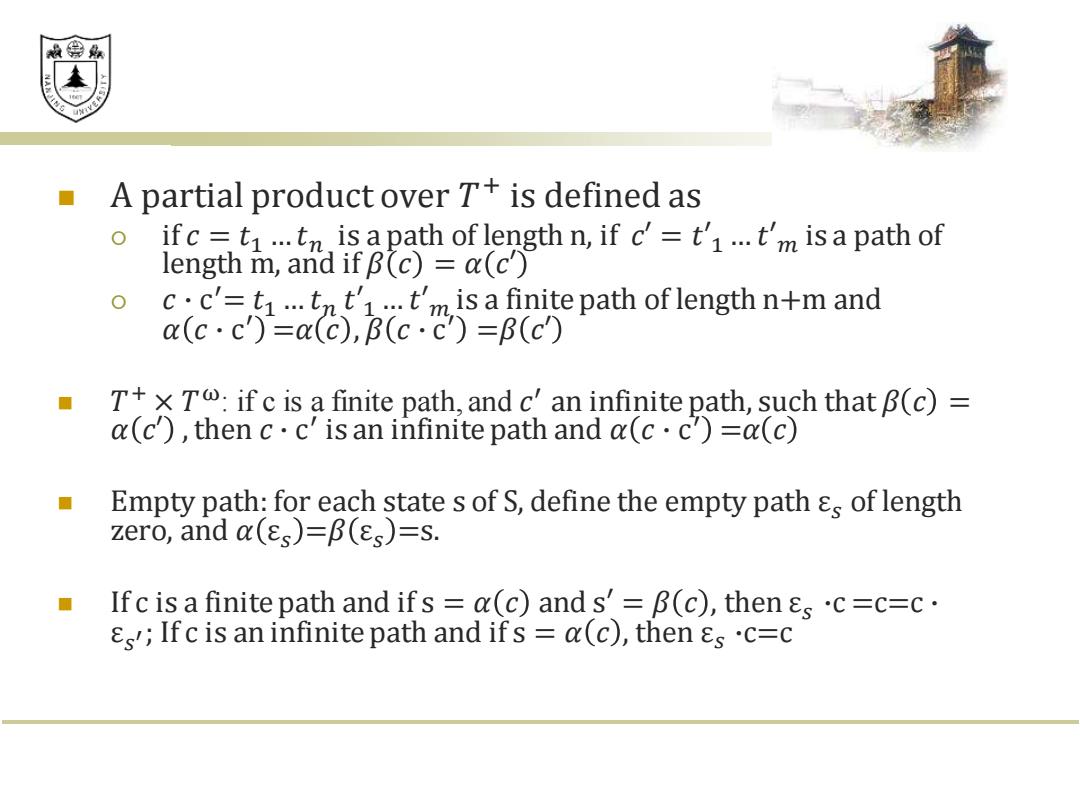
效绵鼎 ■ A partial product over T+is defined as 0 ifc=ti...tn is apath of length n,if c'=t'1...t'm is a path of length m,and if B(c)=a(c) cc'=ti...tnt't'm is a finite path of length n+m and a(c·c)=a(C),B(c·c)=B(c) T+x T:if c is a finite path,and c'an infinite path,such that B(c)= a(c),then c.c'is an infinite path and a(c.c=a(c) Empty path:for each state s of S,define the empty path &s of length zero,and a(s)=B(s)=s. Ifc is a finite path and ifs a(c)and s'=B(c),then sc=c=c. Ifc is an infinite path and ifs =a(c),then s.c=c
◼ A partial product over 𝑇 + is defined as if 𝑐 = 𝑡1 …𝑡𝑛 is a path of length n, if 𝑐′ = 𝑡′1 … 𝑡′𝑚 is a path of length m, and if 𝛽 𝑐 = 𝛼 𝑐′ 𝑐 ∙ c ′ = 𝑡1 … 𝑡𝑛 𝑡′1 … 𝑡′𝑚 is a finite path of length n+m and 𝛼 𝑐 ∙ c ′ =𝛼 𝑐 , 𝛽 𝑐 ∙ c ′ =𝛽 𝑐′ ◼ 𝑇 + × 𝑇 ω: if c is a finite path, and 𝑐 ′ an infinite path, such that 𝛽 𝑐 = 𝛼 𝑐′ ,then 𝑐 ∙ c ′ is an infinite path and 𝛼 𝑐 ∙ c ′ =𝛼 𝑐 ◼ Empty path: for each state s of S, define the empty path ε𝑠 of length zero, and 𝛼 ε𝑠 =𝛽 ε𝑠 =s. ◼ If c is a finite path and if s = 𝛼 𝑐 and s′ = 𝛽 𝑐 , then ε𝑠 ∙c =c=c ∙ ε𝑠 ′ ; If c is an infinite path and if s = 𝛼 𝑐 , then ε𝑠 ∙c=c