
模拟带宽:物理介质的截止频率,以Hz为单位度量 数字带宽:信道的最大数据速率,以bps来计量 数字速率是数字传输过程中采用物理信道模拟带宽所 能获得的最终结果
模拟带宽:物理介质的截止频率,以Hz为单位度量 数字带宽:信道的最大数据速率,以bps来计量 数字速率是数字传输过程中采用物理信道模拟带宽所 能获得的最终结果

问题2:(物理介质)信道的最大传输速率是多少?
问题2:(物理介质)信道的最大传输速率是多少?

2.1.2信道的最大传输速率 Nyquist定理(无噪信道 ) 如果一个任意的信号通过带宽为H的低通滤波 器,那么每秒采样2H次就能完整地重现通过该 滤波器的信号 信号包含V个离散等级,则最大数据传输率 =2H log2V (b/s) Shannon定理(有噪信道) 对任何带宽为HHz,信噪比为S/N的信道,最 大数据传输速率=HIog2(1+S/N)(b/s) S/N用分贝(dB)描述:1Olg(s/N)dB
2.1.2信道的最大传输速率 ◼ Nyquist 定理(无噪信道) ◼ 如果一个任意的信号通过带宽为H的低通滤波 器,那么每秒采样2H次就能完整地重现通过该 滤波器的信号 ◼ 信号包含V个离散等级,则最大数据传输率 =2H log2V(b/s) ◼ Shannon定理(有噪信道) ◼ 对任何带宽为H Hz,信噪比为S/N的信道,最 大数据传输速率=H log2(1+S/N)(b/s) S/N用分贝(dB)描述:10lg(S/N) dB
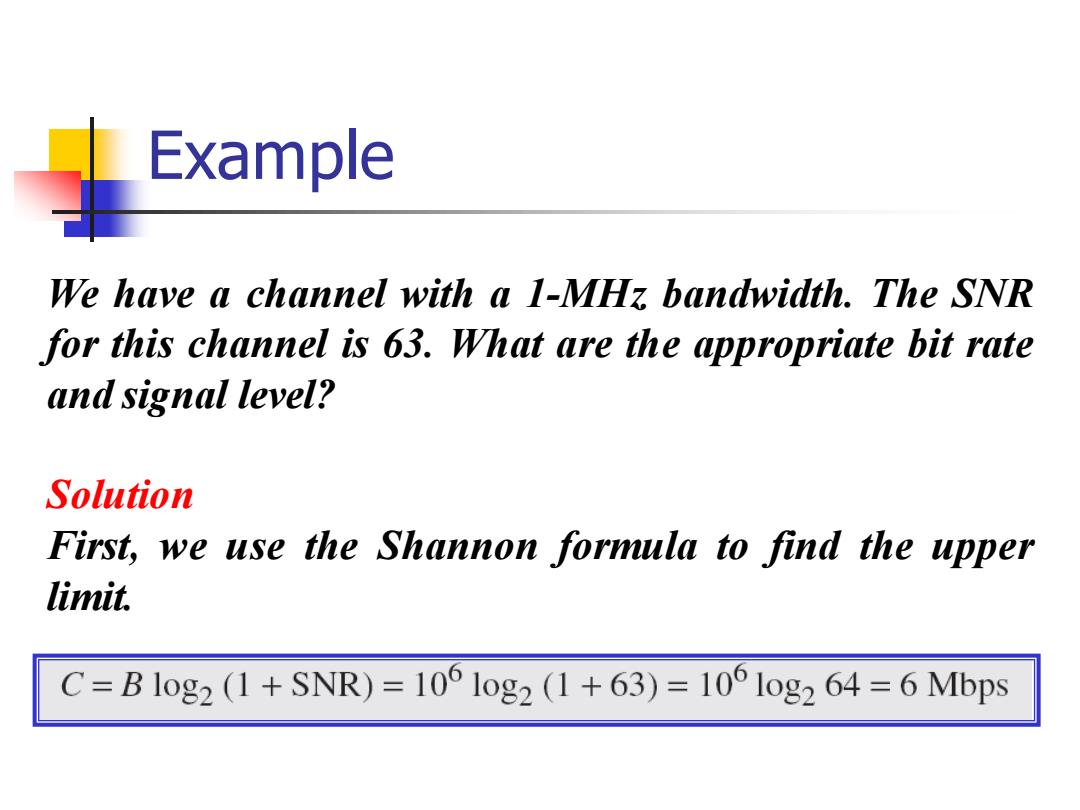
Example We have a channel with a 1-MHz bandwidth.The SNR for this channel is 63.What are the appropriate bit rate and signal level? Solution First,we use the Shannon formula to find the upper limit. C=B1og2(1+SNR)=1061og2(1+63)=101og264=6Mbps
Example We have a channel with a 1-MHz bandwidth. The SNR for this channel is 63. What are the appropriate bit rate and signal level? Solution First, we use the Shannon formula to find the upper limit

Example(continue) The Shannon formula gives us 6 Mbps,the upper limit. For better performance we choose something lower,4 Mbps,for example.Then we use the Nyquist formula to find the number of signal levels. 4Mbps=2×1MHz×log2 L=4 Shannon定理给出了信道容量的理论上限, 而Nyquist定理给出了信号离散等级的数量
Example (continue) The Shannon formula gives us 6 Mbps, the upper limit. For better performance we choose something lower, 4 Mbps, for example. Then we use the Nyquist formula to find the number of signal levels. Shannon定理给出了信道容量的理论上限, 而Nyquist定理给出了信号离散等级的数量