
Scope of Optimization o Optimal design manufacturing o Inverse Problems:output known,find input. oParameter optimization for optimal performance o System modeling o Planning oOptimal control o Forecasting and prediction o Data mining(classification,clustering,pattern recognition) o Machine learning o Bioinformatics CLGO System Engineering by J.J.Gao 6
+ - System Engineering by J. J. Gao 6 Scope of Optimization

Solving Optimization Problem General Optimization Problem Very difficult to solve Methods involve some compromise,e.g.,very long computational time,or not always finding the solution ■ Exceptions:Certain problem classes Least-Squares problem Linear programming problem Convex Optimizitioin problem CLGO System Engineering by J.J.Gao 7
+ - General Optimization Problem Very difficult to solve Methods involve some compromise, e.g., very long computational time, or not always finding the solution Exceptions: Certain problem classes Least-Squares problem Linear programming problem Convex Optimizitioin problem System Engineering by J. J. Gao 7 Solving Optimization Problem

Least Squares minimize 6()=‖Ax-bl3=∑1(ax-b)2. Here ARx (with k>n),af are the rows of A,and the vector 2 R"is the optimization variable. analytical solution:*=(ATA)-1ATb The basis of regression analysis,optimal design,many parameter estimation and data fitting methods. Reliable and efficient algorithms and software Computational time proportional to n2:(A E R*x") System Engineering by J.J.Gao CLGO 8
+ - System Engineering by J. J. Gao 8 Least Squares • The basis of regression analysis, optimal design, many parameter estimation and data fitting methods. • Reliable and efficient algorithms and software • Computational time proportional to
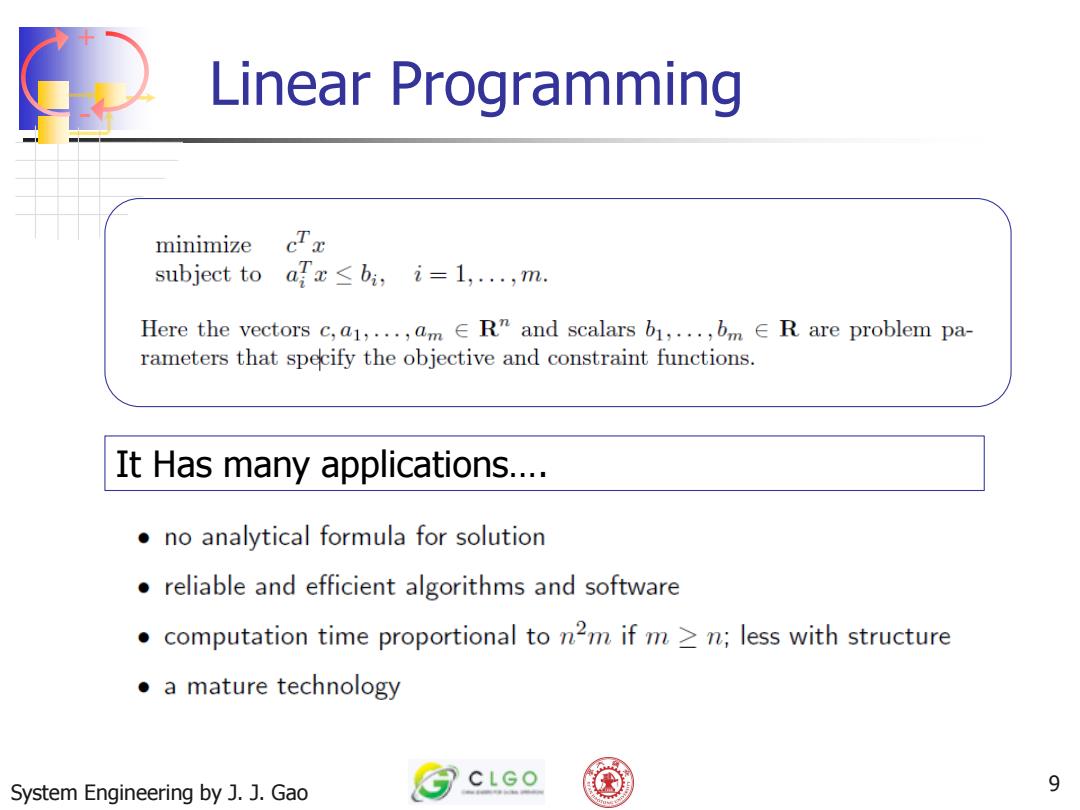
Linear Programming minimize cTa subject to afa s bi,i=1,...,m. Here the vectors c,a1,...,am E R"and scalars b1,...,bm E R are problem pa- rameters that specify the objective and constraint functions. It Has many applications.... no analytical formula for solution reliable and efficient algorithms and software computation time proportional to n2m if m =n;less with structure ●a mature technology CLGO System Engineering by J.J.Gao 9
+ - System Engineering by J. J. Gao 9 Linear Programming It Has many applications…
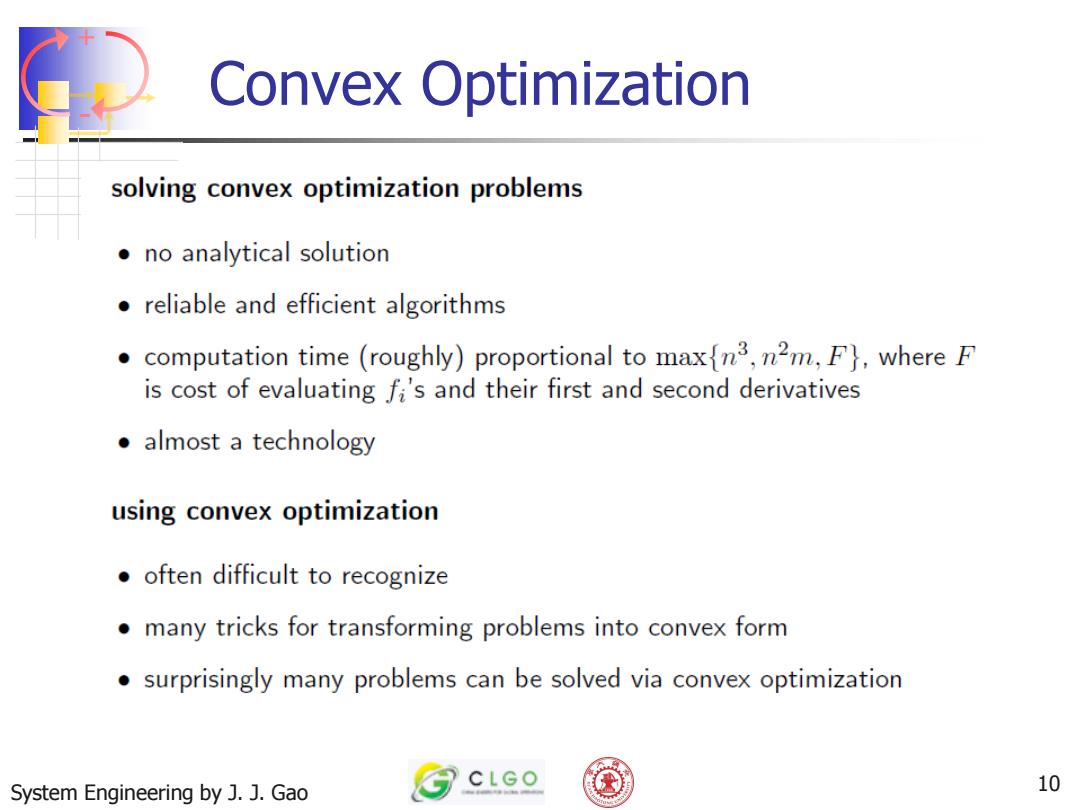
Convex Optimization solving convex optimization problems no analytical solution reliable and efficient algorithms computation time (roughly)proportional to max{n3,n2m,F},where F is cost of evaluating fi's and their first and second derivatives ●almost a technology using convex optimization often difficult to recognize many tricks for transforming problems into convex form surprisingly many problems can be solved via convex optimization CLGO System Engineering by J.J.Gao 10
+ - System Engineering by J. J. Gao 10 Convex Optimization