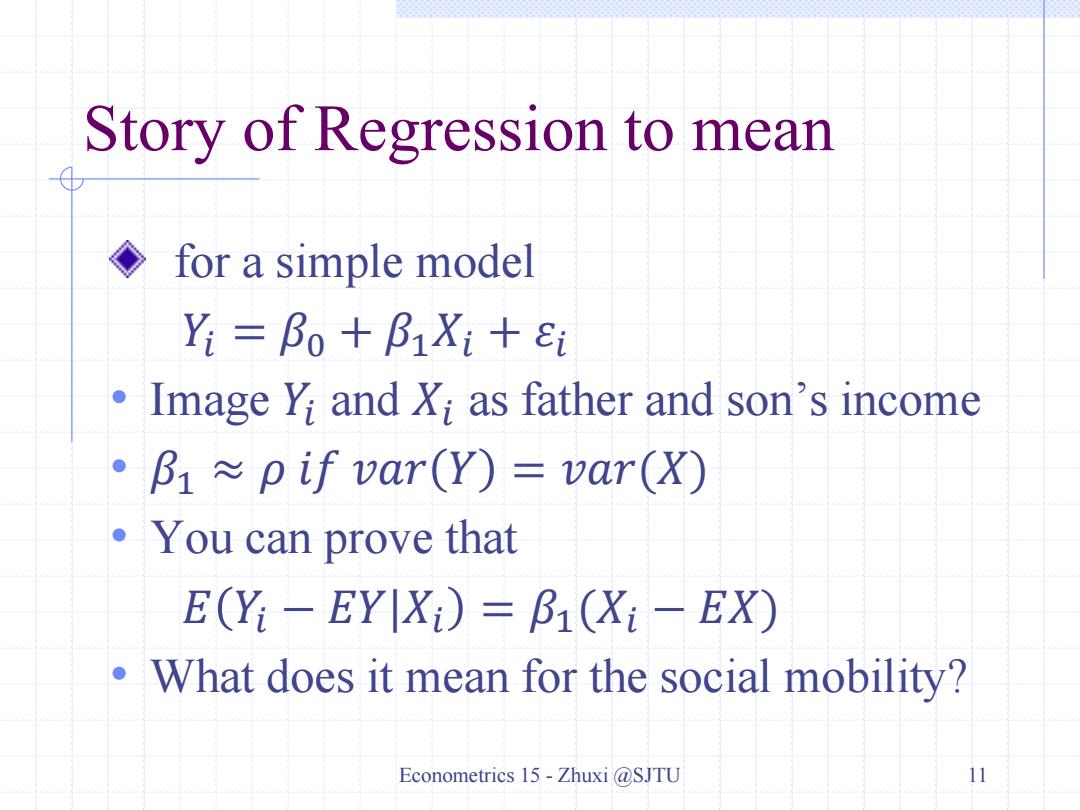
Story of Regression to mean ◆for a simple model Yi Bo BiXi+i Image Yi and Xi as father and son's income ·B1≈p if var(Y)=var(X) .You can prove that EYi-EY Xi)=B1(Xi-EX) What does it mean for the social mobility? Econometrics 15-Zhuxi @SJTU 11
Story of Regression to mean for a simple model 𝑌𝑖 = 𝛽0 + 𝛽1𝑋𝑖 + 𝜀𝑖 • Image 𝑌𝑖 and 𝑋𝑖 as father and son’s income • 𝛽1 ≈ 𝜌 𝑖𝑓 𝑣𝑎𝑟 𝑌 = 𝑣𝑎𝑟(𝑋) • You can prove that 𝐸 𝑌𝑖 − 𝐸𝑌|𝑋𝑖 = 𝛽1(𝑋𝑖 − 𝐸𝑋) • What does it mean for the social mobility? Econometrics 15 - Zhuxi @SJTU 11

Properties of MLR Residuals From above OLS method,we have X'(Y-XB)=0 thus=0 that is ∑x立=0 if X contains constant,we have ∑4=0 (2) Econometrics 15-Zhuxi @SJTU 12
Econometrics 15 - Zhuxi @SJTU 12 Properties of MLR Residuals From above OLS method, we have ˆ 0 thus 0. ˆ that is ˆ 0 (1) if contains constant, we have ˆ 0 (2) ik i i i i X Y X X u x u X u b

A "Partialling Out"Interpretation Consider the case where k =2,i.e. =B+月x+px2,then B=(∑y)/∑, where are the residuals from 元1=Yo+72x2,S01=X1:-元 Econometrics 15-Zhuxi @SJTU 13
Econometrics 15 - Zhuxi @SJTU 13 A “Partialling Out” Interpretation 0 1 1 2 2 2 1 1 1 1 1 0 2 2 1 1 1 Consider the case where 2, i.e. ˆ ˆ ˆ ˆ , then ˆ ˆ ˆ , where are the residuals from ˆ ˆ ˆ ˆ , so ˆ ˆ i i i i i i i k y x x r y r r x x r x x b b b b
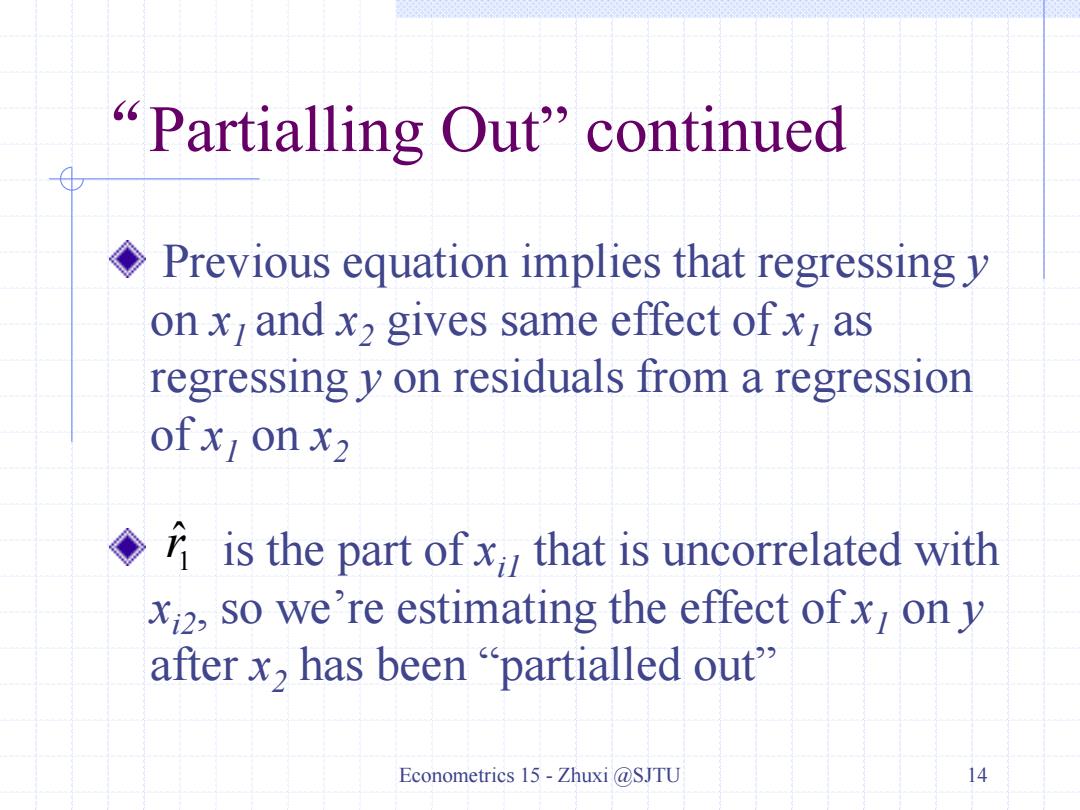
Partialling Out"continued Previous equation implies that regressingy onx and x2 gives same effect of x,as regressing y on residuals from a regression of x on x2 is the part ofx that is uncorrelated with x2,so we're estimating the effect of x,ony after x,has been "partialled out" Econometrics 15-Zhuxi @SJTU 14
Econometrics 15 - Zhuxi @SJTU 14 “Partialling Out” continued Previous equation implies that regressing y on x1 and x2 gives same effect of x1 as regressing y on residuals from a regression of x1 on x2 is the part of xi1 that is uncorrelated with xi2, so we’re estimating the effect of x1 on y after x2 has been “partialled out” 1 r ˆ

Simple vs Multiple Reg Estimate Compare the simple regression=B。+阝,x with the multiple regression+ thus=+,where is the slope coefficient(x2 onx) Generally,B unless: (1)B=0i.e.no partial effect ofx2. (2)=0i.e.and x2 are uncorrelated in the sample. Econometrics 15-Zhuxi @SJTU 15
Econometrics 15 - Zhuxi @SJTU 15 Simple vs Multiple Reg Estimate 0 1 1 0 1 1 2 2 1 1 2 1 1 2 1 1 1 2 Compare the simple regression ˆ ˆ ˆ with the multiple regression ˆ ˆ ˆ thus , where is the slope coefficient( on ) Generally, unless: ˆ ˆ (1) 0 i.e. no y x y x x x x b b b b b b b b b b b 2 1 1 2 partial effect of . (2) 0 i.e. and are uncorrelated in the sample. x x x