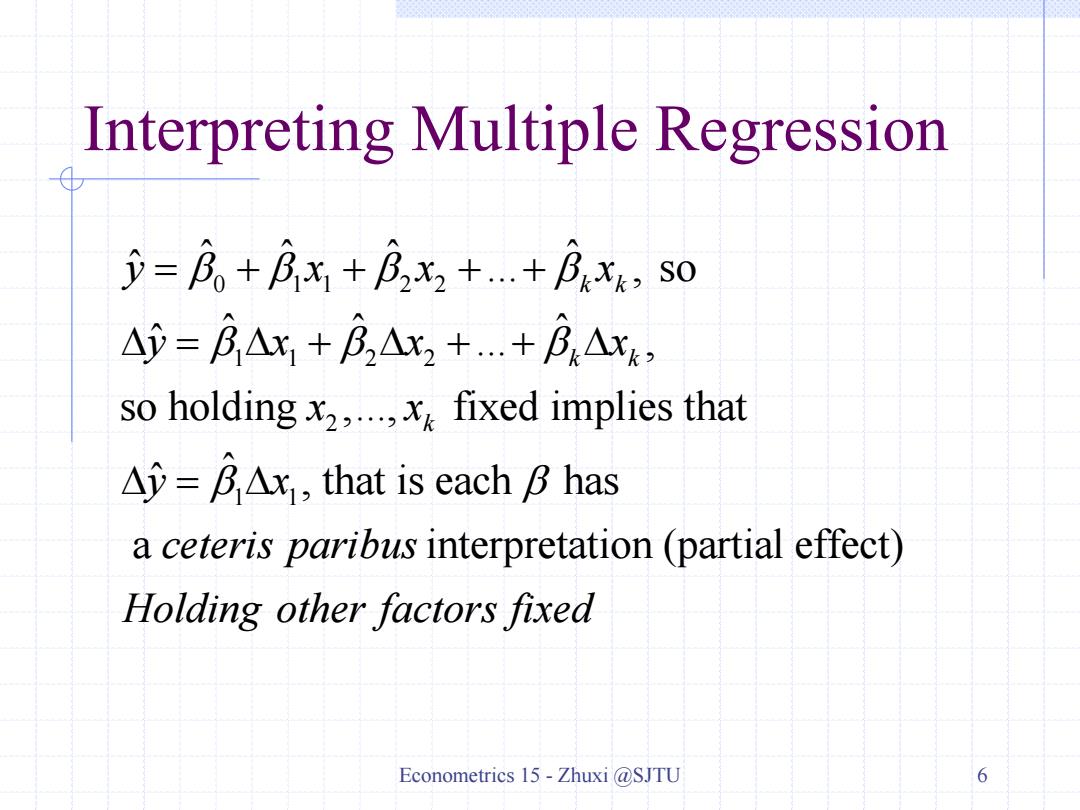
Interpreting Multiple Regression Bo++Bxx So △少=B,△x+B2△x2+.+B△x, so holdingx2,fixed implies that △y=阝,△x,that is each B has a ceteris paribus interpretation (partial effect) Holding other factors fixed Econometrics 15-Zhuxi @SJTU 6
Econometrics 15 - Zhuxi @SJTU 6 Interpreting Multiple Regression 0 1 1 2 2 1 1 2 2 2 1 1 ˆ ˆ ˆ ˆ ˆ ... , so ˆ ˆ ˆ ˆ ... , so holding ,..., fixed implies that ˆ ˆ , that is each has a interpretation (partial effect) k k k k k y x x x y x x x x x y x ceteris paribus Holding other factor b b b b b b b b b s fixed
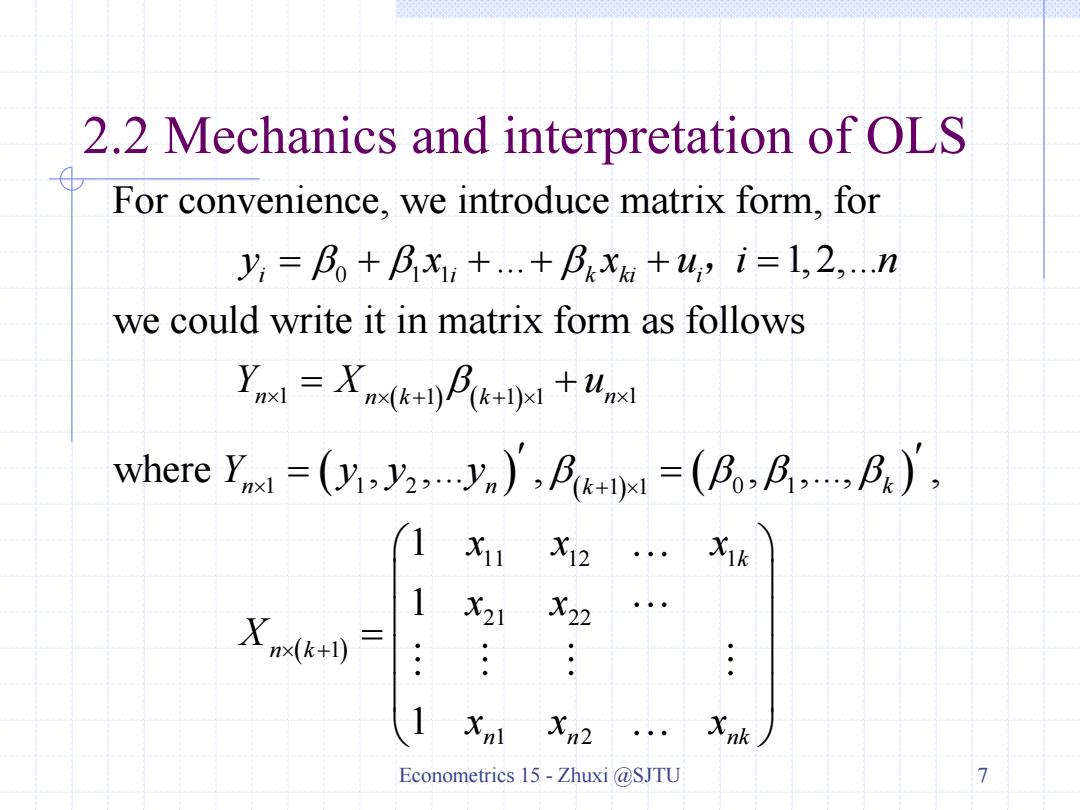
2.2 Mechanics and interpretation of OLS For convenience,we introduce matrix form,for =Bo+Bx.+Bx+u i=1,2....n we could write it in matrix form as follows Yx)B()np where Y=.)B=(BoBB) 1x1 X12..X 1 Xm(k+i) X21 X22 1 Xnl Xn2 Xnk Econometrics 15-Zhuxi @SJTU 7
Econometrics 15 - Zhuxi @SJTU 7 2.2 Mechanics and interpretation of OLS 0 1 1 1 1 1 1 1 1 1 2 0 1 1 1 1 For convenience, we introduce matrix form, for ... 1,2,... we could write it in matrix form as follows where , ,... , , ,..., , i i k ki i n n n k k n n k k n k y x x u i n Y X u Y y y y X b b b b b b b b , 11 12 1 21 22 1 2 1 1 1 k n n nk x x x x x x x x

Obtaining the OLS Estimates(ctd) the method of OLS(minimize SSR): min之td=∑(y-b。-bx:-b,x--bea)) bo,b,… il i- or in matrix form min SSR(b)=(Y-Xb)(Y-X6) Econometrics 15-Zhuxi @SJTU 8
Econometrics 15 - Zhuxi @SJTU 8 Obtaining the OLS Estimates(ctd) 0 1 2 2 0 1 1 2 2 , ,... the method of OLS (minimize SSR): min ... ˆ or in matrix form min n n i i i i k ki b b i=1 i=1 b u y b b x b x b x SSR b Y Xb Y Xb
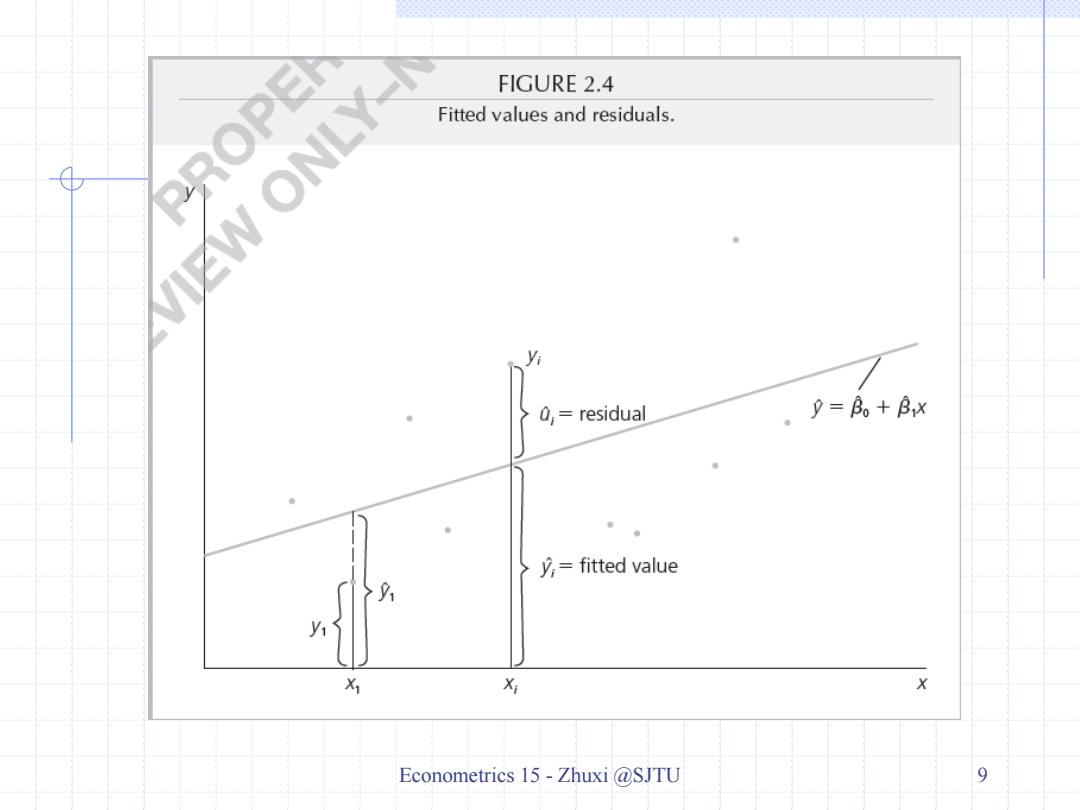
PROPEN FIGURE 2.4 W∠V ONLY- Fitted values and residuals. y 0,=residual ,9=B。+Bx y,=fitted value X X Econometrics 15-Zhuxi @SJTU 9
Econometrics 15 - Zhuxi @SJTU 9
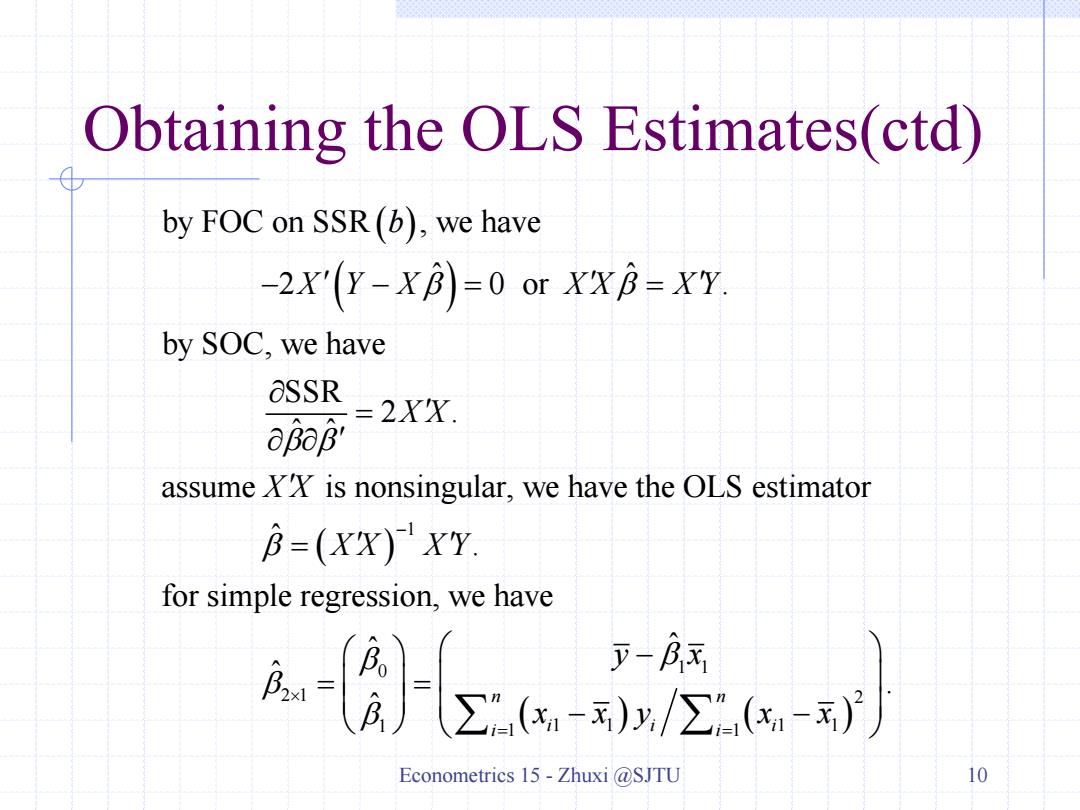
Obtaining the OLS Estimates(ctd) by FOC on SSR(b),we have -2x(Y-XB)=0 or XXB=XY. by SOC,we have OSSR =2XX. aBaB assume XX is nonsingular,we have the OLS estimator B=(XX)XY. for simple regression,we have -Bx ∑(,-)y/∑(-)) Econometrics 15-Zhuxi @SJTU 10
Econometrics 15 - Zhuxi @SJTU 10 Obtaining the OLS Estimates(ctd) 1 0 2 1 by FOC on SSR , we have ˆ ˆ 2 0 or . by SOC, we have SSR 2 . ˆ ˆ assume is nonsingular, we have the OLS estimator ˆ . for simple regression, we have ˆ ˆ ˆ b X Y X X X X Y X X X X X X X Y b b b b b b b b 1 1 2 1 1 1 1 1 1 1 ˆ . n n i i i i i y x x x y x x b