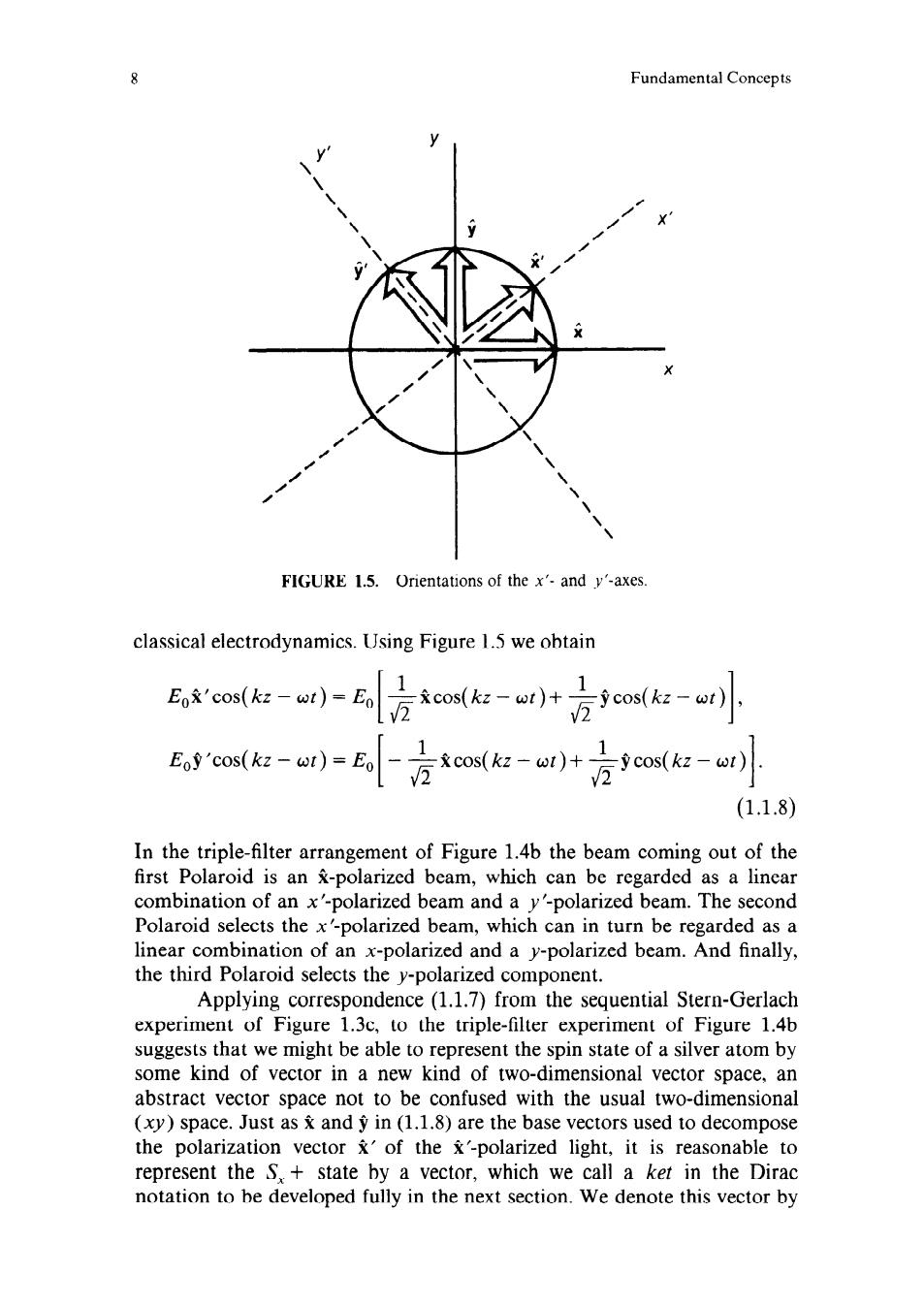
8 Fundamental Concepts FIGURE 1.5.Orientations of the x'and y'-axes classical electrodynamics.Using Figure 1.5 we obtain E'con(ka-ul)-2oak-or)+方 cos(k:-ur) Eoy'cos(kz-wt)=Eo 2 cos(k-r)+ 方cos(红-r) (1.1.8) In the triple-filter arrangement of Figure 1.4b the beam coming out of the first Polaroid is an &-polarized beam,which can be regarded as a linear combination of an x'-polarized beam and a y'-polarized beam.The second Polaroid selects the -polariz am, which can in tu be regarded linear combination of an x-polarized and a y-polarized beam.And finally, the third Polaroid selects the y-polarized component. Applying correspondence (1.1.7)from the sequential Stern-Gerlach experiment of Figure 1.3c.to the triple-filter experiment of Figure 1.4b suggests that we might be able to represent the spin state of a silver atom by some kind of vector in a new kind of two-dimensional vector space,an abstract vector space not to be confused with the usual two-dimensional (xy)space.Just as andy in(1.1.8)are the base vectors used to decompose the polarization vector of the &'-polarized light it re asonable to represent the S.+state by a vector,which we call a ker in the Dirac notation to be developed fully in the next section.We denote this vector by

1.1.The Stern Gerlach Experiment 9 S:+)and write it as a linear combination of two base vectors,S.;+) and S.;->,which correspond to the S.+and the S.-states,respectively. So we may conjecture +)21 心:+)+ :) (1.1.9a) S:-)2-1 心:+)+ (1.1.9b) in analogy with (1.1.8).Later we will show how to obtain these expressions using the general formalism of quantum mechanics. Thus the unblocked component coming out of the second (SG) apparatus of Figure 1.3c is to be regarded asa superposition S:-in the nse of (1.1.9a).It is for this reason that two components emerge from the third(SG)apparatus. The next question of immediate concern is,How are we going to represent arguments sest that if we observe anS.± beam going in the n and ject it to an apparatus the resulting situation will be very similar to the case where an S.+beam going in the y-direction is subjected to an SG&apparatus.The kets for S should then be regarded as a linear combination of S.;+,but it appears from (1.1.9)that we have aready used up the availabe possibilities in writing).How can our vector space formalism distinguishS. states from S states? An analogy with polarized light again rescues us here.This time we polarized bea of light,which can be obtained by letting a linearly polarized light pas through a quarter-wave plate.When we pass such a circularly polarized light through an x-filter or a y-filter,we again obtain either an x-polarized beam or a y-polarized beam of equal intensity.Yet everybody knows that the circularly polarized light is totally different from the 45 nearly polarized (x'-polarize or y-polarized)light Mathematically,how do we represent a circularly polarized light?A right circularly polarized light is nothing more than a linear combination of an x-polarized light and a y-polarized light,where the oscillation of the electric field for the y-polarized component is 90 out of phase with that of the x-polarized component: E=Eo 万cosz-or)+ 万ok如-w+ .(1.1.10) It is more elegant to use complex notation by introducing as follows: Re(e)=E/Eo (1.1.11) no unanimity in the definition of right versus left circularly eratur

10 Fundamental Concepts For a right circularly polarized light,we can then write e- (1.1.12) where we have used i=e'/2. We can make the following analogy with the spin states of silver atoms: S.atom right circularly polarized beam (1.1.13) S.-atomleft circularly polarized beam. Applying this analogy to (1.1.12),we see that if we are allowed to make the coefficients preceding base kets complex,there is no difficulty in accommo- dating the S+atoms in our vector space formalism 1 ISv;±〉= :+)± lS;-〉, (1.1.14) which are obviously different from (1.1.9).We thus see that the two-dimen- sional vector space needed to des cribe the spin states of silver atoms mus be a complex vector space;an arbitrary vector in the vector space is written as a linear combination of the base vectors S.;+)with,in general,complex coefficients.The fact that the necessity of complex numbers is already apparent in such an elementary example e is rather remarkable. The reade r must have noted by this time that we have deliberately avoided talking about photons.In other words,we have completcly ignored the quantum aspect of light;nowhere did we mention the polarization states of individual photons.The analogy we worked out is between kets in an abstract vector space that de states of individual atoms with the polarization vectors of the classical electromagnetic field.Actually we could have made the analogy even more vivid by introducing the photon concept and talking about the probability of finding a circularly polarized photon in a linea arly polarized sta ate.and so forth;h tha t is no needed here.Without doing so.we have already accomplished the main goal of this section:to introduce the idea that quantum-mechanical states are to be represented by vectors in an abstract complex vector space. 1.2.KETS,BRAS,AND OPERATORS In the preceding section we showed how analyses of the Stern-Gerlach experiment lead us to consider a complex vector space.In this and the *The reader who is inte polanzaiping rested ir the hasic hanics thr a careful study of pho illuminating

1.2.Kets,Bras,and Operators 11 following section we formulate the basic mathematics of vector spaces as used in quantum mechanics.Our notation throughout this book is the bra and ket notation developed by P.A.M.Dirac.The theory of linear vector spaces had. of course,been known to mathematicians prior to the birth of quantum mechanics,but Dirac's way of introducing vector spaces has many advantages,especially from the physicist's point of view. Ket Space We consider a complex vector space whose dimensionality is specified according to the nature of a physical system under consideration.In Stern-Gerlach-type experiments where the only quantum-mechanical de- gree of freedo s the spin of an atom,the dimens onality is determined by the number of alternative paths the atoms can follow when subjected to a SG apparatus;in the case of the silver atoms of the previous section,the dimensionality is just two,corresponding to the two possible values S.can assume.*Later, in Se ction 1.6, we onsider the case of continuc spectra -for example,the position (coordinate)or momentum of a particl where the number of alternatives is nondenumerably infinite,in which case the vector space in question is known as a Hilbert space after D. Hilbert,who studied vec es in infinite dime nsions In quantum mechanics a physical state,for example,a silver atom with a definite spin orientation,is represented by a state vector in a complex vector space.Following Dirac,we call such a vector a ket and denote it by This state ket is postulated to contain about the ysical state;everything we a allowed to ask about the state is containe in the ket.Two kets can be added: la)+18>=ly>. (1.2.1) The sum is just another ket.If we multiplyby a complex number the resulting product cla)is another ket.The number c can stand on the left or on the right of a ket;it makes no difference: cla〉=lac (1.2.2) In the particular case where c is zero,the resulting ket is said to be a null ket. One of the physics postulates is that la)and cla),with c+0, represent the same physical state.In ther words,only ector space is of ignifica nce.Mathematicians may prefer to say that we are here dealing with rays rather than vectors. ◆For many physical systems the ension of the ensions

12 Fundamental Concepts An observable,such as momentum and spin components,can be represented by an operator,such as A,in the vector space in question.Quite generally,an operator acts on a ket from the left, A.(la))=Ala). (1.2.3) which is yet another ket.There will be more on multiplication operations later. In general,Ala)is not a constant times a).However,there are particular kets of importance,known as eigenkets of operator A,denoted by la),la"),a"),. (1.2.4) with the property Aa)=a1a)、a")=a"1a"), (1.2.5) where a',a", are just numbers.Notice that applying A o an cigenket just reproduces the same ket apart froma.The setof numbers {a,a".a"....),more compactly denoted by {a'),is called the set of eigenvalues of operator A.When it becomes necessary to order eigenvalues in a specific manner.(a,a.a()....)may be used in place of (a',a",a' The physical state corresponding to an eigenket is called an eigen- state.In the simplest case of spin systems,the eigenvalue-eigenket relation (1.2.5)is expressed as S.1S:+)=2S+ SlS;-〉=-51S;-),(1.2.6) where .:)are eigenkets of operator S.with eigenvalues+h/2.Here we could have used just h/2)for IS.;+in conformity with the notation la'), where an eigenket is labeled by its eigenvalue,but the notation S.:+). already used in the previous section,is more convenient here because we also consider eigenkets of S: SS:t)=±S:t). (1.2.7) We remarked earlier that the dimensionality of the vector space is determined by the number of alternatives in Stern-Gerlach-type experi- ments.More formally.we are concerned with an N-dimensional vecto space spanned by the N eigenkets of observable Any arbitrary ket can be written as la)=>cla). (1.2.8) with a',a",...up to a(N),where c is a complex cocfficicnt.The question of the uniqueness of such an expansion will be postponed until we prove th orthogonality of eigenkets