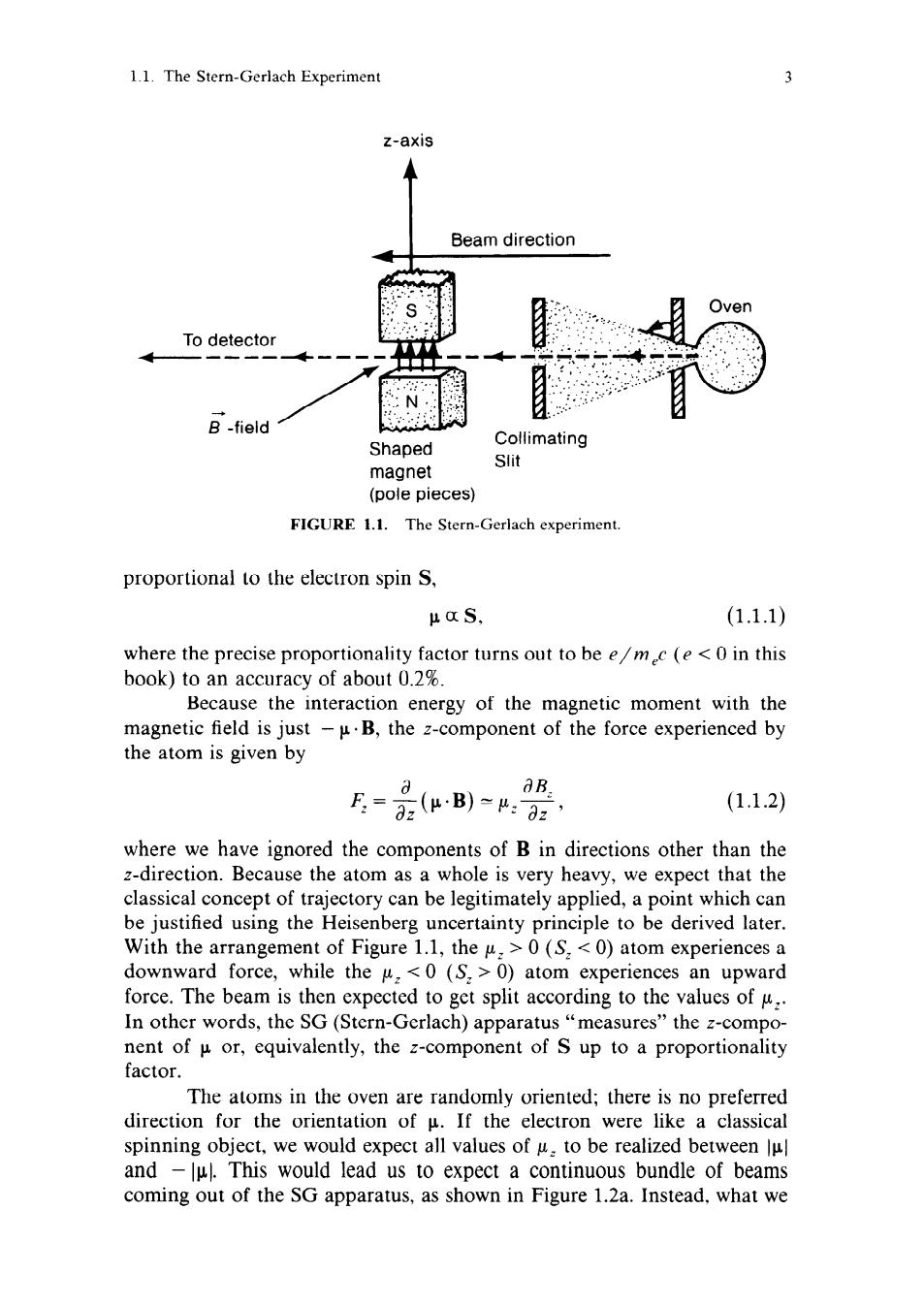
1.1.The Stern-Gerlach Experiment Z-axis Beam direction Over To detector B-field Shaped limating Sli magnet (pole pieces) FIGURE 1.1.The Stern-Gerlach experiment proportional to the electron spin S, μaS (1.1.1) where the precise proportionality factor turns out to be e/m c(e <0 in this book)to an accuracy of about 0.2%. the interaction energy of the magnetic moment with the magnetic field is just-u.B,the z-component of the force experienced by the atom is given by aB. F=2(uB):a2 (1.1.2) where we have ignored the components of B in directions other than the z-direction.Because the atom as a whole is very heavy,we expect that the classical concept of trajectory can be legitimately applied,a point which can be justified using the Heisenberg uncertainty p derived late With the arrangement of Figure 1.1,the(S)atom experiencesa downward force,while the u.<0(S.>0)atom experiences an upward force.The beam is then expected to get split according to the values of u. In other words,the SG(Stern-Gerlach)apparatus"measures"the z-comp nent of or,equivalently,the z-component of S up to a proportionality factor. The atoms in the oven are randomly oriented;there is no preferred direction for the orientation of u.If the electron were like a classical spinning object we wo expect all values oftobe realized between and-lul.This would lead us to expect a continuous bundle of beams coming out of the SG apparatus,as shown in Figure 1.2a.Instead,what we
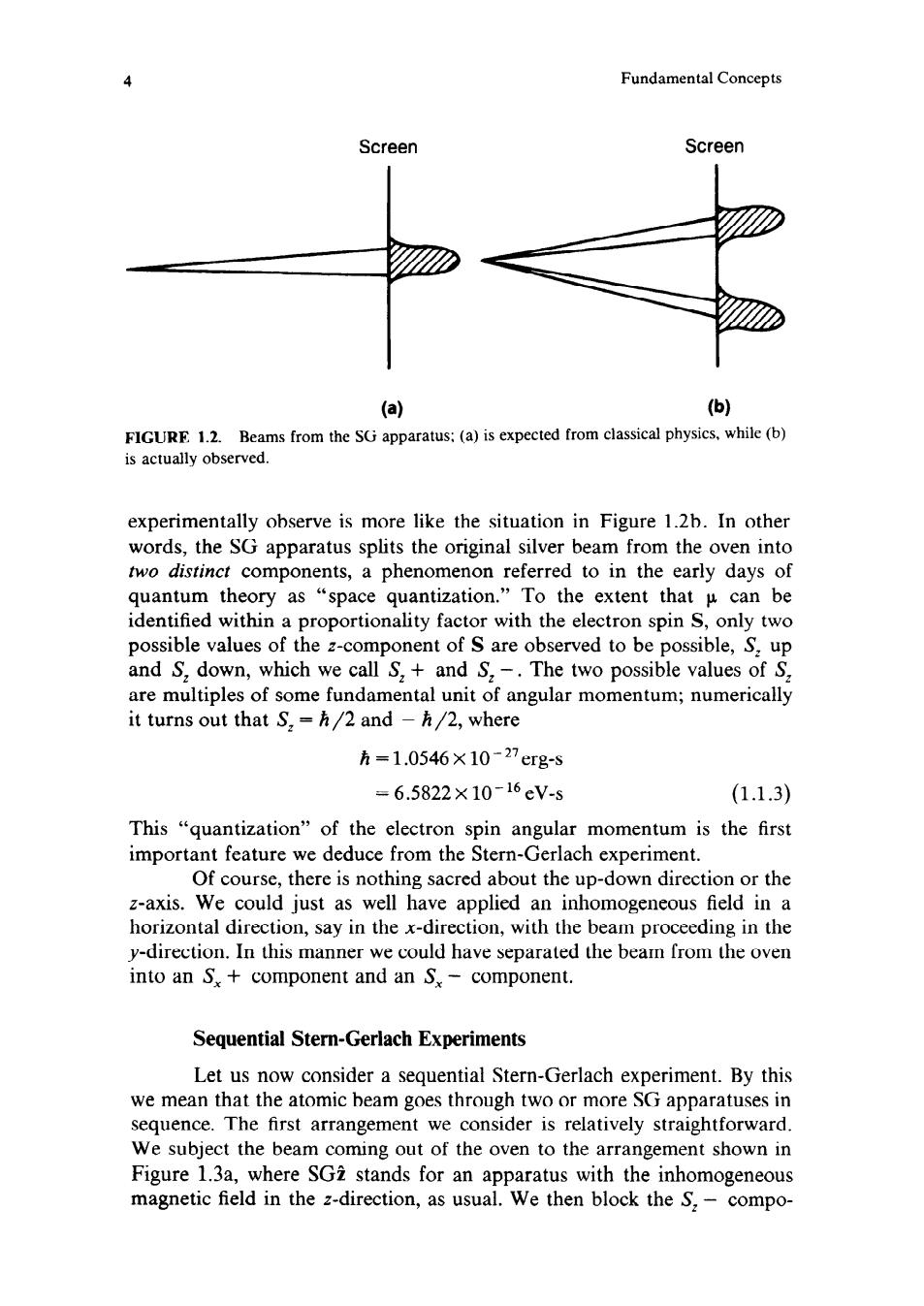
Fundamental Concepts Screen (a) (b) hCafbeasomtkXawansaaeaeaomdstpask experimentally observe is more like the situation in Figure 1.2b.In other words,the SG apparatus splits the original silver beam from the oven into two distinct components,a phend referred to in the early days f quantum theory as“space quantization.”To the extent thatμcan be identified within a proportionality factor with the electron spin S,only two possible values of the z-component of S are observed to be possible,S.up and,which we call+and S..The two possible values of are multiples of some fundamental unit of angular momentum;numerically it turns out that S.h/2 and-h/2,where 方=1.0546×10-27erg-s =6.5822×10-16cV-s (1.1.3) This "quantization"of the electron spin angular momentum is the first important feature we deduce from the Stern-Gerlach experiment. Of course,there is nothing sacred about the up-down direction or the e beam proceeding in the y-direction.In this manner we could have separated the beam from the oven into an S,component and an S,-component. Sequential Stern-Gerlach Experiments Let us now consider a sequential Stern-Gerlach experiment.By this we mean that the atomic beam goes through two or more SG apparatuses in we consider is relatively straightforward Figure 1.3a,where SG2 stands for an apparatus with the inhomogeneous magnetic field in the z-direction,as usual.We then block the S.-compo-

1.1.The Stern-Gerlach Experiment S.+comp. .S.+comp SG2 No S,-comp S2-comp Sx+beam. S.+bean Dven -S,-bean S:-beam. ⊙ S:+beam. S.+beam. sG☑ SG2 S,-beam. S.-beam. FIGGURE 1.3.Sequential Stern-(ierlach experiments. paratus and let the remaining S.+ nent coming out or the aaratus.This time there is only one beam component coming out of the second apparatus-just the S.+ component.This is perhaps not so surprising;after all if the atom spins are up,they are expected to remain so,short of any external ficld that rotates the spins between the first and the second SGi apparatuses A little more interesting is the arrangement shown in Figure 1.3b Here the first SG apparatus is the same as before but the second one(SG&) has an inhomogeneous magnetic field in the x-direction.The S.+beam that enters the second apparatus(SG&)is now split into two comp nents,an +component and anS-component,with cqual intensities.How can we explain this?Does it mean that 50%of the atoms in the S.+beam coming out of the first apparatus(SG)are made up of atoms characterized by both S.and S.+,while the remaining 50%have both S.+and S.-? It turns out that such a picture e runs into difficulty,as will be shown bel We now consider a third step,the arrangement shown in Figure 1.3(c),which most dramatically illustrates the peculiarities of quantum- mechanical systems.This time we add to the arrangement of Figure 1.3b imentally that beams are seen to have both an S.+component and an S.-component. This is a complete surprise because after the atoms emerged from the first

6 Fundamental Concepts apparatus,we made sure that the S.-component was completely blocked How is it possible that the S.-component which,we thought,we eliminated earlier reappears?The model in which the atoms entering the third appara- evsualized to have both S.and S.+is clearly unsatisfactory. This example is often used to illustrate that in we cannot determine both S.and S,simultancously.Morc preciscly,we can say that the selection of the S,+beam by the second apparatus (SG&) completely destroys any previous information about S.. It is amusing to compare this situation with that of a spinning top in classical mechanics,where the angular momentum L=Iw (1.1.4) can be measured by determining the components of the angular-velocity aning in which direction computable if we know the mass density and the geometric shape of the spinning top,so there is no difficulty in specifying both L,and L,in this classical situation It is to be clearly understood that the limitation we have ncountered in determining S.and S,is not due to the incompetence of the experi mentalist.By improving the experimental techniques we cannot make the S.-component out of the third apparatus in Figure 1.3c disappear.The uliarities of quantum mechanics imposed upon us by the experiment Analogy with Polarization of Light Because this situation looks so novel,some analogy with a familiar classical situation may be helpful here.To this end we now digress to consider the polarization of light waves. Consider a monochromatic light way polarized)light pa with a ization vector in the x-direction,which we call for short an x-polarized light,has a space-time dependent electric field oscillating in the x-direction E=Eo cos(kz-wt). (1.1.5) Likewise,we may consider a y-polarized light,also propagating in the z-direction, E=Eo cos(kz wt). (1.1.6) Polarized light beams of type (11.5)or(11.6)ca be obtained by letting an unpolarized light beam go through a Polaroid filter.We call a filter that selects only beams polarized in the x-direction an x-filter.An x-filter,of course,becomes a y-filter when rotated by 90 about the propagation (z)
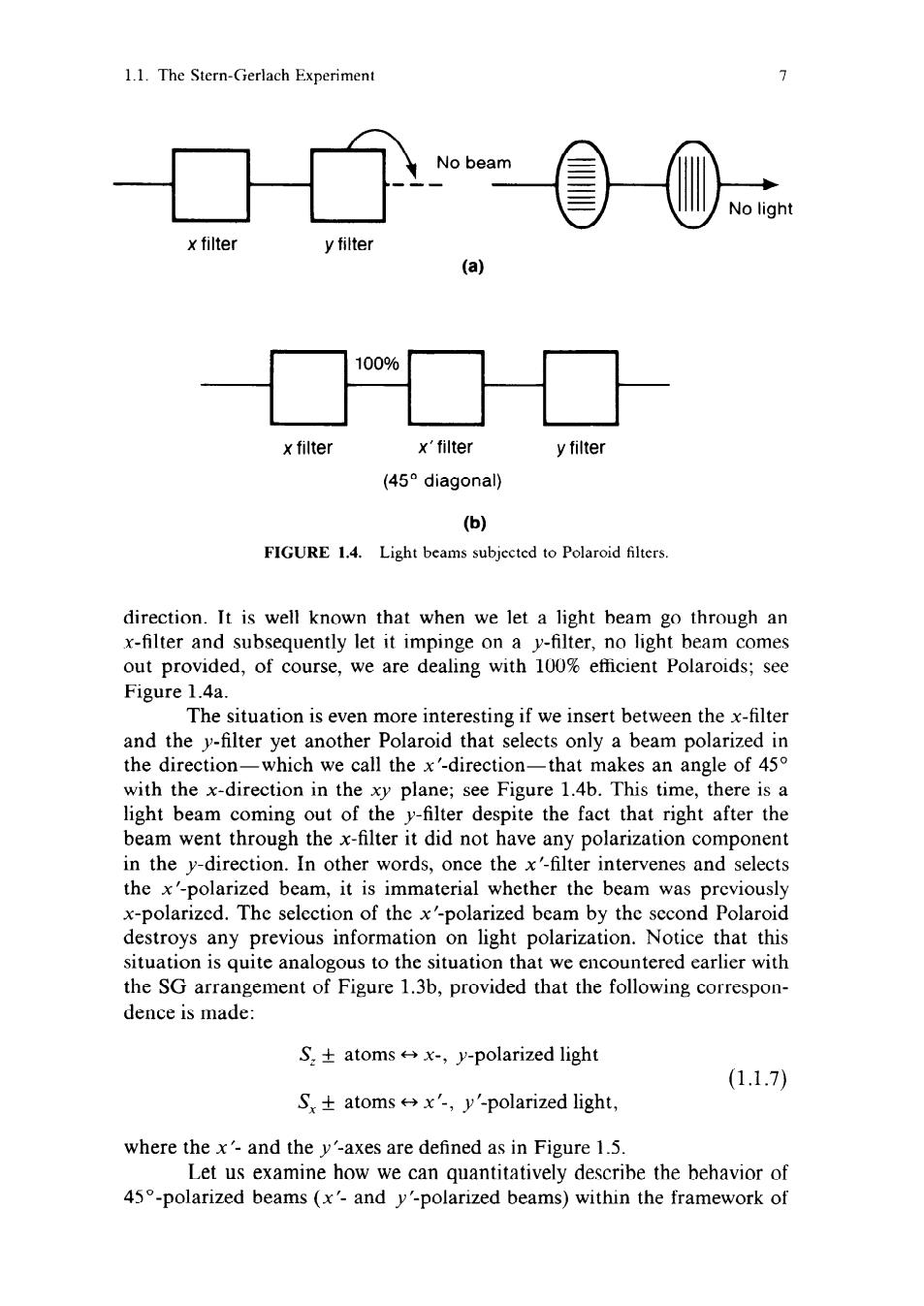
1.1.The Stern-Gerlach Experiment x filter y filte (a) 100% x filter x'filter ytilter (45°diagonal)) 6 FIGURE 1.4.Light beams subiected to Polaroid filters. direction.It is well known that when we let a light beam go through an x-filter and subsequently let it impinge on a y-filter,no light beam comes out provided,of course,we are dealing with 100%efficient Polaroids:see Figure 1.4a. The situation is even more interesting if we insert between the x-filter and the y-filter yet another Polaroid that selects only a beam polarized in the direction-which we call the x'-direction-that makes an angle of 45 with the x-direction in the xy plane;see Figure 1.4b.This time,there is a light beam oming out of the r despite the fact that right after the beam went through the x-filter it did not have any polarization component in the y-direction.In other words,once the x'-filter intervenes and selects the x'-polarized beam,it is immaterial whether the beam was previously am by the ond Polaroid destroys any previous information on light polarization.Notice that this situation is quite analogous to the situation that we encountered earlier with the SG arrangement of Figure 1.3b,provided that the following correspon- dence is made: S.+atomsx-,y-polarized light (1.1.7) S.+atomsx',y'-polarized light where the x'-and the y'-axes are defined as in Figure 1.5. Let us examine how we can quantitatively describe the behavior of 45-polarized beams (x'-and y'-polarized beams)within the framework of