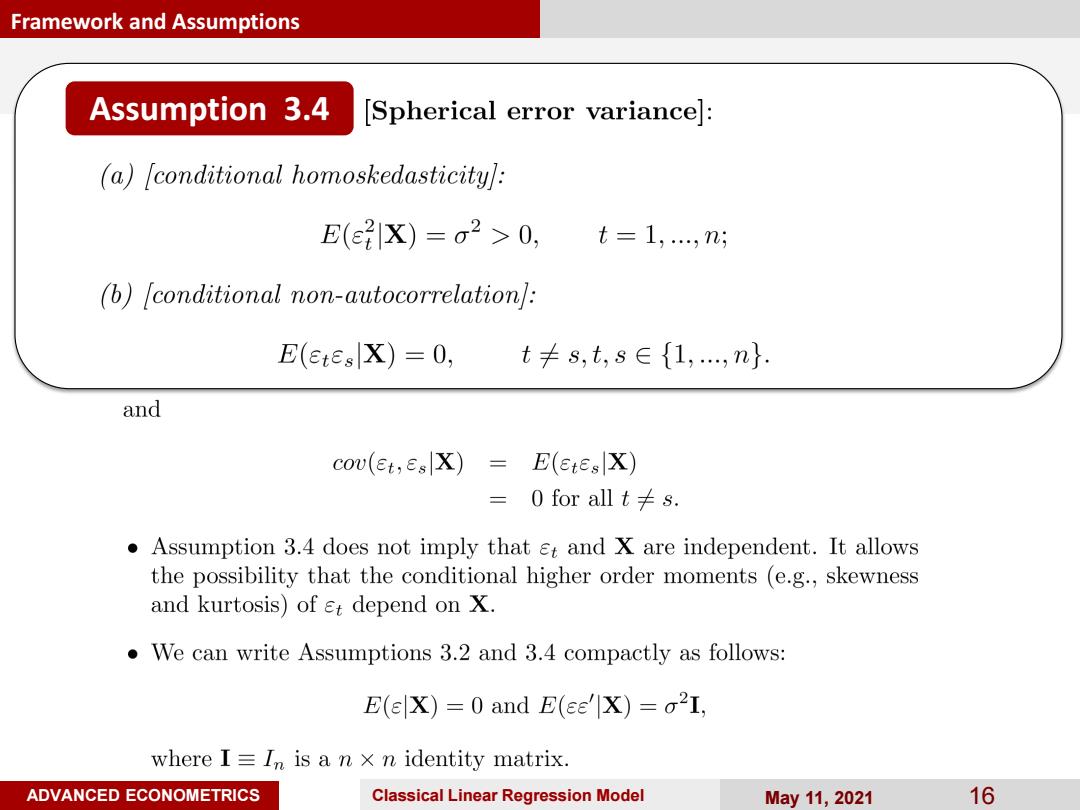
Framework and Assumptions Assumption 3.4 [Spherical error variancel: (a)(conditional homoskedasticity: E(e21X)=σ2>0,t=1,,n (b)conditional non-autocorrelation|: E(Etes X)=0, t≠s,t,s∈{1,,n}. and Cov(Et,Es X)E(EtEs X) =0 for all t≠s. Assumption 3.4 does not imply that et and X are independent.It allows the possibility that the conditional higher order moments (e.g.,skewness and kurtosis)of et depend on X. We can write Assumptions 3.2 and 3.4 compactly as follows: E(eX)=0 and E(ee'X)=o21, where I≡In is a n×n identity matrix. ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 16
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 16 Framework and Assumptions Assumption 3.4

CONTENTS 3.1 Framework and Assumptions 3.2 Ordinary Least Squares (OLS)Estimation 3.3 Goodness of Fit and Model Selection Criteria 3.4 Consistency and Efficiency of OLS 3.5 Sampling Distribution of OLS 3.6 Variance Estimation for OLS 3.7 Hypothesis Testing ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 17
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 17 3.1 Framework and Assumptions 3.2 Ordinary Least Squares (OLS) Estimation 3.3 Goodness of Fit and Model Selection Criteria 3.4 Consistency and Efficiency of OLS 3.5 Sampling Distribution of OLS 3.6 Variance Estimation for OLS 3.7 Hypothesis Testing CONTENTS

Ordinary Least Squares (OLS)Estimation Questions: How to estimate Bo using an observed data set generated from the random sample [Zt)r1,where Zt=(Yt,X)'? Definition 1 [OLS estimator]: Suppose Assumptions 3.1 and 3.3(a)hold.Define the sum of squared resid- uals (SSR)of the linear regression model Yi XB+ut as SSR(B)=(Y-XB)'(Y-XB) ∑(Y-X492. t= Then the OLS estimator B is the solution to 6= arg min SSR(B). B∈RK ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 18
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 18 Ordinary Least Squares (OLS) Estimation Questions: Definition 1

Ordinary Least Squares (OLS)Estimation Theorem 1 Existence of OLS]: Under Assumptions 3.1 and 3.3,the OLS estimator B exists and B=(X'X)X'Y =(xx) ∑ XYi t=1 (容 X:Yi. t=1 ●Proof:Using the formula that for an K×1 vector A and and K×l vector B,the derivative ∂(A'3) ∂3 二A To be continued ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 19
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 19 Ordinary Least Squares (OLS) Estimation Theorem 1 To be continued
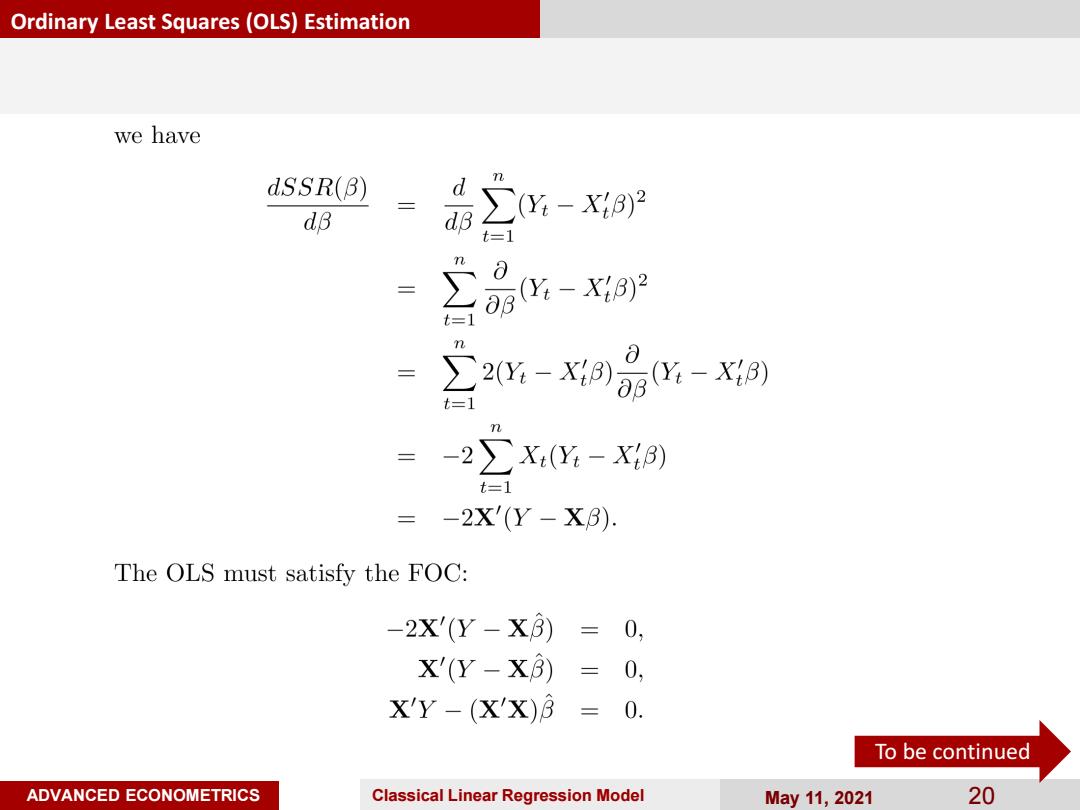
Ordinary Least Squares (OLS)Estimation we have dSSR(B) d dB 8 x-X2 t=1 = 0B (Yi-X1B)2 t=1 n = t= 2-9品出-X m = -2∑XY-X8) t=l -2X'(Y-XB). The OLS must satisfy the FOC: -2x'(Y-X8) 0 X'(Y-XB) = 0, X'Y-(X'X)B =0. To be continued ADVANCED ECONOMETRICS Classical Linear Regression Model May11,2021 20
ADVANCED ECONOMETRICS Classical Linear Regression Model May 11, 2021 20 Ordinary Least Squares (OLS) Estimation To be continued