同济大学：《空气动力学》课程电子教案（课件讲稿）Chapter 2 Some Fundamental Principles and Equations

Copyright by Dr.Zheyan Jin Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Divergence of a Vector Field The time rate of change of the volume (scalar quantity) 7。V of a moving fluid element of fixed mass,per unit volume of that element. Cartesian Coordinates: V-Vi+Vj+Vk r-那+y+y dx dy dz Cylindrical Coordinates: V=Ve,Voeo+Ve V.V-10(V)+10VOV ror 'r 00 dz Spherical Coordinates: 7=',e,+'e+',e。 V(V)sin)o r2 or rsin0 00 rsine do

Copyright by Dr. Zheyan Jin Chapter 2 Some Fundamental Principles and Equations Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Divergence of a Vector Field                            V r V r r V r r V V V e V e V e r r r sin 1 sin sin 1 ( ) 1 2 2      z V y V x V V V V i V j V k x y z x y                    z (scalar quantity) Cartesian Coordinates: Cylindrical Coordinates:   r z r r r z r               1 V V V 1 V V V e V e V e r r z z          Spherical Coordinates: V    The time rate of change of the volume of a moving fluid element of fixed mass, per unit volume of that element

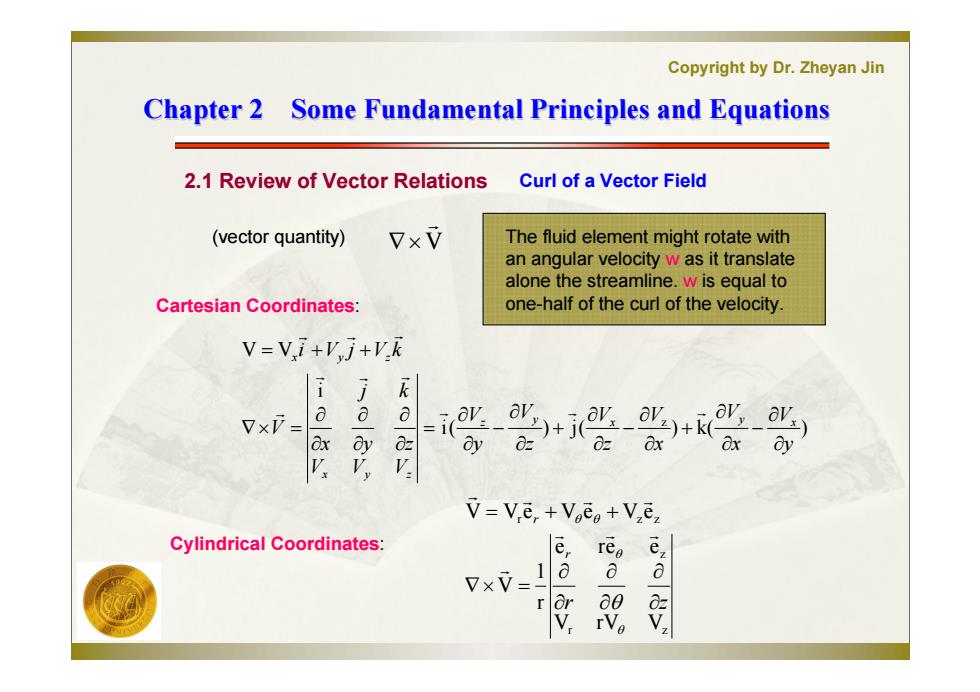
Copyright by Dr.Zheyan Jin Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Curl of a Vector Field (vector quantity) VxV The fluid element might rotate with an angular velocity w as it translate alone the streamline.w is equal to Cartesian Coordinates: one-half of the curl of the velocity. V=Vi+Vj+Vk i R VxV= ⊙ a dy R Ox r , V=Ve,+Voeo+Vez Cylindrical Coordinates: e VxV= a0 o

Copyright by Dr. Zheyan Jin Chapter 2 Some Fundamental Principles and Equations Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Curl of a Vector Field i( ) j( ) k( ) i V V z y V x V x V z V z V y V V V V x y z j k V i V j V k z y x y x x y z x y z                                        (vector quantity) Cartesian Coordinates: Cylindrical Coordinates: r z z r z z V rV V e re e r 1 V V V e V e V e     r  z r r                    The fluid element might rotate with an angular velocity w as it translate alone the streamline. w is equal to one-half of the curl of the velocity. V  

Copyright by Dr.Zheyan Jin Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Line Integrals ds b ds C The line integral of A along Closed curve curve C from point a to point b is Ads h A.ds

Copyright by Dr. Zheyan Jin Chapter 2 Some Fundamental Principles and Equations Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Line Integrals Closed curve The line integral of A along curve C from point a to point b is   b a A ds  A  ds n  A  a b C C ds   C A ds 

Copyright by Dr.Zheyan Jin Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Surface Integrals n An open surface S bounded by the closed curve C.At point P,let dS be an dS elemental area of the surface and n be unit vector normal to the surface. ds nds J∬pas Surface integral of a scalar p over the open surface S(the result is a vector) fAds Surface integral of a vector A over the open surface S(the result is a scalar) J∬Axas Surface integral of a vector A over the open surface S(the result is a vector)

Copyright by Dr. Zheyan Jin Chapter 2 Some Fundamental Principles and Equations Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Surface Integrals Surface integral of a scalar p over the open surface S (the result is a vector）   S A dS   S pdS  dS ndS      S A dS   C n  P dS Surface integral of a vector A over the open surface S (the result is a scalar） Surface integral of a vector A over the open surface S (the result is a vector） An open surface S bounded by the closed curve C. At point P, let dS be an elemental area of the surface and n be unit vector normal to the surface

Copyright by Dr.Zheyan Jin Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Volume Integrals Close surface S Volume V P ds 用aaW Volume integral of a scalar p over the closed surface S(the result is a scalar) Adv Volume integral of a vector A over the closed surface S(the result is a vector)

Copyright by Dr. Zheyan Jin Chapter 2 Some Fundamental Principles and Equations Chapter 2 Some Fundamental Principles and Equations 2.1 Review of Vector Relations Volume Integrals Volume integral of a scalar p over the closed surface S (the result is a scalar） V dV  V AdV  Close surface S n  P dS Volume integral of a vector A over the closed surface S (the result is a vector） Volume V