Decision-Procedure Based Theorem Provers Tactic-Based Theorem Proving Inferring Loop Invariants CS294-8 Lecture 12 Prof.Necula CS 294-8 Lecture 12 1
Prof. Necula CS 294-8 Lecture 12 1 Decision-Procedure Based Theorem Provers Tactic-Based Theorem Proving Inferring Loop Invariants CS 294-8 Lecture 12
Review Source language VCGen FOL Theories Goal-directed Sat.proc Sat.proc proving 1 n Prof.Necula CS 294-8 Lecture 12 2
Prof. Necula CS 294-8 Lecture 12 2 Review Source language VCGen FOL Theories Sat. proc 1 Sat. proc n Goal-directed proving … ?

Combining Satisfiability Procedures Consider a set of literals F Containing symbols from two theories Ti and Ta We split F into two sets of literals Fi containing only literals in theory Ti F2 containing only literals in theory T2 We name all subexpressions: pi(f2(E))is split into f2(E)=n^pi(n) We have:unsat (F1A F2)iff unsat(F) unsat(F)v unsat(F2)=unsat(F) But the converse is not true So we cannot compute unsat(F)with a trivial combination of the sat.procedures for Ti and Ta Prof.Necula CS 294-8 Lecture 12 3
Prof. Necula CS 294-8 Lecture 12 3 Combining Satisfiability Procedures • Consider a set of literals F – Containing symbols from two theories T1 and T2 • We split F into two sets of literals – F1 containing only literals in theory T1 – F2 containing only literals in theory T2 – We name all subexpressions: p1 (f2 (E)) is split into f2 (E) = n p1 (n) • We have: unsat (F1 F2 ) iff unsat(F) – unsat(F1 ) unsat(F2 ) unsat(F) – But the converse is not true • So we cannot compute unsat(F) with a trivial combination of the sat. procedures for T1 and T2
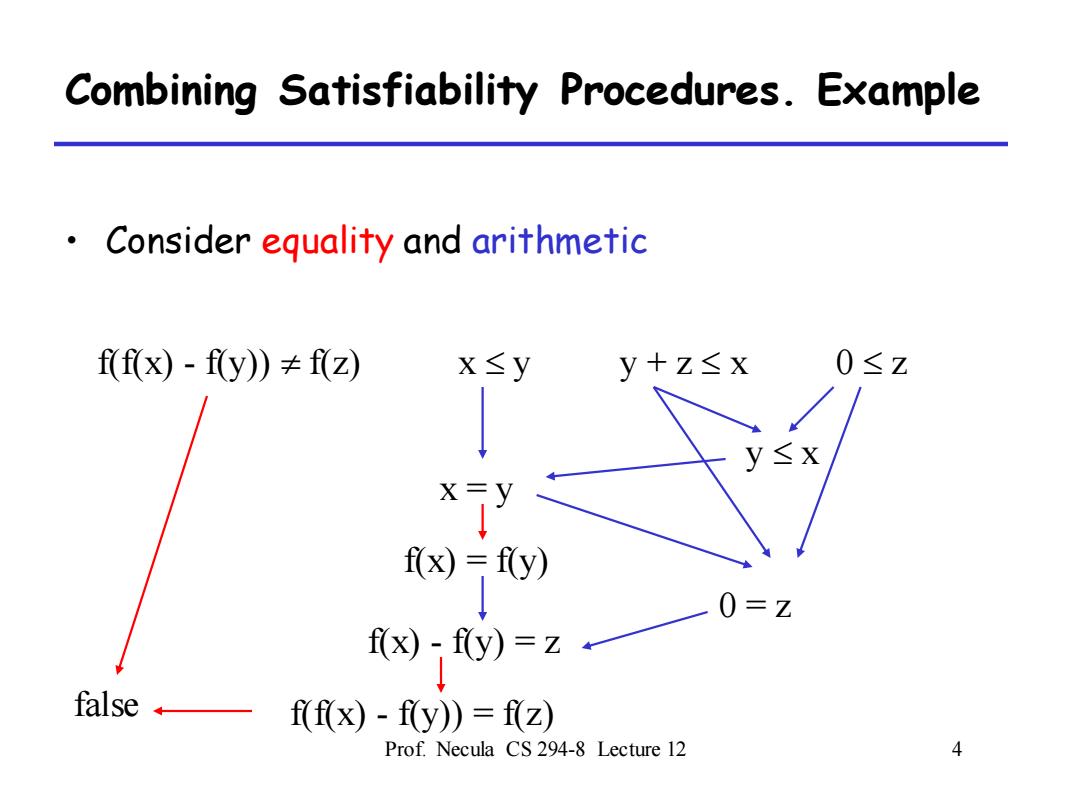
Combining Satisfiability Procedures.Example Consider equality and arithmetic ffx)-fy)≠fz) x≤y y+Z≤X 0≤Z y≤x X- f(x)=f(y) 0=Z fx)-fy)=z false← f(f(x)-f(y))=f(z) Prof.Necula CS 294-8 Lecture 12 4
Prof. Necula CS 294-8 Lecture 12 4 Combining Satisfiability Procedures. Example • Consider equality and arithmetic f(f(x) - f(y)) f(z) x y y + z x 0 z false f(x) = f(y) y x x = y 0 = z f(x) - f(y) = z f(f(x) - f(y)) = f(z)

Combining Satisfiability Procedures Combining satisfiability procedures is non trivial And that is to be expected: Equality was solved by Ackerman in 1924,arithmetic by Fourier even before,but E+A only in 1979 yet in any single verification problem we will have literals from several theories: Equality,arithmetic,lists,... When and how can we combine separate satisfiability procedures? Prof.Necula CS 294-8 Lecture 12 5
Prof. Necula CS 294-8 Lecture 12 5 Combining Satisfiability Procedures • Combining satisfiability procedures is non trivial • And that is to be expected: – Equality was solved by Ackerman in 1924, arithmetic by Fourier even before, but E + A only in 1979 ! • Yet in any single verification problem we will have literals from several theories: – Equality, arithmetic, lists, … • When and how can we combine separate satisfiability procedures?