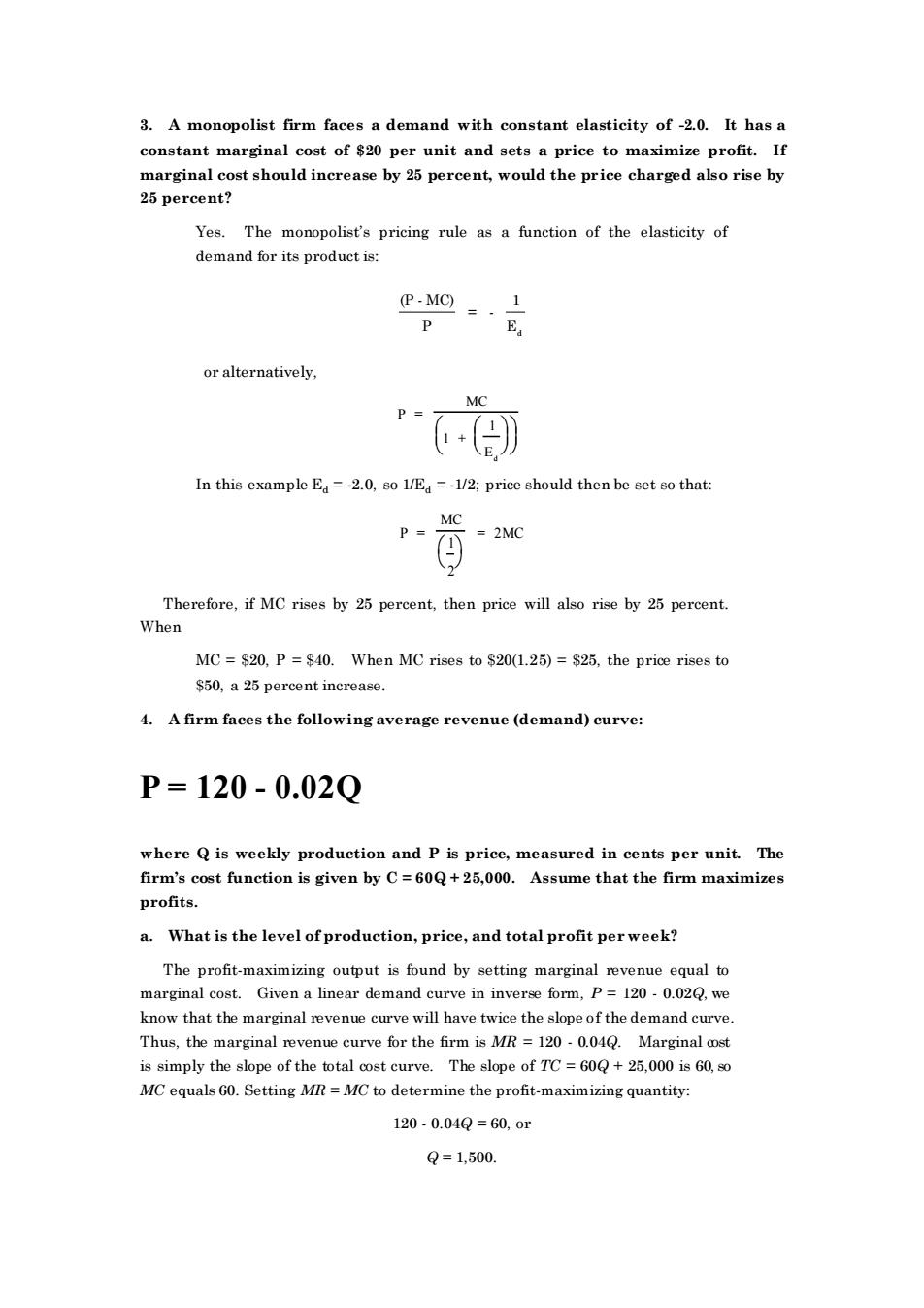
3.A monopolist firm faces a demand with constant elasticity of-2.0.It has a constant marginal cost of $20 per unit and sets a price to maximize profit.If marginal Icost should increase by 25 percent,would the price charged also rise by 25 percent? Yes.The monopolist's pricing rule as a function of the elasticity of demand for its product is: or alternatively MC P=7 In this example E=-2.0.so 1/E=-1/2:price should then be set so that P= MC =2MC Therefore,if MC rises by 25 percent,then price will also rise by 25 percent When MC=$20.P=$40.When MC rises to $20(1.25)=$25,the price rises to $50,a 25 percent increase. 4.A firm faces the following average revenue(demand)curve: P=120-0.02Q where Q is weekly production and P is price,measured in cents per unit.The firm's cost function is given by C=60Q+25,000.Assume that the firm maximizes profits. a.What is the level ofproduction,price,and total profit perweek? The profit-ma四g0upu此found by sctting margina9"o mmal to margina ost Giver linear demand c rm, 20.0.02Q,we know that the marginal revenue curve will have twice the slope of the demand curve Thus,the marginal revenue curve for the firm is MR =120.0.04Q.Marginal oost is simply the slope of the total cost curve.The slope of TC=60Q+25.000 is 60.s MC equals 60.Setting MR=MC to determine the profit-maximizing quantity: 120.0.04Q=60.0or Q=1,500
3. A monopolist firm faces a demand with constant elasticity of -2.0. It has a constant marginal cost of $20 per unit and sets a price to maximize profit. If marginal cost should increase by 25 percent, would the price charged also rise by 25 percent? Yes. The monopolist’s pricing rule as a function of the elasticity of demand for its product is: (P - MC) P = - 1 Ed or alternatively, P = MC 1 + 1 Ed In this example Ed = -2.0, so 1/Ed = -1/2; price should then be set so that: P = MC 1 2 = 2MC Therefore, if MC rises by 25 percent, then price will also rise by 25 percent. When MC = $20, P = $40. When MC rises to $20(1.25) = $25, the price rises to $50, a 25 percent increase. 4. A firm faces the following average revenue (demand) curve: P = 120 - 0.02Q where Q is weekly production and P is price, measured in cents per unit. The firm’s cost function is given by C = 60Q + 25,000. Assume that the firm maximizes profits. a. What is the level of production, price, and total profit per week? The profit-maximizing output is found by setting marginal revenue equal to marginal cost. Given a linear demand curve in inverse form, P = 120 - 0.02Q, we know that the marginal revenue curve will have twice the slope of the demand curve. Thus, the marginal revenue curve for the firm is MR = 120 - 0.04Q. Marginal cost is simply the slope of the total cost curve. The slope of TC = 60Q + 25,000 is 60, so MC equals 60. Setting MR = MC to determine the profit-maximizing quantity: 120 - 0.04Q = 60, or Q = 1,500

Substituting the profit-maximizing quantity into the inverse demand function to determine the price: P=120.(0.021,500)=90 cents Profitequals total revenue minus total cost: 元=(901,500)-(25,000+(601,500).or 元=$200 per week. b.If the government decides to levy a tax of 14 cents per unit on this product what will be the new level of production,price,and profit? Suppose initially that the consumers must pay the tax to the government. Since the total price (including the tax)consumers woul be willing to pay remains unchanged,we know that the demand function is p*+T=120.0.02Q or p*=120.0.02Q.T where is the price recived by the suppliers Because the tax increases the price of each unit,total revenue for the moropolist decreases by T and marginal revenue,the revenue on each additional unit,decreases by 7. MR=120-0.04Q-T where T=14 cents.To determine the profit-maximizing level of output with the tax,equate marginal revenue with marginal cost: 120.0.04Q-14=60,or Q=1,150 units Substituting into the demand function to determine price p*=120-(0.021,150)-14=83 cents Profit is total revenue minus total cos π=830150)-(600,150)+25,000)-1450 cents,o $14.50 per week. Note:The price facing theco after the imposition of the tax is 97cent The monopolist receives 83 cents.Therefore,the consumer and the monopolist each pay 7 cents of the tax If the monopolist had to pay the tax instead of the consumer,we would arrive at the same result.The monopolist's cost function would then be TC=60Q+25,000+TQ=(60+T)Q+25,000. The slope of the cost function is(60+T so MC=60+T.We set this MC to the marginal revenue function from part (a) 120.0.04Q=60+14,o
Substituting the profit-maximizing quantity into the inverse demand function to determine the price: P = 120 - (0.02)(1,500) = 90 cents. Profit equals total revenue minus total cost: = (90)(1,500) - (25,000 + (60)(1,500)), or = $200 per week. b. If the government decides to levy a tax of 14 cents per unit on this product, what will be the new level of production, price, and profit? Suppose initially that the consumers must pay the tax to the government. Since the total price (including the tax) consumers would be willing to pay remains unchanged, we know that the demand function is P* + T = 120 - 0.02Q, or P* = 120 - 0.02Q - T, where P* is the price received by the suppliers. Because the tax increases the price of each unit, total revenue for the monopolist decreases by TQ, and marginal revenue, the revenue on each additional unit, decreases by T: MR = 120 - 0.04Q - T where T = 14 cents. To determine the profit-maximizing level of output with the tax, equate marginal revenue with marginal cost: 120 - 0.04Q - 14 = 60, or Q = 1,150 units. Substituting Q into the demand function to determine price: P* = 120 - (0.02)(1,150) - 14 = 83 cents. Profit is total revenue minus total cost: = (83)(1,150)− ((60)(1,150) + 25,000)= 1450 cents, or $14.50 per week. Note: The price facing the consumer after the imposition of the tax is 97 cents. The monopolist receives 83 cents. Therefore, the consumer and the monopolist each pay 7 cents of the tax. If the monopolist had to pay the tax instead of the consumer, we would arrive at the same result. The monopolist’s cost function would then be TC = 60Q + 25,000 + TQ = (60 + T)Q + 25,000. The slope of the cost function is (60 + T), so MC = 60 + T. We set this MC to the marginal revenue function from part (a): 120 - 0.04Q = 60 + 14, or

Q=1,150. Thus,it does not matter who ends the tax payment to the vernment.The burdenofthe tax is reflected in the price ofthe good. 5.The following table shows the demand curve facing a monopolist who produces at a constant marginal cost of $10 Price Quantity 0 16 2 12 10 8 20 6 24 4 28 2 32 0 36 a.Calculate the firm's marginal revenue curve. To find the marginal revenue curw we first derive the inverse demand curve curve the .The of the inverse demand curveisthe change in price divided by the change in quantity Fo example,a decrease in price from 18 to 16 yields an increase in quantity from 0 to 4. Therer the sopeand the demand curveis P=18-0.50 The marginal revenue curve corresponding to a linear demand curve is a line with the same interept as the inverse demand curve and a slope that is twice as steep.Therefore,the marginal revenue curve is MR=18-Q. b.What are the firm's profit-maximizing output and price?What is its profit? The monopolist's maximizing output occurs where marginal revenue equals marginal cost.Marginal cost is a constant $10.Setting MR equal to MC to determine the profit-maximizing quantity: 18-Q=10.orQ=8. To find the profit-maximizing price,substitute this quantity into the demand equation: P=18-0.5)8)=$14. Total revenue is price times quantity: TR=04)8)=S112
Q = 1,150. Thus, it does not matter who sends the tax payment to the government. The burden of the tax is reflected in the price of the good. 5. The following table shows the demand curve facing a monopolist who produces at a constant marginal cost of $10. Price Quantity 18 0 16 4 14 8 12 12 10 16 8 20 6 24 4 28 2 32 0 36 a. Calculate the firm’s marginal revenue curve. To find the marginal revenue curve, we first derive the inverse demand curve. The intercept of the inverse demand curve on the price axis is 18. The slope of the inverse demand curve is the change in price divided by the change in quantity. For example, a decrease in price from 18 to 16 yields an increase in quantity from 0 to 4. Therefore, the slope is − 1 2 and the demand curve is P = 18 − 0.5Q. The marginal revenue curve corresponding to a linear demand curve is a line with the same intercept as the inverse demand curve and a slope that is twice as steep. Therefore, the marginal revenue curve is MR = 18 - Q. b. What are the firm’s profit-maximizing output and price? What is its profit? The monopolist’s maximizing output occurs where marginal revenue equals marginal cost. Marginal cost is a constant $10. Setting MR equal to MC to determine the profit-maximizing quantity: 18 - Q = 10, or Q = 8. To find the profit-maximizing price, substitute this quantity into the demand equation: P = 18− (0.5)(8) = $14. Total revenue is price times quantity: TR = (14)(8) = $112

The profit of the firm is total revenue minus total cost,and total cost is equal to average cost times the kvel of ouput produed.Since marginal cos isconstan average variable cost is equal to marginal cost.Ignoring any fixed costs,total cost is 100 or 80,and profit is 112-80=$32 c.What would the equilibrium price and quantity be in a competitive industry? Setting the expre for price equal toamarginal cot of10 18-0.50=10→2=16→P=10. Note the increase in the equilibrium quantity compared to the monopoly solution. d.What would the social gain be if this monopolist were forced to produce and price at the competitive equilibrium?Who would gain and lose as a result? The social gain arises from the elimination ofdeadweight loss.Deadweight lss in this case is equal to the triangle above the onstant marginal cos eurve,below the demand curve.and between the quanti es8 and 16.or numerically (14-10)(16-8M.5=$16. Consumers gain this deadweight lss plus the monopolist's profit of $32.The monopolist's profits are reduced toro,and the consumer surplus increases by $48. 6.Suppose that an industry is characterized as follows: C=100+2Q Firmtotal cost finction MC=40 Frm marginal cost function P=90-20 Industry demand curve MR=90-4Q a.If there is only one firm in the industry,find the monopoly price,quantity, and level of profit. If there is only one firm in the industry,then the firm will act like a monopolist and produce at the point where marginal revenue is equal to marginal cost. MC=4Q=90-4Q=MR Q=11.25 For a quantity of 11.25.the firm will charge a price P=90-2*11.25=67.50 The1 evel of67.50*11.25-10-2*11.25*11.25=s406.25. b.Find the price,quantity,and level of profit if the industry is competitive
The profit of the firm is total revenue minus total cost, and total cost is equal to average cost times the level of output produced. Since marginal cost is constant, average variable cost is equal to marginal cost. Ignoring any fixed costs, total cost is 10Q or 80, and profit is 112 − 80 = $32. c. What would the equilibrium price and quantity be in a competitive industry? For a competitive industry, price would equal marginal cost at equilibrium. Setting the expression for price equal to a marginal cost of 10: 18 − 0.5Q =10 Q = 16 P =10. Note the increase in the equilibrium quantity compared to the monopoly solution. d. What would the social gain be if this monopolist were forced to produce and price at the competitive equilibrium? Who would gain and lose as a result? The social gain arises from the elimination of deadweight loss. Deadweight loss in this case is equal to the triangle above the constant marginal cost curve, below the demand curve, and between the quantities 8 and 16, or numerically (14-10)(16-8)(.5)=$16. Consumers gain this deadweight loss plus the monopolist’s profit of $32. The monopolist’s profits are reduced to zero, and the consumer surplus increases by $48. 6. Suppose that an industry is characterized as follows: C = 100 + 2Q 2 Firm total cost function MC = 4Q Firm marginal cost function P = 90 − 2Q Industry demand curve MR = 90 − 4Q Industry marginal revenue curve . a. If there is only one firm in the industry, find the monopoly price, quantity, and level of profit. If there is only one firm in the industry, then the firm will act like a monopolist and produce at the point where marginal revenue is equal to marginal cost: MC=4Q=90-4Q=MR Q=11.25. For a quantity of 11.25, the firm will charge a price P=90-2*11.25=$67.50. The level of profit is $67.50*11.25-100-2*11.25*11.25=$406.25. b. Find the price, quantity, and level of profit if the industry is competitive