《化工热力学》课程授课教案（讲义）Chapter 5 The Second Law of Thermodynamics

Chapter 5 The Second Law of Thermodynamics Introduction 5.1 Statements of the Second Law 5.2 Heat Engines 5.4 Entropy 5.5 Entropy Change of an Ideal as 5.6 Mathematical Statement of the Second Law 5.7 Entropy Balance for Open Systems 5.8 Calculation of Ideal Work 5.9 Lost Work Introduction The First Law of thermodynamics Conservation and transformation of energy Can the process which accords with the First Law occur spontaneousty? :A process which can occur automatically without any outside effect eous process exist mposes no restriction on the process direction The Second Law of thermodynamics to describe the direction.conditions and bounds of the spontaneous process 5.1 Statements of the Second Law Statement /(Kelvin-Planck Statement):No apparatus can operate in such a way that its only effect is to convert heat absorbed by a system completely into work done by the system. 不可能从单一热源取热，并使之完全转变为有用功而不产生其它影响 Statement 2 (Clausius t)No is possible which consists solely in the transfer of heat from one temperature level to a higher one 不可能将热从低温物体传至高温物体而不引起其它变化 两种表述完全等效：违反一种表述，必违反另一种表述： It is impossible by a eyclic process to convert the heat absorbed by a system mpletely into work done by the Se:perpetual-motion machine of the kind can'tbe buil 5.2 Heat Engines Heat engine is a device or machine that roduce work from heat in a cyclic pr The First Law therefore reduces to w=lo-loc The thermal efficiency Offering water

Chapter 5 The Second Law of Thermodynamics Introduction 5.1 Statements of the Second Law 5.2 Heat Engines 5.4 Entropy 5.5 Entropy Change of an Ideal Gas 5.6 Mathematical Statement of the Second Law 5.7 Entropy Balance for Open Systems 5.8 Calculation of Ideal Work 5.9 Lost Work Introduction The First Law of thermodynamics Conservation and transformation of energy Can the process which accords with the First Law occur spontaneously? spontaneous process: A process which can occur automatically without any outside effect In nature, the spontaneous process exist direction The First Law of thermodynamics reflects the observation that energy is conserved, but it imposes no restriction on the process direction The Second Law of thermodynamics to describe the direction, conditions and bounds of the spontaneous process 5.1 Statements of the Second Law Statement 1 (Kelvin－Planck Statement):No apparatus can operate in such a way that its only effect is to convert heat absorbed by a system completely into work done by the system. 不可能从单一热源取热，并使之完全转变为有用功而不产生其它影响 Statement 2 (Clausius statement):No process is possible which consists solely in the transfer of heat from one temperature level to a higher one. 不可能将热从低温物体传至高温物体而不引起其它变化 两种表述完全等效! 违反一种表述,必违反另一种表述! Statement 1a: It is impossible by a cyclic process to convert the heat absorbed by a system completely into work done by the system. Statement 1b: perpetual-motion machine of the second kind can’t be built 5.2 Heat Engines Heat engine is a device or machine that produce work from heat in a cyclic process For example: a steam power plant The First Law therefore reduces to: The thermal efficiency condenser Q c boiler turbin e Generato r W Q H Offering water W Q Q = − H C

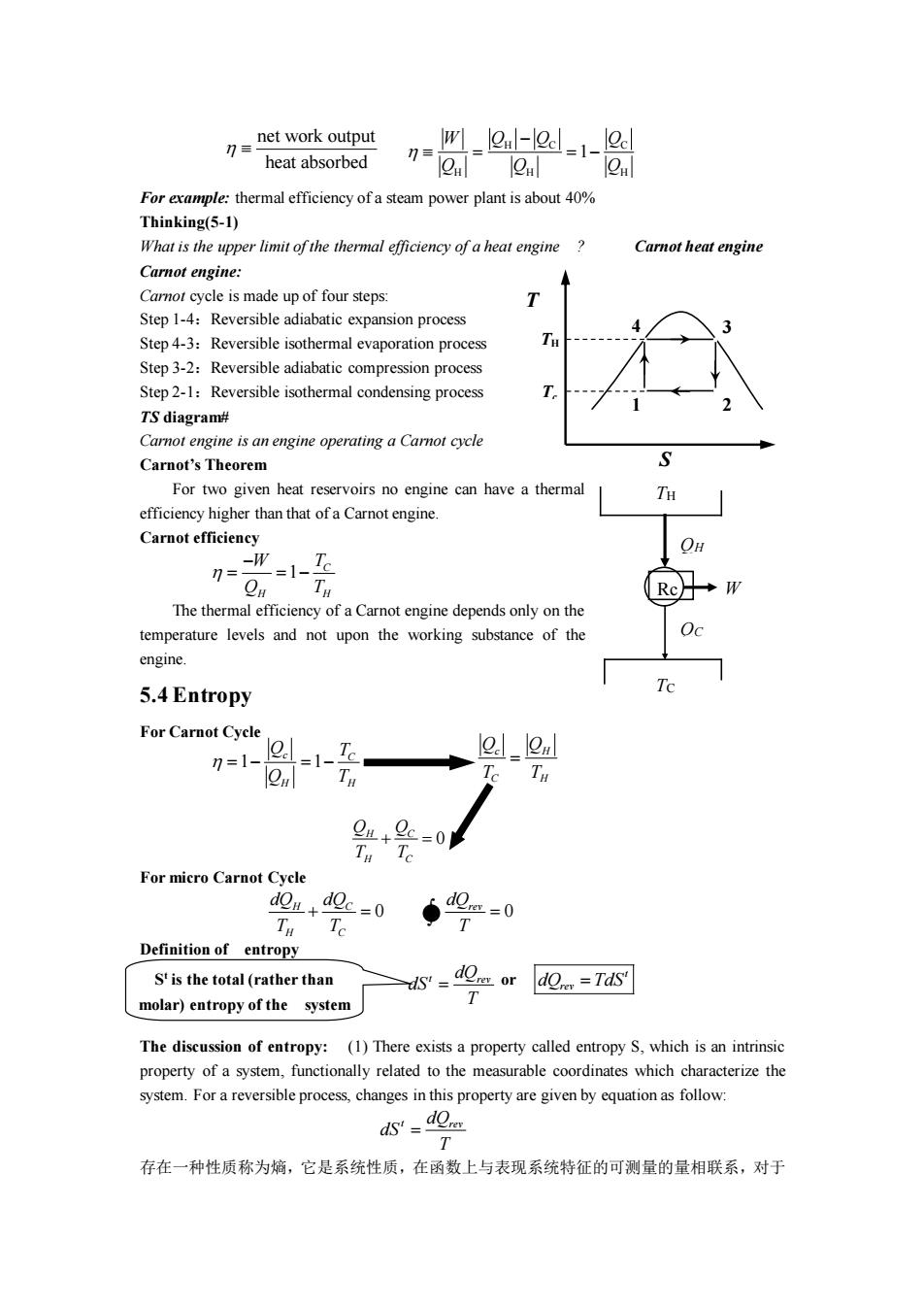
net work output n heat absorbed m_-1- -jeu eul 2h :thermal efficiency of a steam power plant is about40% Thinking(5-1) What is the upper limit of the thermal efficiency of a heat engine Carnot heat engine Carnot engine: ismade up of four steps Step 1-4:Reversible adiabatic expansion process Step4-3:Reversible isothermal evaporation process T Step3-2:Reversible adiabatic compression process Step2-1:Reversible isothermal condensing process TS diagram# Carnot engine is an engine operating a Camot cycle Carnot's Theorem For two given heat reservoirs no engine can have a thermal TH efficiency higher than that of a Carnot engine. Carnot efficiency -1-T Tu ◆W The thermal efficiency of a Carnot engine depends only on the temperature levels and not upon the working substance of the 5.4Entropy Te For Carnot Cycle =11 1C l2ml .2c-0 T I For micro Carnot Cycle Te 4-0 S'is the total (rather than s.0-or0n=7☒ molar)entropy of the system The discussion of entropy:(1)There exists a property called entropy S.which is an intrinsic able coo swhich characterize the 1T 存在一种性质称为嫡，它是系统性质，在函数上与表现系统特征的可测量的量相联系，对于

For example: thermal efficiency of a steam power plant is about 40% Thinking(5-1) What is the upper limit of the thermal efficiency of a heat engine ? Carnot heat engine Carnot engine: Carnot cycle is made up of four steps: Step 1-4：Reversible adiabatic expansion process Step 4-3：Reversible isothermal evaporation process Step 3-2：Reversible adiabatic compression process Step 2-1：Reversible isothermal condensing process TS diagram# Carnot engine is an engine operating a Carnot cycle Carnot’s Theorem For two given heat reservoirs no engine can have a thermal efficiency higher than that of a Carnot engine. Carnot efficiency The thermal efficiency of a Carnot engine depends only on the temperature levels and not upon the working substance of the engine. 5.4 Entropy For Carnot Cycle For micro Carnot Cycle Definition of entropy The discussion of entropy: (1) There exists a property called entropy S, which is an intrinsic property of a system, functionally related to the measurable coordinates which characterize the system. For a reversible process, changes in this property are given by equation as follow: 存在一种性质称为熵，它是系统性质，在函数上与表现系统特征的可测量的量相联系，对于 net work output heat absorbed   H C C H H H 1 W Q Q Q Q Q Q  −  = = − 2 4 3 1 T S TH Tc TH TC Rc QH QC W 1 C H H W T Q T  − = = − 1 1 c C H H Q T Q T  = − = − c H C H Q Q T T = 0 H C H C Q Q T T + = 0 H C H C dQ dQ T T + = 0 rev dQ T =  t rev dQ dS T = or t rev S dQ TdS = t is the total (rather than molar) entropy of the system t rev dQ dS T =

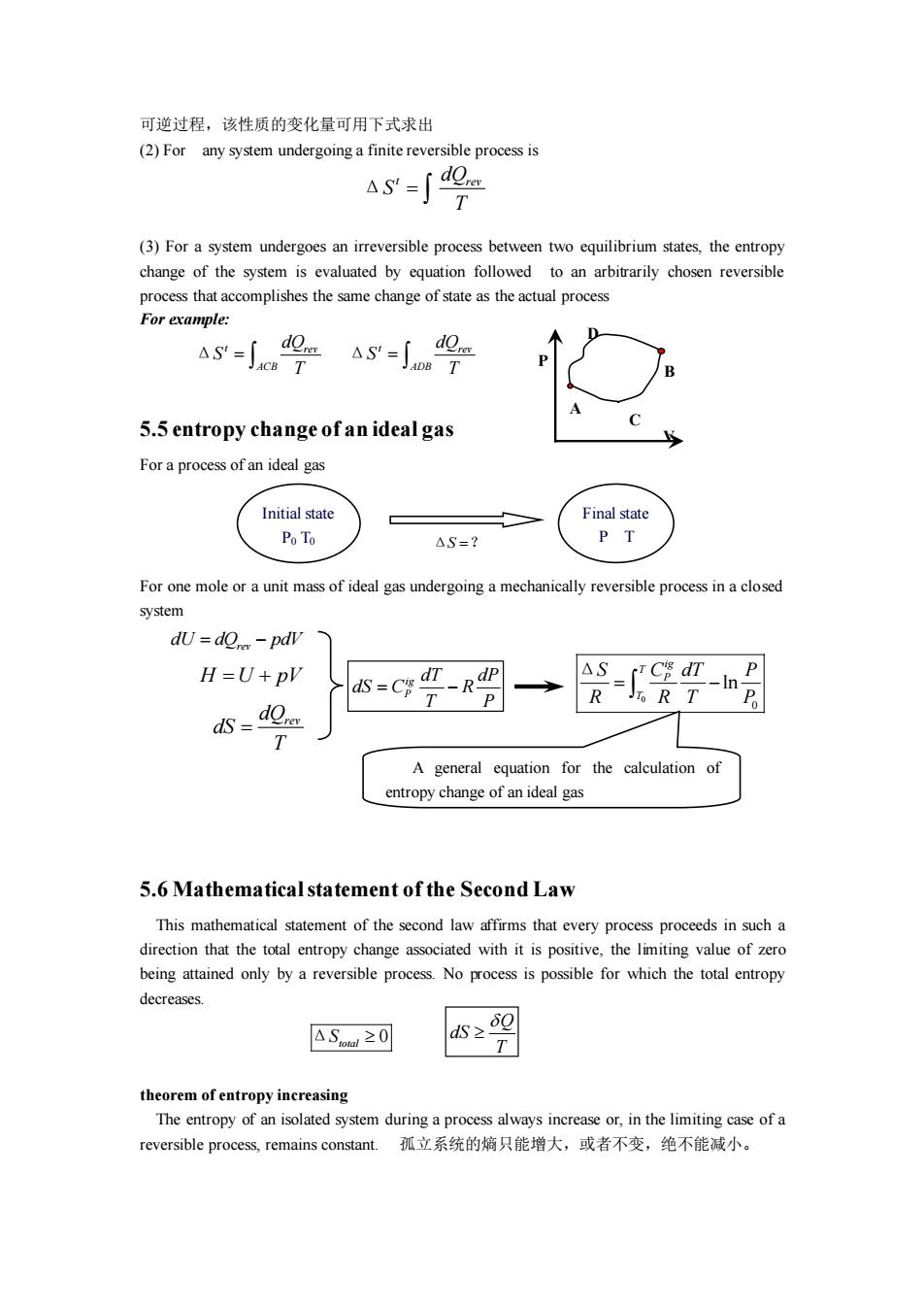
可逆过程，该性质的变化量可用下式求出 (2)For any system undergoing a finite reversible process is 4s-∫№ (3)For a system undergoes an irreversible process between two equilibrium states.the entropy change of the ystem is evaluated byqution followed to a arbitrarily chosen reversibl proces that accomplishes the same change of state as the actual process For example: 5.5 entropy change of an ideal gas For a process of an ideal gas Initial state Final state PoTo △S=? PT For one mole or a unit mass of ideal gas undergoing a mechanically reversible process in a closed system du =de-pdv H=U+pV dS=Cg T-R T P as-cd-In P A general equation for the calculation of entropy change of an ideal gas 5.6 Mathematical statement of the Second Law roceeds in such a that the tol entropy change with it is positive,value ofe being attained only by a reversible process.No process is possible for which the total entropy decreases. △Saa≥2d theorem of entropy increasing The entropy of an isolated system during a process always increase or,in the limiting case of a reversible process,remains constant.孤立系统的熵只能增大，或者不变，绝不能减小

可逆过程，该性质的变化量可用下式求出 (2) For any system undergoing a finite reversible process is (3) For a system undergoes an irreversible process between two equilibrium states, the entropy change of the system is evaluated by equation followed to an arbitrarily chosen reversible process that accomplishes the same change of state as the actual process For example: 5.5 entropy change of an ideal gas For a process of an ideal gas For one mole or a unit mass of ideal gas undergoing a mechanically reversible process in a closed system 5.6 Mathematical statement of the Second Law This mathematical statement of the second law affirms that every process proceeds in such a direction that the total entropy change associated with it is positive, the limiting value of zero being attained only by a reversible process. No process is possible for which the total entropy decreases. theorem of entropy increasing The entropy of an isolated system during a process always increase or, in the limiting case of a reversible process, remains constant. 孤立系统的熵只能增大，或者不变，绝不能减小。 Initial state P0 T0 Final state P T Δ ？ S = ig P dT dP dS C R T P = − 0 0 ln ig T P T S dT P C R R T P = −  Δ A general equation for the calculation of entropy change of an ideal gas rev dU dQ pdV = − H U pV = + rev dQ dS T = t rev dQ S T = Δ  t rev ACB dQ S T = Δ  t rev ADB dQ S T = Δ  V P D B A C Q dS T  0  total ΔS 

Thinking(5-2) 第二类永动机？ 如果三峡水电站用降温法发电，使水温降低5C,发电能力可提高11.7倍。 设水位差为180米 重力势能转化为电能 E=mgh =1800m(J] mkg水降低5C放热： 2_21000m=117 E1800m Q=cm△1=21000mlJ] Thinking(5-3) 若工质从同一初态，分别经可逆和不可逆过程，到达同一终态，己知两过程热源相同，问传 热量是否相同？ △S≥∫一可递过程 >:不可逆过程 相同初终态，△s相同 热源T相同 80R>60R Q+AU+W 阳 Thinking(5-4) 若工质从同一初态出发，从相同热源吸收相同热量，问末态箱可逆与不可逆谁大？ AS2 ：可逆过程 相阿路层格短而明不思 相同初态相同S2R>S2R

Thinking(5-2) 第二类永动机??? 如果三峡水电站用降温法发电，使水温降低 5C，发电能力可提高 11.7 倍。 设水位差为 180 米 重力势能转化为电能： Thinking(5-3) 若工质从同一初态，分别经可逆和不可逆过程，到达同一终态，已知两过程热源相同，问传 热量是否相同？ 相同初终态，s 相同 热源 T 相同 Thinking(5-4) 若工质从同一初态出发，从相同热源吸收相同热量，问末态熵可逆与不可逆谁大？ E mgh m J = =1800 [ ] mkg水降低5C放热： Q cm t m J =  = 21000 [ ] 21000 11.7 1800 Q m E m = = 相同热量，热源T相同 Q S T     =：可逆过程 >：不可逆过程 IR R    S S 相同初态s1相同 2,IR 2,R S S  Q S T     =：可逆过程 >：不可逆过程 Q Q R IR    Q U W =  + 相同 W W R IR 

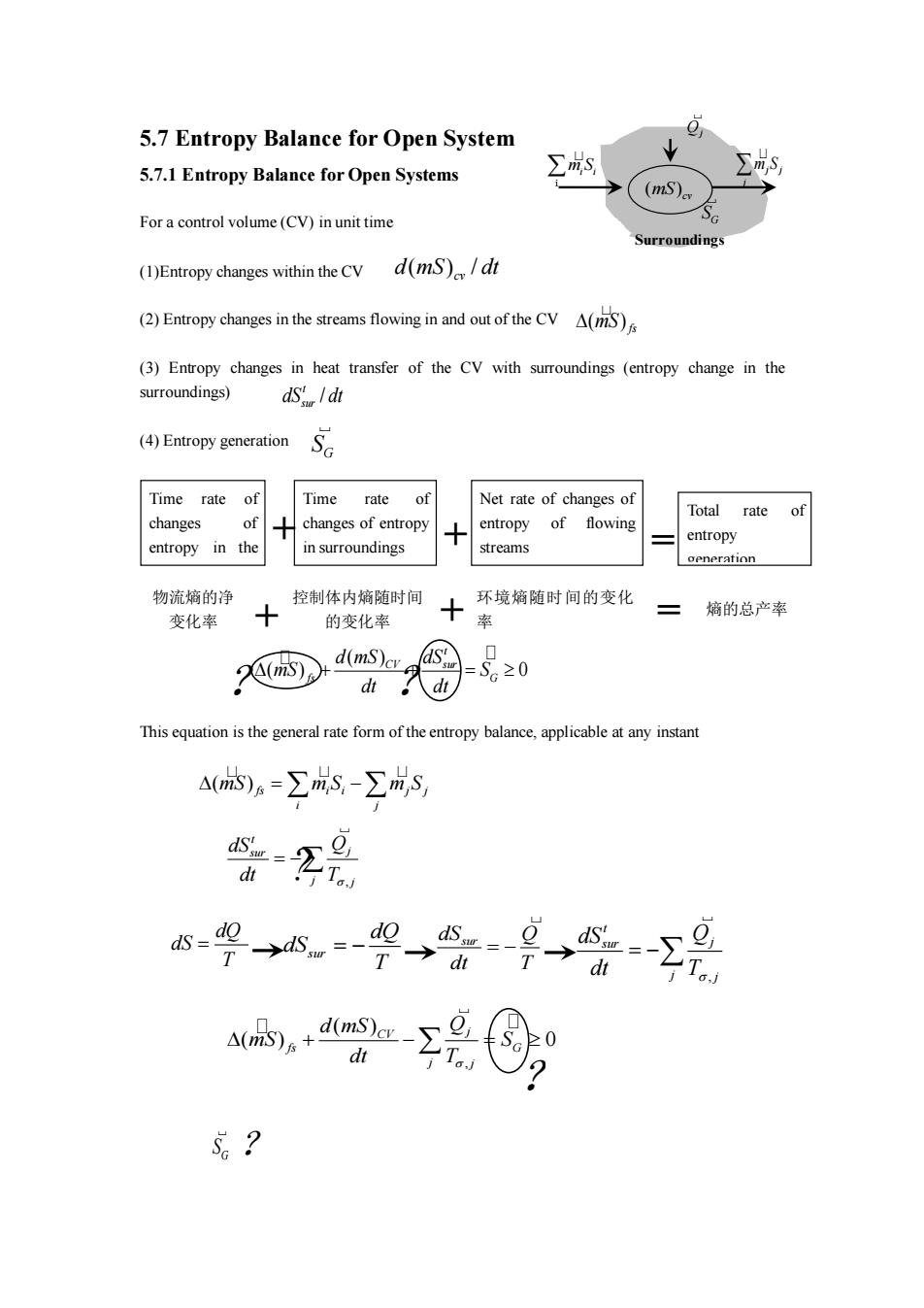
5.7 Entropy Balance for Open System 5.7.1 Entropy Balance for Open Systems ∑s (mS) For a control volume(CV)inunit time rroundings (1)Entropy changes within the CV d(mS)/dt (2)Entropy changes in the streams flowing in and out of the CVA( (3)Entropy changes in heat transfer of the CV with surroundings (entropy change in the surroundings) ds /dt (4)Entropy generationS Time rate of Time rate of Net rate of changes of Total rate of changes of changes of entropy entropy of flowing entropy in the in surroundings streams entropy gen tion 物流熵的净 控制体内箱随时间 环境熵随时间的变化 变化率 三熵的总产率 的变化率 率 )d( @-20 d。d山 This equation is the general rate form of the entropy balance,applicable at any instant △)a=∑iS-∑成S, T 4(d。+dmsa-ygQ d ?

( )fs i i j j i j  = − mS m S m S   , t j sur j j dS Q dt T = − , t j sur j j dS Q dt T = − dSsur Q dt T = − sur dQ dS T = − dQ dS T = , ( ) ( ) 0 CV j fs G j j d mS Q mS S dt T  + − =   G S 5.7 Entropy Balance for Open System 5.7.1 Entropy Balance for Open Systems For a control volume (CV) in unit time (1)Entropy changes within the CV (2) Entropy changes in the streams flowing in and out of the CV (3) Entropy changes in heat transfer of the CV with surroundings (entropy change in the surroundings) (4) Entropy generation This equation is the general rate form of the entropy balance, applicable at any instant Time rate of changes of entropy in the CV Time rate of changes of entropy in surroundings Total rate of entropy generation Net rate of changes of entropy of flowing + + streams = mSi i i j j j m S Qj ( ) mS cv Surroundings GS ( ) / cv d mS dt ( )  mS fs G S / t sur dS dt 物流熵的净 变化率 控制体内熵随时间 的变化率 环境熵随时间的变化 率 + = 熵的总产率 + ( ) ( ) 0 t CV sur fs G d mS dS mS S dt dt  + + =  ？ ？ ? ？ ？