
ME369-系统模型、分折与控制 3.1拉普拉氏变换-定义及性质 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 内容汇总 -拉普拉氏变换(LT)定义 按定义 LT求取 查表 通过LT性质 -拉普拉氏反变换(LD求取 部分分式法 终值定理小议 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 1
1 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 3.1 拉普拉氏变换 –定义及性质 ME 369– 系统模型、分析与控制 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University --拉普拉氏变换(LT) 定义 --LT 求取 按定义 通过LT性质 查表 --拉普拉氏反变换(ILT)求取 部分分式法 --终值定理小议 内容汇总
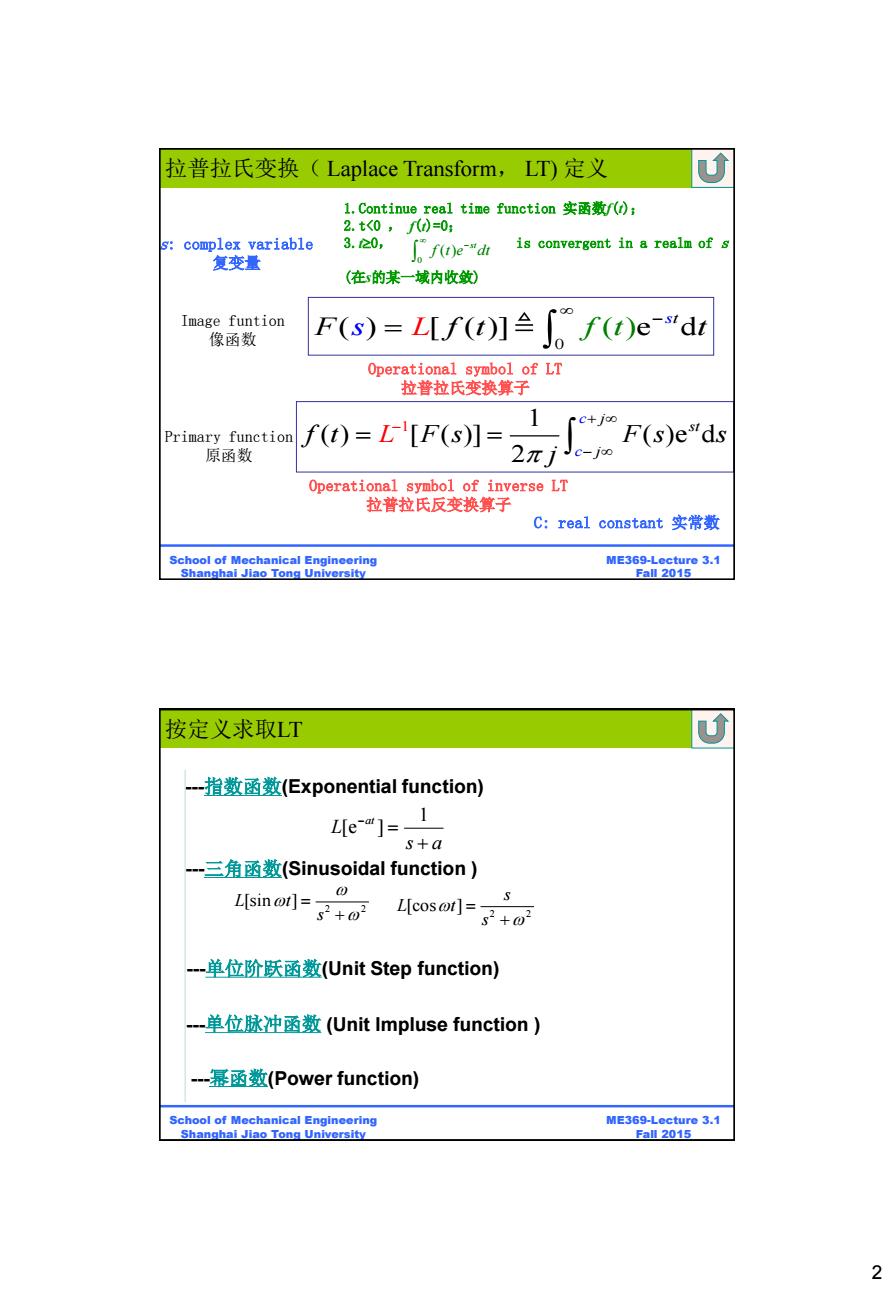
拉普拉氏变换(Laplace Transform,LT定义 i,Continue real time function实函数r(): 2.t0,f()=0: s:complex variable 3.20, is convergent in a realm of s 复变量 f(De"dr (在的某一域内收敛) Image funtion 像函数 F(s) f(t)e-sdt Operational symbol of LT 拉普拉氏变换算子 1 Primary function f(t)=L'[F(s)]= F(s)e"ds 原函数 2πjJc-jm Operational symbol of inverse LT 拉普拉氏反变换算子 C:real constant实常数 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 按定义求取LT -一指数函数(Exponential function) [e]= s+a -三角函数(Sinusoidal function) Lisinon=o Licosor= -单位阶跃函数(Unit Step function) -单位脉冲函数(Unit Impluse function) -幂函数(Power function) School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 2
2 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 拉普拉氏变换( Laplace Transform, LT) 定义 0 ( ) [ ( )] e d ( ) st F f t t s L f t s: complex variable 复变量 Image funtion 像函数 Primary function 原函数 1.Continue real time function 实函数f(t); 2.t<0 , f(t)=0; 3.t0, is convergent in a realm of s (在s的某一域内收敛) 0 ( ) st f t e dt Operational symbol of LT 拉普拉氏变换算子 1 1 ( ) [ ( )] ( )e d 2 c j c st j f t F s F s s j L C: real constant 实常数 Operational symbol of inverse LT 拉普拉氏反变换算子 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 按定义求取LT ---指数函数(Exponential function) ---三角函数(Sinusoidal function ) ---幂函数(Power function) ---单位阶跃函数(Unit Step function) ---单位脉冲函数 (Unit Impluse function ) 1 [e ] at L s a 2 2 L t [sin ] s 2 2 [cos ] s L t s
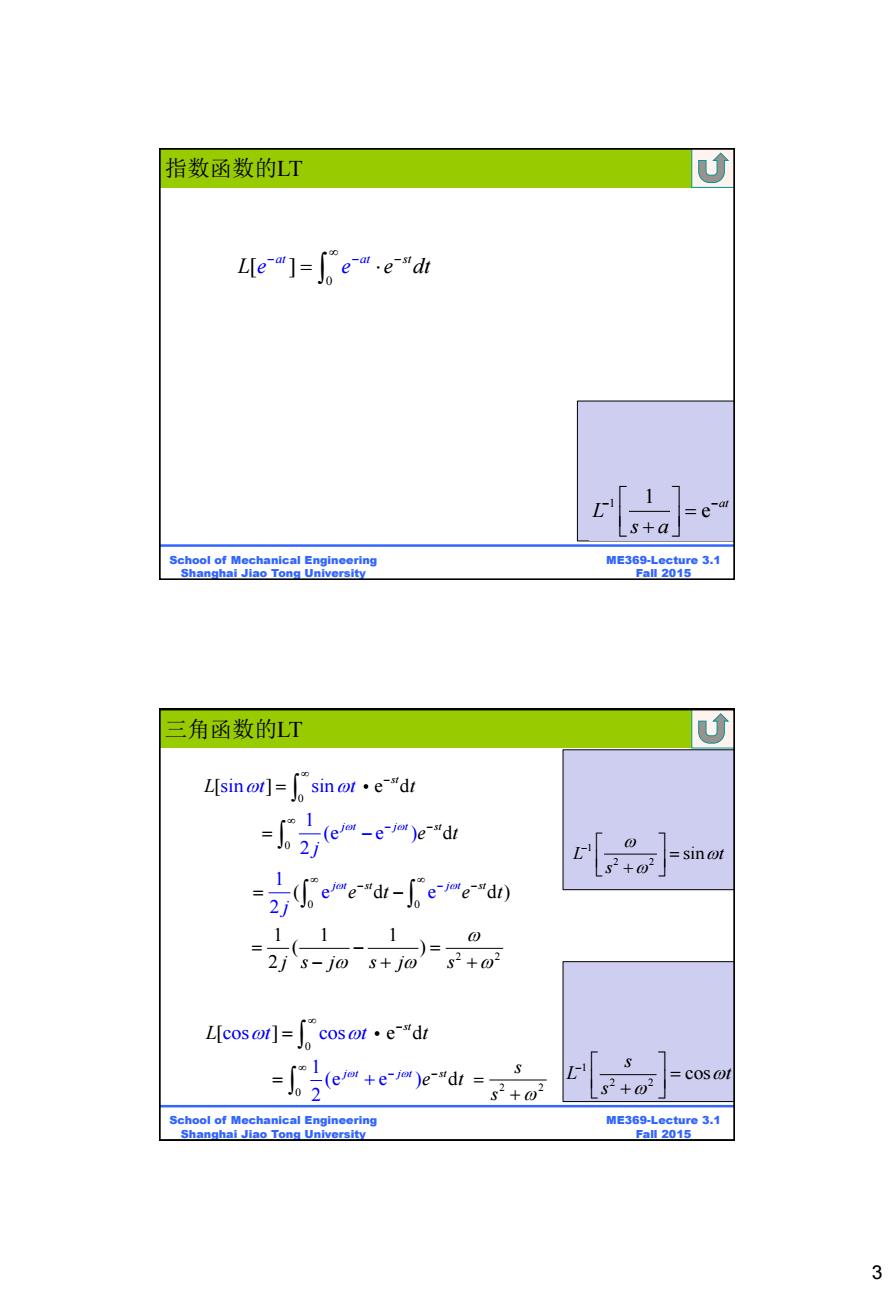
指数函数的LT Led 1=e s+a School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 三 角函数的LT 4 sin]=人'sinat·ed 。(e-e)e"d s+o sinot jega-egan 1,1-1)= 2j s-jo s+j@'s2+02 cos@t·edt (el)e-"dt coSot J02 s2+o2 s2+o2 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 3
3 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 1 1 e at L s a 0 [ ] at at st L e e e dt 指数函数的LT BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 三角函数的LT 0 [ ] sin sin d e st L t t t 0 1 (e e ) 2 d j t j t st t j e 0 [ ] cos cos d e st L t t t 2 2 1 1 1 ( ) 2 j s j s j s 0 1 (e e ) d 2 j t j t st e t 1 2 2 L t sin s 2 2 s s 0 0 1 ( e 2 d e d ) j t j t st st j e t e t 1 2 2 cos s L t s
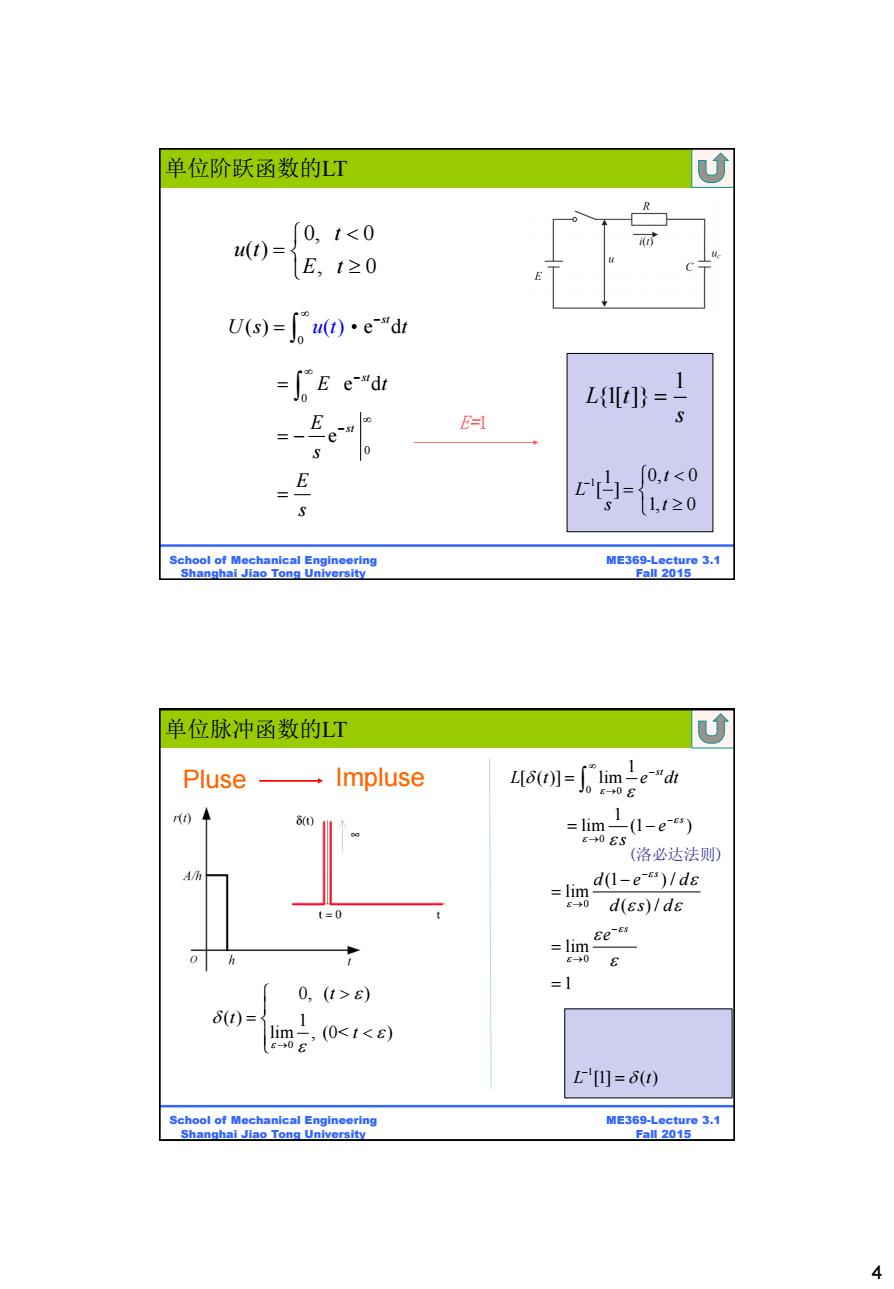
单位阶跃函数的LT 0,t<0 (t)= E,1≥0 Us)=0)e"d -edr L=1 =-Eg E=1 /0 E 0,t<0 1,t≥0 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 单位脉冲函数的LT Pluse一 Impluse 1 461=m后e”h nt) 8t) =lim L1-e") E→0ES (洛必达法则) A/h d(1-e-s)/ds =lim 6→0 t=0 d(ss)/da =lim 0 =1 0,(t>e) 6(t)= 1 画20<1<) L=) School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 4
4 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 单位阶跃函数的LT 0, 0 ( ) , 0 t u t E t 0 ( ) d ( ) e st U s t u t 0 0 e d e st st E t E s E s 1 L t {1[ ]} s 1 1 0, 0 [ ] 1, 0 t L s t E=1 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 单位脉冲函数的LT 0 0, ( ) ( ) 1 lim , (0< ) t t t 0 0 1 [ ( )] lim st L t e dt 0 1 lim (1 )s e s (洛必达法则) 0 0 (1 ) / lim ( ) / lim 1 s s d e d d s d e 1 L t [1] ( ) Pluse Impluse
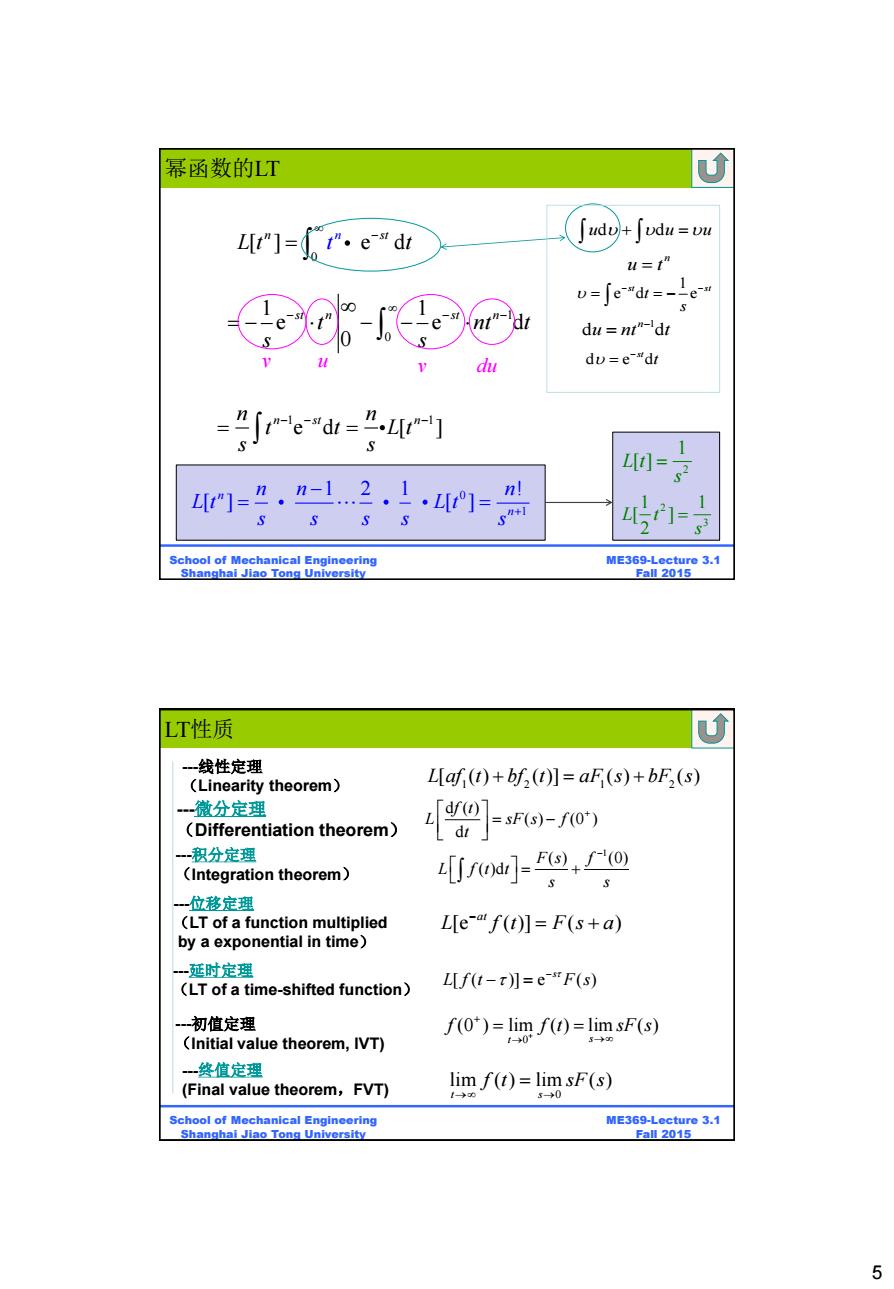
幂函数的LT Lt"]=t".e"dt udv+vdu=vu u=1" 1 v=∫e"d=--e" 0- du nt"-dt du du=e-"dt ∫red=”] 用= 1=”.-2.1.1= n School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fa12015 LT性质 一线性定理 (Linearity theorem) L[a时(t)+bf,(】=aF(s)+bF,(s) 徽分定理 (Differentiation theorem) =sF(s)-f0) 积分定理 (Integration theorem) froj-o -一位移定理 (LT of a function multiplied L[e-f(t)]=F(s+a) by a exponential in time) 一延时定理 (LT of a time-shifted function) LLf(t-t】=e-"F(s) 初值定理 f(0)=lim f(t)=lim sF(s) (Initial value theorem,IVT) 终值定理 (Final value theorem,FVT) lim f(t)=lim sF(s) → 3→0 School of Mechanical Engineering ME369-Lecture 3.1 Shanghai Jiao Tong University Fall 2015 5
5 BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 幂函数的LT 0 [ ] e d n st n L t t t 1 0 1 1 e e d 0 st n st n t nt t s s 1 1 e d [ ] n n n st n t t L t s s 0 1 1 2 1 ! [ ] [ ] n n n n n L t L t s s s s s u u u d d n u t d e dst t 1 d d n u nt t 1 e d e st st t s 2 1 L t[ ] s 2 3 1 1 [ ] 2 L t s v u v du BE315-Lecture 3.2 Fall 2011 ME369 Lecture 3.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University LT性质 ---微分定理 (Differentiation theorem) ---积分定理 (Integration theorem) ---位移定理 (LT of a function multiplied by a exponential in time) ---延时定理 (LT of a time-shifted function) ---终值定理 (Final value theorem,FVT) ---初值定理 (Initial value theorem, IVT) ---线性定理 (Linearity theorem) d ( ) ( ) (0 ) d f t L sF s f t 1 ( ) (0) ( )d F s f L f t t s s [ ( )] e ( ) s L f t F s [e ( )] ( ) at L f t F s a 0 lim ( ) lim ( ) t s f t sF s 0 (0 ) lim ( ) lim ( ) t s f f t sF s 1 2 1 2 L af t bf t aF s bF s [ ( ) ( )] ( ) ( )