同济大学：《空气动力学》课程电子教案（课件讲稿）Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow

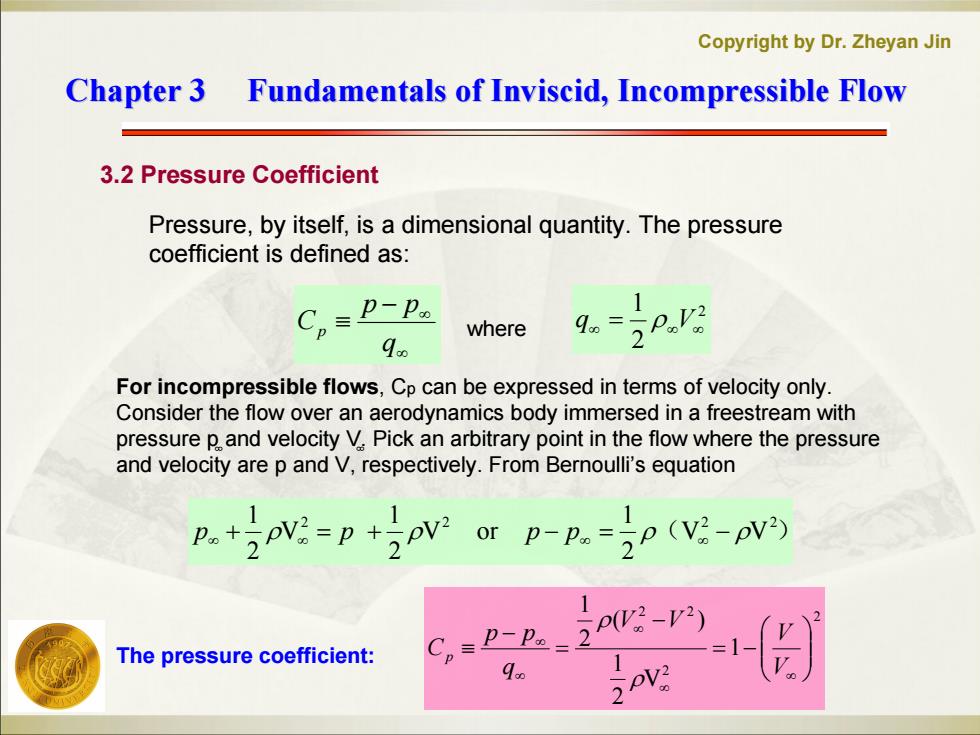
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.2 Pressure Coefficient Pressure,by itself,is a dimensional quantity.The pressure coefficient is defined as: C。=P-p2 1 where qoo For incompressible flows,Cp can be expressed in terms of velocity only Consider the flow over an aerodynamics body immersed in a freestream with pressure p and velocity VPick an arbitrary point in the flow where the pressure and velocity are p and V,respectively.From Bernoulli's equation P.+V:-P+V:OF P-P.-0 (V-PV) 2p-2 The pressure coefficient: 1

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.2 Pressure Coefficient For incompressible flows, Cp can be expressed in terms of velocity only. Consider the flow over an aerodynamics body immersed in a freestream with pressure p ͚and velocity V͚. Pick an arbitrary point in the flow where the pressure and velocity are p and V, respectively. From Bernoulli’s equation where 2 2 1    V q      q p p Cp 2 2 （ V 2 V 2 ） 2 1 V or 2 1 V 2 1 p      p   p  p       The pressure coefficient: Pressure, by itself, is a dimensional quantity. The pressure coefficient is defined as: 2 2 2 2 1 V 2 1 ( ) 2 1                    V V V V q p p Cp  

Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.3 Laplace's Equation Continuity equation: +v.pV=0 t For incompressible flow,p =constant ap=0、 e(pV)=pv.V at 7.V=0 币=V中 For irrotational flow: 7·(V)=0 7b=0 Laplace's equation.Solutions of Laplace's equation are called harmonic functions

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.3 Laplace’s Equation Laplace’s equation. Solutions of Laplace’s equation are called harmonic functions. For incompressible flow, ρ=constant V 0 0 V V t                  （ ） V 0 t          0 ( ) 0 2           V   For irrotational flow: Continuity equation:

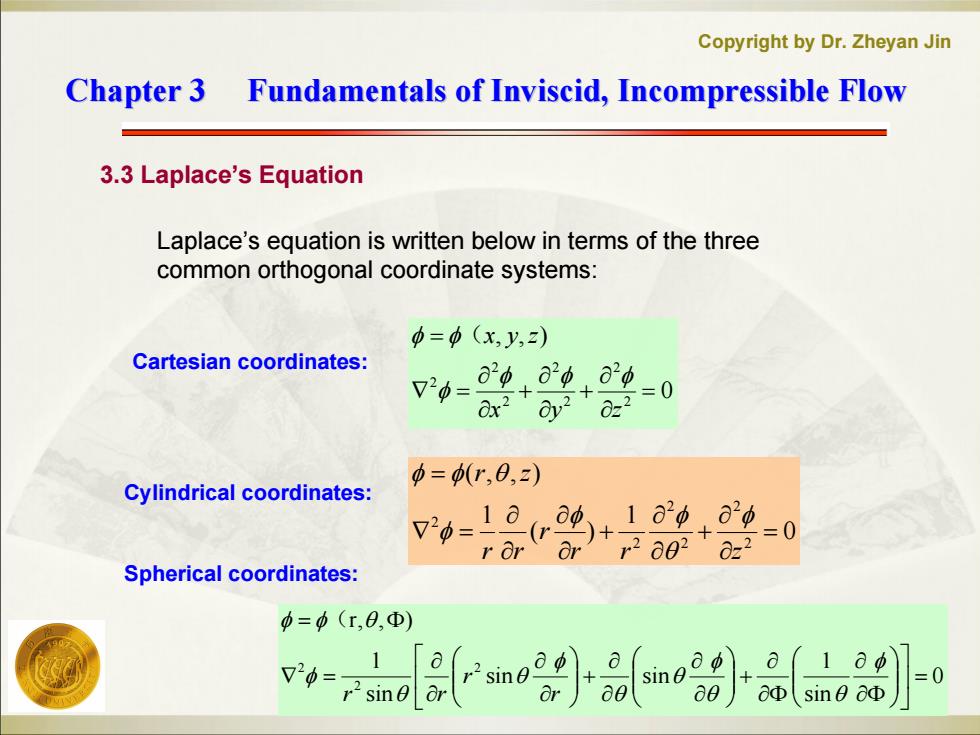
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.3 Laplace's Equation Laplace's equation is written below in terms of the three common orthogonal coordinate systems: 中=φ(x,y,2) Cartesian coordinates: v0=0+8,o' Oxoo =0 p=(t,0,z) Cylindrical coordinates: vb=18 o 、,1a2中，a2 -(r =0 r2a02 0z2 Spherical coordinates: =(r,0,Φ) 1 7= 2 a a 1 in sin rsine a0 Φ sin0oΦ

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.3 Laplace’s Equation 0 , , ) 2 2 2 2 2 2 2             x y z x y z       （ 0 1 ( ) 1 ( , , ) 2 2 2 2 2 2               r r z r r r r z         Cartesian coordinates: Laplace’s equation is written below in terms of the three common orthogonal coordinate systems: Cylindrical coordinates: Spherical coordinates: 0 sin 1 sin sin sin 1 r, , ) 2 2 2                                                               r r r r （

Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.3 Laplace's Equation 1.Any irrotational,incompressible flow has a velocity potential and stream function that both satisfy Laplace's equation. 2.Conversely,any solution of Laplace's equation represents the velocity potential or stream function(two-dimensional)for an irrotational,incompressible flow. Note that Laplace's equation is a second-order linear partial differential equation.The fact that it is linear is particularly important,because the sum of any particular solutions of a linear differential equation is also a solution of the equation: 0=4+42+…+9

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.3 Laplace’s Equation   1  2    n 1. Any irrotational, incompressible flow has a velocity potential and stream function that both satisfy Laplace’s equation. Note that Laplace’s equation is a second-order linear partial differential equation. The fact that it is linear is particularly important, because the sum of any particular solutions of a linear differential equation is also a solution of the equation: 2. Conversely, any solution of Laplace’s equation represents the velocity potential or stream function ( two-dimensional) for an irrotational, incompressible flow

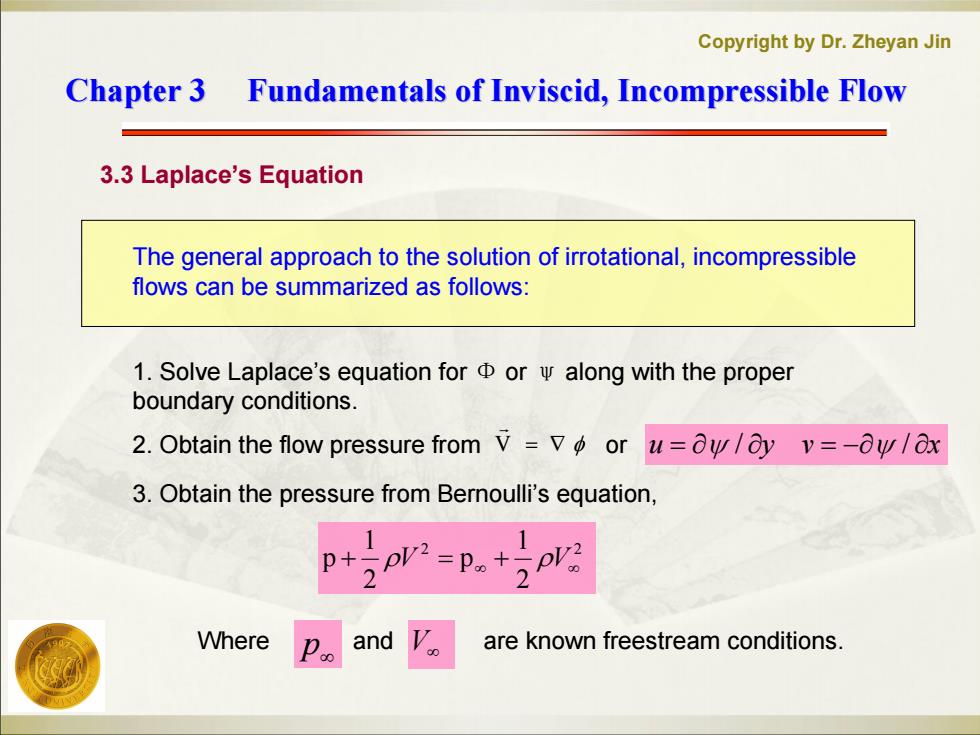
Copyright by Dr.Zheyan Jin Chapter 3 Fundamentals of Inviscid,Incompressible Flow 3.3 Laplace's Equation The general approach to the solution of irrotational,incompressible flows can be summarized as follows: 1.Solve Laplace's equation for or w along with the proper boundary conditions. 2.Obtain the flow pressure from V=or u=du/dy v=-dy/x 3.Obtain the pressure from Bernoulli's equation, 1 p+pV2=P.+pV2 2 Where P and are known freestream conditions

Copyright by Dr. Zheyan Jin Chapter 3 Fundamentals of Chapter 3 Fundamentals of Inviscid Inviscid, Incompressible Flow , Incompressible Flow 3.3 Laplace’s Equation 1. Solve Laplace’s equation for Φ or ψ along with the proper boundary conditions. 2. Obtain the flow pressure from The general approach to the solution of irrotational, incompressible flows can be summarized as follows: V    u   / y v    / x  2 2 2 1 p 2 1 p   V     V p  V or 3. Obtain the pressure from Bernoulli’s equation, Where and are known freestream conditions