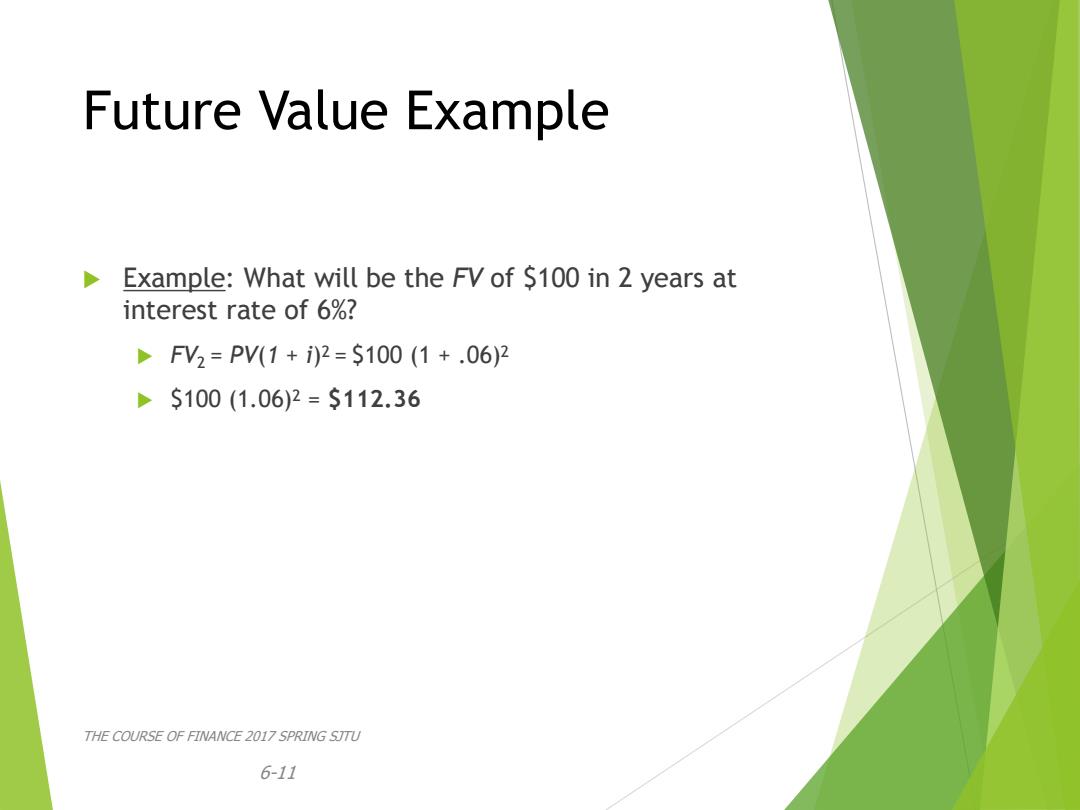
Future Value Example Example:What will be the FV of $100 in 2 years at interest rate of 6%? FV2=PV(1+i)2=$100(1+.06)2 $100(1.06)2=5112.36 THE COURSE OF FINANCE 2017 SPRING STTU 6-11
Future Value Example Example: What will be the FV of $100 in 2 years at interest rate of 6%? FV2 = PV(1 + i) 2 = $100 (1 + .06) 2 $100 (1.06) 2 = $112.36 THE COURSE OF FINANCE 2017 SPRING SJTU 6-11

How to Increase the Future Value? Future Value can be increased by: Increasing number of years of compounding (N) Increasing the interest or discount rate (r or i) Increasing the original investment (PV) See example on next slide THE COURSE OF FINANCE 2017 SPRING SJTU 6-12
How to Increase the Future Value? Future Value can be increased by: Increasing number of years of compounding (N) Increasing the interest or discount rate (r or i) Increasing the original investment (PV) See example on next slide THE COURSE OF FINANCE 2017 SPRING SJTU 6-12

Changing R(or i),N,and PV a.You deposit $500 in bank for 2 years.What is the FV at 2%?What is the FV if you change interest rate to 6%? FVat2%=500*(1.02)2=$520.2 FVat6%=500*(1.06)2=$561.8 b.Continue the same example but change time to 10 years.What is the FV now? FV=500*(1.06)10=$895.42 c.Continue the same example but change contribution to S1500.What is the FV now? DFV=1,500*(1.06)10=$2,686.27 THE COURSE OF FINANCE 2017 SPRING SJTU 6-13
Changing R(or i), N, and PV a. You deposit $500 in bank for 2 years. What is the FV at 2%? What is the FV if you change interest rate to 6%? FV at 2% = 500*(1.02) 2 = $520.2 FV at 6% = 500*(1.06) 2 = $561.8 b. Continue the same example but change time to 10 years. What is the FV now? FV = 500*(1.06) 10= $895.42 c. Continue the same example but change contribution to $1500. What is the FV now? FV = 1,500*(1.06) 10 = $2,686.27 THE COURSE OF FINANCE 2017 SPRING SJTU 6-13

RULE OF 72 This rule says that the number of years it takes for a sum of money to double in value ("the doubling time")is approximately equal to the number 72 divided by the interest rate expressed in percent per year 72 Doubling Time= Interest Rate THE COURSE OF FINANCE 2017 SPRING SJTU 14
RULE OF 72 This rule says that the number of years it takes for a sum of money to double in value (“the doubling time”) is approximately equal to the number 72 divided by the interest rate expressed in percent per year 72 Interest Rate THE COURSE OF FINANCE 2017 SPRING SJTU 14 Doubling Time=

Present Value of a Lump Sum FV=PV*(1+i)” Divide both sides by (1+i)"to obtain: PV= =*u+0” (1+i)” 15 THE COURSE OF FINANCE 2017 SPRING STTU
Present Value of a Lump Sum THE COURSE OF FINANCE 2017 SPRING SJTU 15 n n n n FV i i FV PV i FV PV i *(1 ) (1 ) Divide both sides by (1 ) to obtain : *(1 )